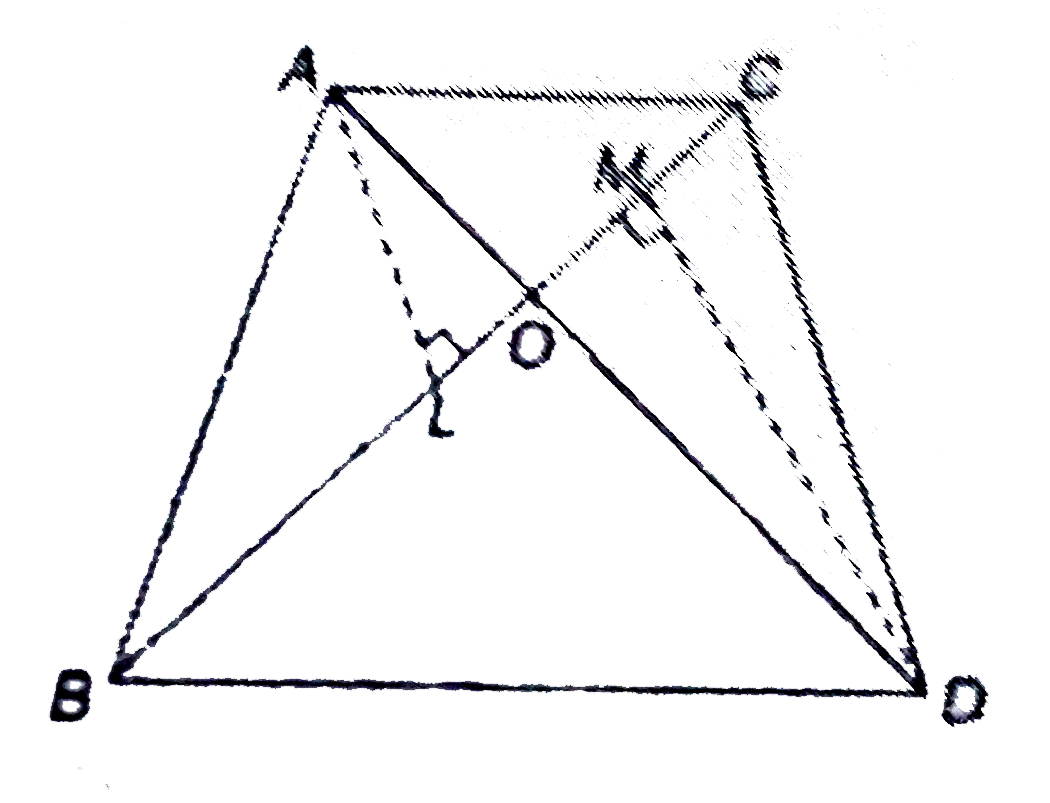
In figure ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)ar(DBC)=AODO.
Draw AL⊥BCandMC⊥BC
In △OLAand△OMD
∠ALO=∠=90∘
and ∠AOL=∠DOM ( vertically opposite angle)
∠OLA~∠OMD ( AAA similarity criterion)
ALDM=AODO …(1)
Now, ar(△ABC)ar(△DBC)=12×(BC)×(AL)12×(BC)×(DM)ALDM=AODO
Hence , (ar△ABCar(△DBC)=AODO Hence proved.
Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a
24 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Eercise 6b
1 Video
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Revision Exercise Long Questions
1 Video
- STATISTICS BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
4 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
In Figure, △ABC and △DBC are on the same base BC . If AD and BC intersect at O , prove that Area (ABC)Area (DBC)=AODO.
View SolutionThe diagonals of quadrilateral ABCD intersect at O . Prove that ar(ACB)ar(ACD)=BODO
View SolutionABC and DBC are two isosceles triangles on the same base BC (see Fig. 7.33). Show that∠ABD =∠ACD

View SolutionIn Figure, ABC and ABD are two triangles on the base AB. If line segment CD is bisected by AB at O, show that ar (△ABC)=ar ( △ABD)
View SolutionTriangles ABC and DBC are on the same base BC with A, D on opposite side of line BC, such that ar(△ABC)=ar(△DBC). Show that BC bisects AD.
View SolutionTriangles ABC and DBC are on the same base BC with A, D on opposite side of line BC, such that ar(⊥ABC)=ar(DBC). Show that BC bisects AD.
View SolutionTriangles ABC and DBC are two isosceles triangles on the same base BC and their vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
AP bisects angle BAC and BDC.
View SolutionTriangles ABC and DBC are two isosceles triangles on the same base BC and their vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
ΔABP≅ΔACP
View SolutionABC and DBC are two triangle on the same base BC such that A and D lie on the opposite sides of BC,AB=AC and DB =DC ,Show that AD is the perpedicular bisector of BC.
View SolutionΔABCand ΔDBCare two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC

View Solution
