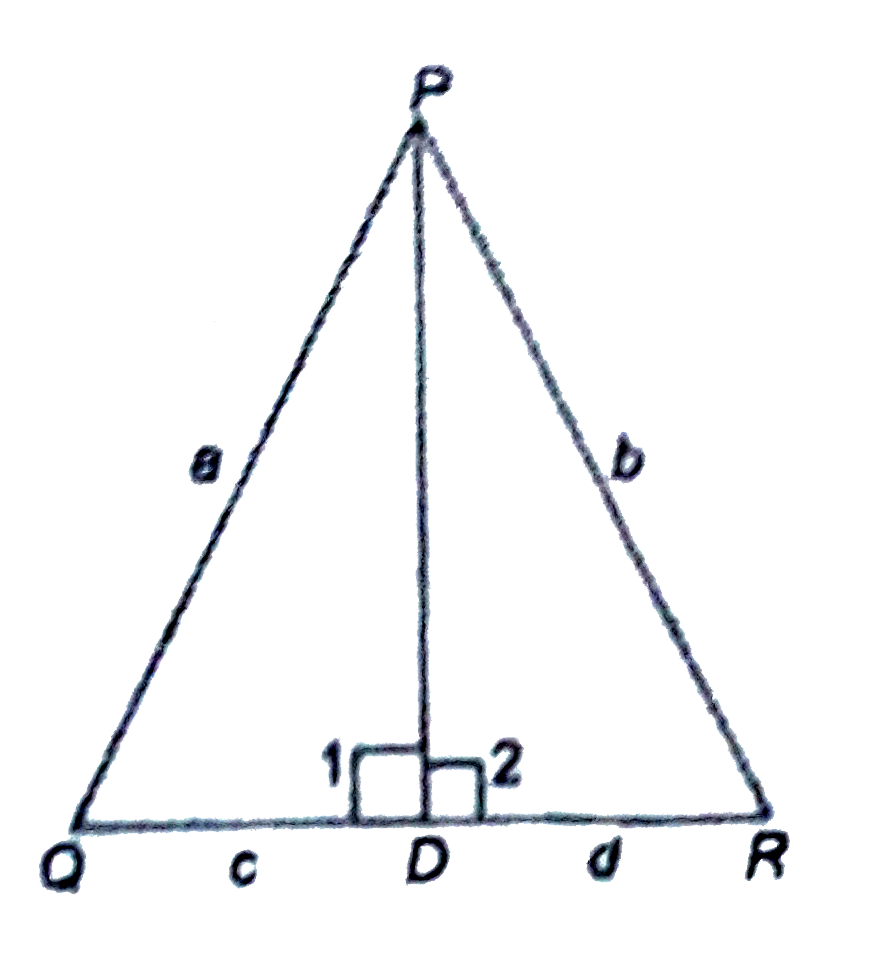
Ii , PDQR such that D lies on QR, if PQ=a,PR=b,QD=c and DR=d, then prove that (a+b)(a-b)=(c+d)(c-d).
Video Solution
Text Solution
Given: In
PQ=a,PR=b,QD=c and DR=d
To prove: (a+b) (a-b) = (c+d) (c-d)
Proof : in right angle
(by pythagoras theorem)
...(1)
similarlym in right angled
( by pythagoras theorem) (by pythagoras theorem )
From (1) and (2) ,we have
(a-b) (a+b) = (c-d) (c+d) Hence proved.
|
Topper's Solved these Questions
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a
24 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Eercise 6b
1 Videos
- TRIANGLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Revision Exercise Long Questions
1 Videos
- STATISTICS BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
4 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions
5 Videos
Similar Questions
If a ,b,c , d are in G.P. prove that
(b + c)(b + d) = (c + a)(c+d)
View SolutionIf a,b,c,d are in G.P. prove that: ab−cdb2−c2=a+cb
View SolutionIn ABC , if BD⊥AC and BC2=2 ACCD , then prove that AB=AC .
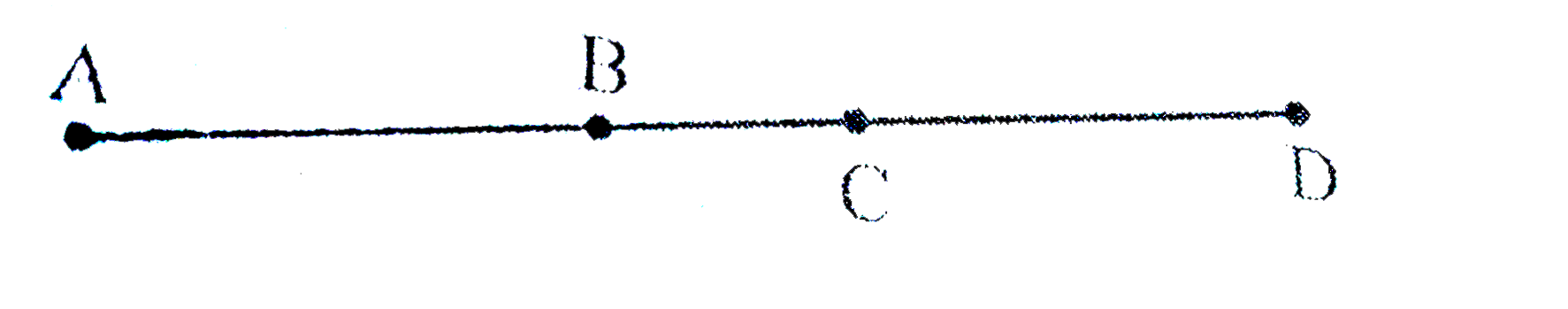
View SolutionIn Fig. 5.10, if AC=BD, then prove that AB=CD.
View SolutionIf a ,b,c , d are in G.P. prove that
(a−d)2=(b−c)2+(c−a)2+(d−b)2
View SolutionIn ABC , ray AD bisects ∠A and intersects BC in D . If BC=a , AC=b and AB=c , prove that BD=acb+c (ii) D C = abb+c
View SolutionIf a, b, c and d are four coplanr points, then prove that [abc]=[bcd]+[abd]+[cad].
View SolutionIn figure ∠ACB=90∘and CD⊥AB. Prove that BC2AC2=BDAD.
View SolutionIn Figure, ∠ACB=90. and CD⊥AB . Prove that CB2CA2=BDAD
View SolutionIn Figure, it is given that AB=CD and AD=BC. Prove that ADC≅CBA
View Solution
