A wheel of mass M and radius a and M.I. IG (about centre of mass) is set rolling with angular velocity ω up a rough inclined plane of inclination theta. The distance traveled by it up the plane is
IGω22mgsinθ
ω2(Ma2+IG)2Mgsinθ
IGω22ωMgsinθ
IGω2Mgsinθ
The correct Answer is:2
To solve the problem of a wheel rolling up a rough inclined plane, we will follow these steps:
Step 1: Understand the Problem
We have a wheel of mass M, radius a, and moment of inertia IG about its center of mass. The wheel is rolling up an inclined plane with an angle θ and an initial angular velocity ω. We need to find the distance S0 that the wheel travels up the incline before coming to a stop.
Step 2: Set Up the Coordinate System
Draw the inclined plane and identify the angle θ. The height h that the wheel rises can be expressed in terms of the distance S0 traveled along the incline:
h=S0sinθ
Step 3: Analyze the Forces Acting on the Wheel
The forces acting on the wheel are:
- The gravitational force Mg acting downwards.
- The normal force N perpendicular to the incline.
- The frictional force f acting up the incline.
Since the wheel is rolling without slipping, the point of contact with the incline is momentarily at rest.
Step 4: Apply Work-Energy Principle
The work done by gravity as the wheel moves up the incline is equal to the change in kinetic energy (both translational and rotational). The work done by gravity when the wheel rises a height h is:
W=−Mgh=−Mg(S0sinθ)
The initial kinetic energy of the wheel consists of both translational and rotational components:
KEinitial=12Mv2+12IGω2
Since the wheel is rolling without slipping, the relationship between linear velocity v and angular velocity ω is:
v=ωa
Substituting this into the kinetic energy expression gives:
KEinitial=12M(ωa)2+12IGω2=12ω2(Ma2+IG)
Step 5: Set Up the Energy Equation
Setting the work done by gravity equal to the change in kinetic energy, we have:
−Mg(S0sinθ)=12ω2(Ma2+IG)
Step 6: Solve for S0
Rearranging the equation to solve for S0:
S0sinθ=−12ω2(Ma2+IG)Mg
S0=−12ω2(Ma2+IG)Mgsinθ
Since the distance traveled cannot be negative, we take the absolute value:
S0=12ω2(Ma2+IG)Mgsinθ
Final Answer
The distance traveled by the wheel up the incline is:
S0=ω2(Ma2+IG)2Mgsinθ
We have a wheel of mass M, radius a, and moment of inertia IG about its center of mass. The wheel is rolling up an inclined plane with an angle θ and an initial angular velocity ω. We need to find the distance S0 that the wheel travels up the incline before coming to a stop.
Draw the inclined plane and identify the angle θ. The height h that the wheel rises can be expressed in terms of the distance S0 traveled along the incline:
h=S0sinθ
The forces acting on the wheel are:
- The gravitational force Mg acting downwards.
- The normal force N perpendicular to the incline.
- The frictional force f acting up the incline.
The work done by gravity as the wheel moves up the incline is equal to the change in kinetic energy (both translational and rotational). The work done by gravity when the wheel rises a height h is:
W=−Mgh=−Mg(S0sinθ)
KEinitial=12Mv2+12IGω2
Since the wheel is rolling without slipping, the relationship between linear velocity v and angular velocity ω is:
v=ωa
Substituting this into the kinetic energy expression gives:
KEinitial=12M(ωa)2+12IGω2=12ω2(Ma2+IG)
Setting the work done by gravity equal to the change in kinetic energy, we have:
−Mg(S0sinθ)=12ω2(Ma2+IG)
Rearranging the equation to solve for S0:
S0sinθ=−12ω2(Ma2+IG)Mg
S0=−12ω2(Ma2+IG)Mgsinθ
S0=12ω2(Ma2+IG)Mgsinθ
The distance traveled by the wheel up the incline is:
S0=ω2(Ma2+IG)2Mgsinθ
Topper's Solved these Questions
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE-1
4 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE-2
5 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE
56 Videos
Similar Questions
A solid cylinder is rolling down a rough inclined plane of inclination θ. Then
View SolutionA uniform solid sphere of radius R, rolling without sliding on a horizontal surface with an angular velocity ω0, meets a rough inclined plane of inclination θ=60∘. The sphere starts pure rolling up the plane with an angular velocity ω Find the value of ω.
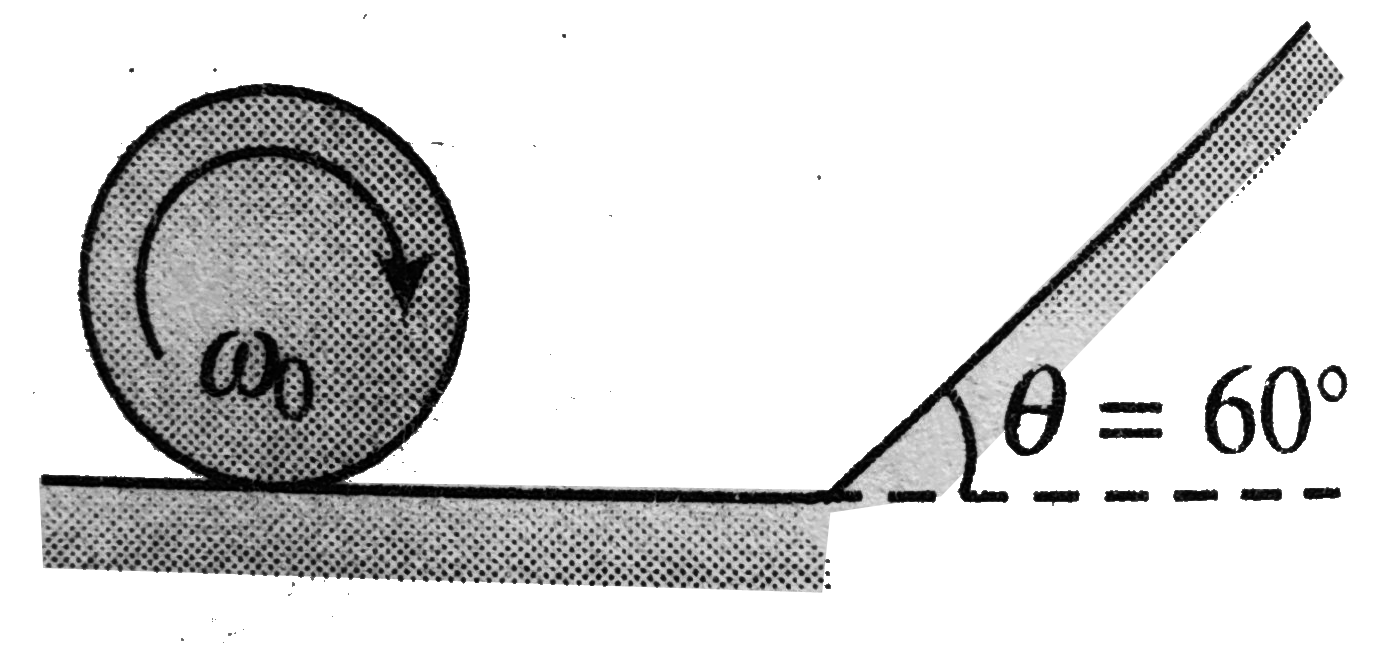
View Solution
Knowledge Check
A solid cylinder rolls up an inclined plane of inclination θ with an initial velocity v. How far does the cylinder go up the plane ?
A3v22gsinθBv24gsinθC3v2gsinθD3v24gsinθ
A solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionA solid sphere is given an angular velocity ω and kept on a rough fixed incline plane. The choose the correct statement.
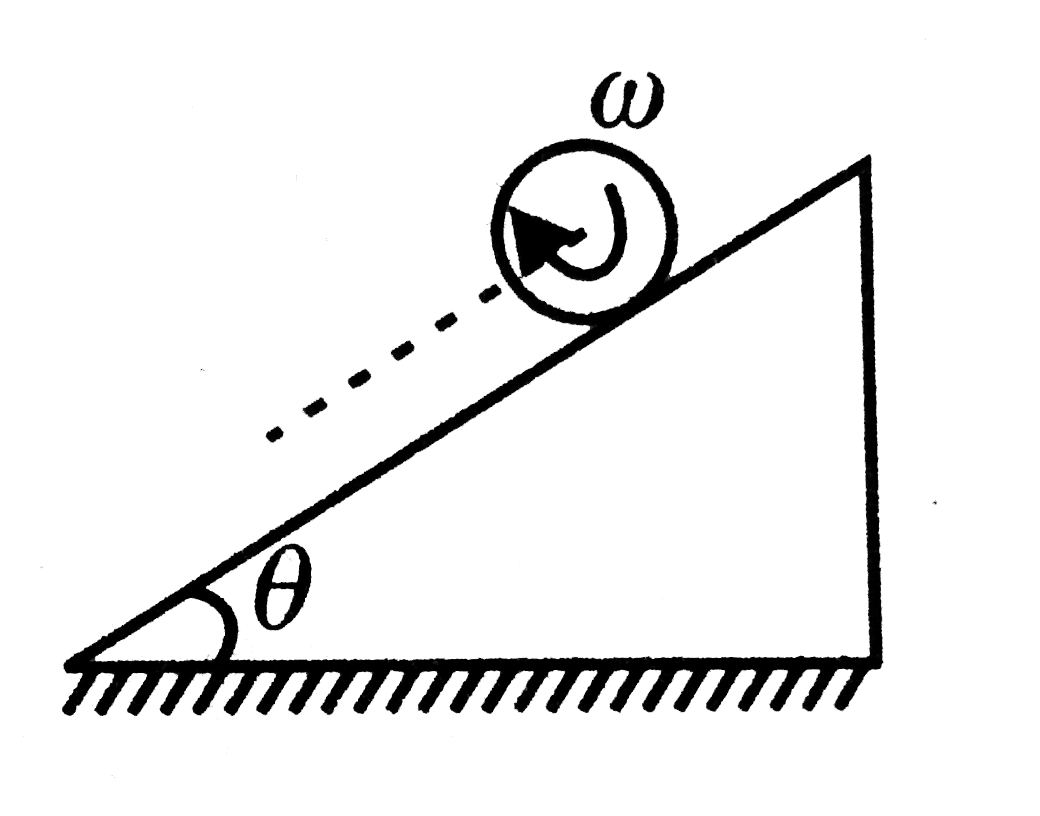
View SolutionA hollow sphere of mass m and radius R is rolling downdard on a rough inclined plane of inclination θ. If the coefficient of friction between the hollow sphere and incline is μ, then
View SolutionA disc is rotated about its axis with a certain angular velocity and lowered gently on a rough inclined plane as shown in Fig., then

View SolutionA disc is rotated about its axis with a certain angular velocity and lowered gently on a rough inclined plane as shown in Fig., then

View SolutionA block of mass m is thrown upwards with some initial velocity up a rough inclined plane as shown

Match the following Column-I with Column-II

View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View Solution
