A fully charged capacitor has a capacitance 'C'. It is discharged through a small coil of resistance wire embedded in a thermally insulated block of specific heat capacity 's' and mass 'm'. If the temperature of the block is raised by 'DeltaT', the potential difference 'V' across the capacitance is
√2mCΔTS
mCΔTS
msΔTC
√2mSΔTC
The correct Answer is:D
To solve the problem step by step, we will use the concepts of energy stored in a capacitor and the heat energy transferred to the block.
Step 1: Understand the energy stored in a capacitor
The energy (U) stored in a capacitor is given by the formula:
U=12CV2
where C is the capacitance and V is the potential difference across the capacitor.
Hint: Remember that the energy stored in a capacitor is directly related to its capacitance and the square of the voltage across it.
Step 2: Understand the heat energy transferred to the block
When the capacitor discharges, the energy is used to raise the temperature of the block. The heat energy (H) required to raise the temperature of a mass m by ΔT with a specific heat capacity s is given by:
H=msΔT
Hint: Heat energy is calculated using mass, specific heat capacity, and the change in temperature.
Step 3: Apply the law of conservation of energy
According to the law of conservation of energy, the energy stored in the capacitor will be equal to the heat energy transferred to the block:
12CV2=msΔT
Hint: The energy lost by the capacitor is equal to the energy gained by the block.
Step 4: Rearrange the equation to solve for V2
From the equation above, we can rearrange it to find V2:
CV2=2msΔT
V2=2msΔTC
Hint: Isolate V2 to find a relationship between voltage, mass, specific heat capacity, temperature change, and capacitance.
Step 5: Solve for V
Now, take the square root of both sides to find V:
V=√2msΔTC
Hint: Remember that taking the square root will give you the potential difference across the capacitor.
Final Answer
Thus, the potential difference V across the capacitor is:
V=√2msΔTC
The energy (U) stored in a capacitor is given by the formula:
U=12CV2
where C is the capacitance and V is the potential difference across the capacitor.
When the capacitor discharges, the energy is used to raise the temperature of the block. The heat energy (H) required to raise the temperature of a mass m by ΔT with a specific heat capacity s is given by:
H=msΔT
According to the law of conservation of energy, the energy stored in the capacitor will be equal to the heat energy transferred to the block:
12CV2=msΔT
From the equation above, we can rearrange it to find V2:
CV2=2msΔT
V2=2msΔTC
Now, take the square root of both sides to find V:
V=√2msΔTC
Thus, the potential difference V across the capacitor is:
V=√2msΔTC
Topper's Solved these Questions
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 2
1 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 3
1 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 2
40 Videos
- BASIC MATHEMATICS & VECTORSBOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable
50 Videos
- CURRENT ELECTRICITYBOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F
10 Videos
Similar Questions
A capacitor of capacitance C is fully charged by a 200 V battery. It is then discharged through a small coil of resistance wire embedded in a thermally insulated block of specific heat 2.5×102Jkg−1K−1 and of mass 0.1 kg. if the temperature of the block rises by 0.4 K, what is the value of C?
View SolutionA capacitor of capacitance C has charge Q. it is connected to an identical capacitor through a resistance. The heat produced in the resistance is
View SolutionA parallel plate capacitor of capacitance 1μF has a charge of +2μC on one of the plates and a charge of +4μC on the other. The potential difference developed across the capacitor is
View SolutionTwo parallel plate capacitors of capacitances C and 2C are connected in parallel and charged to a potential difference V. The battery is then disconnected and the region between the plates of the capacitor C is completely filled with a material of dielectric constant K. The potential differences across the capacitors now becomes...........
View SolutionTwo capacitors of capacitance 2μF and 3μF respectively are charged to potential difference 20 V and 40 V respectively. Now the capacitors are connected in series with a resistance such that the positively charged plate of one capacitor is connected to the positively charged plate of the other. The initial current through the resistance is I0. The potential difference across the 2μF capacitor at the instant the current has reduced to I02 is_______ V.
View SolutionA 5.0(μ)F capacitor having a charge of (20(μ)C)is discharged through a wire aof resistance (5.0Ω). Find the heat dissipated in the wire between 25 to 50 (mu)s after the connections are made.
View SolutionA capacitor of capacitance C is charged to a potential difference V0. The charged battery is disconnected and the capacitor is connected to a capacitor of unknown capacitance Cx. The potential difference across the combination is V. The value of Cx should be
View SolutionA capacitor is connected to a 12 V battery through a resistance of (10Ω).It is found that the potential difference across the capacitor rises to 4.0 V in 1(mu)s. find the capacitance of the capacitor.
View SolutionTwo capacitors of capacitance C and 3C are charged to potential difference V0 and 2V0, respectively, and connected to an inductor of inductance L as shows in Fig. Initially, the current in the inductor is zero. Now, switch S is closed.
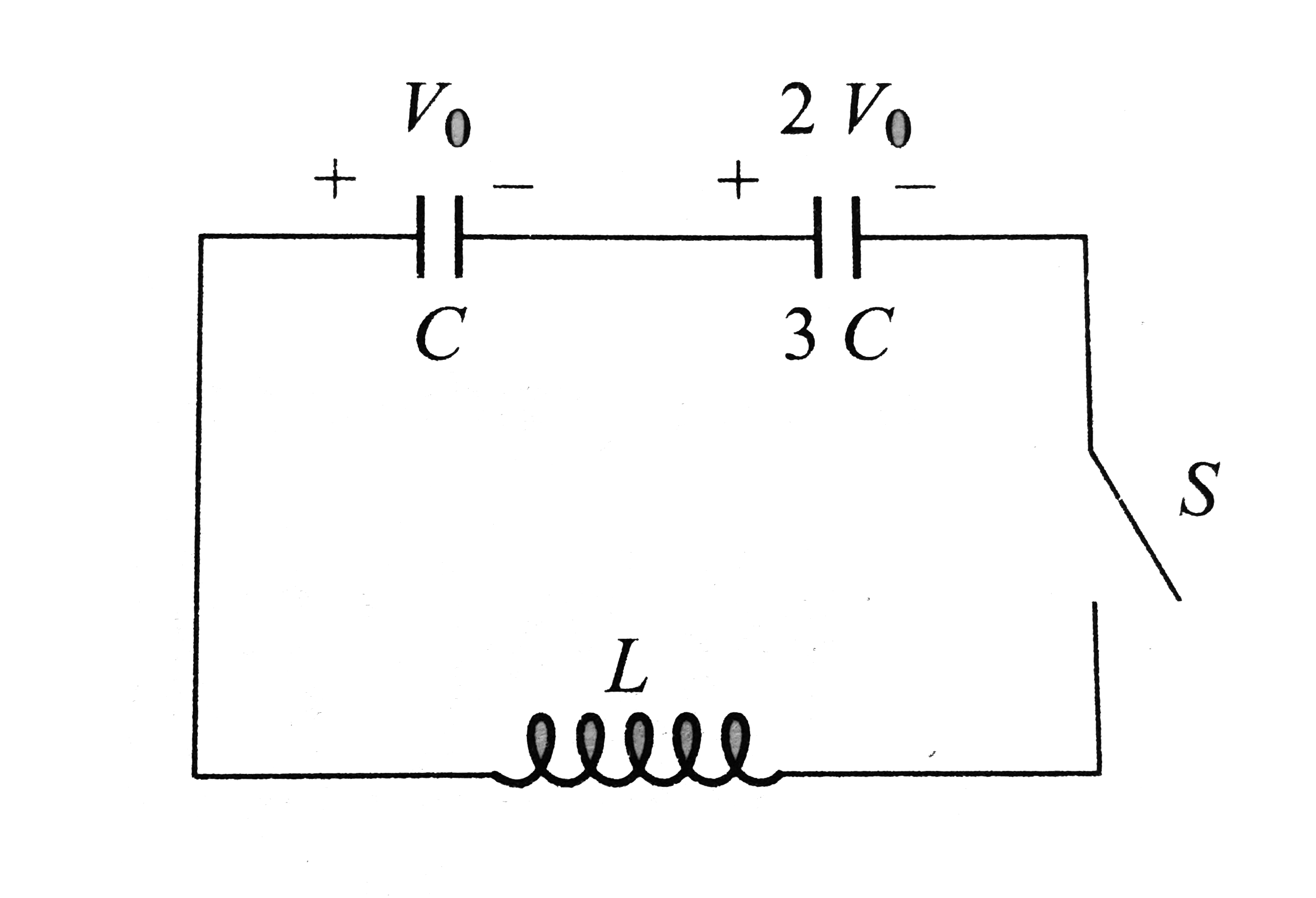
Potential difference across capacitor of capacitance C when the current in the circuit is maximum is
View SolutionTwo capacitors of capacitance C and 3C are charged to potential difference V0 and 2V0, respectively, and connected to an inductor of inductance L as shows in Fig. Initially, the current in the inductor is zero. Now, switch S is closed.
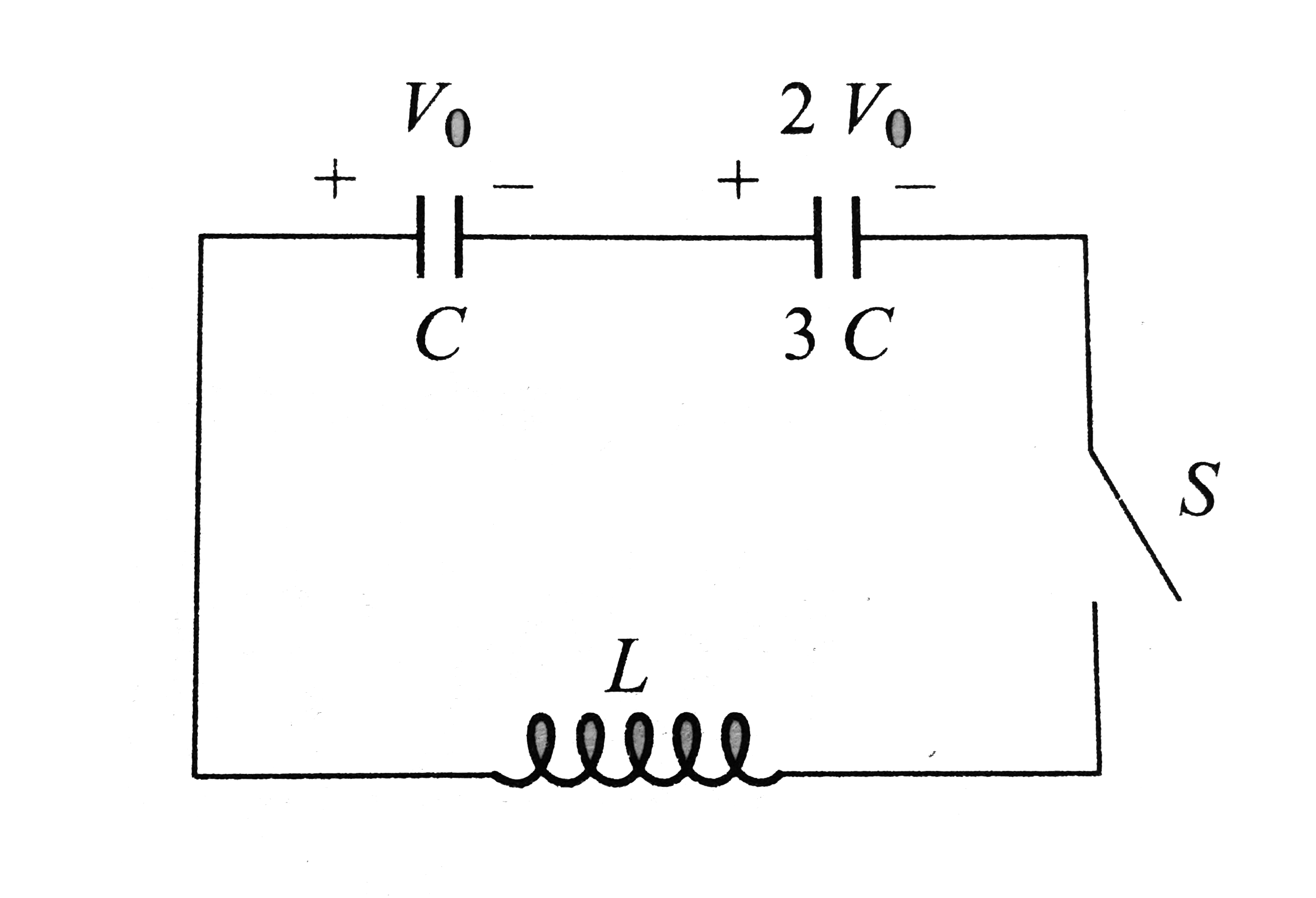
Potential difference across capacitor of capacitance 3C when the current in the circuit is maximum is
View Solution
