Consider regular polygons with number of sides n = 3, 4, 5 ...... as shown in the figure, The center of mass of all the polygons is at height h from the ground. They roll on a horizontal surface about the leading vertex without slipping and sliding as depicted,. The maximum increase in height of the locus of the center of mass for each polygton is Δ. Then Δ depends on n and h as
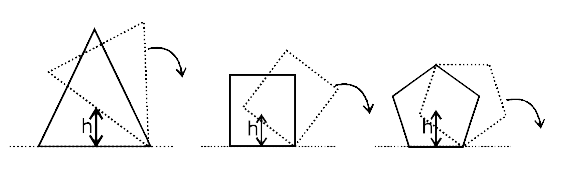
Δ=hsin2(πn)
Δ=hsin2(2πn)
Δ=hsin2(π2n)
Δ=h⎛⎜ ⎜⎝1cos(πh)−1⎞⎟ ⎟⎠
The correct Answer is:D
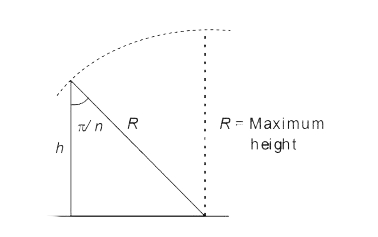
cos(πn)=hR
Δ=R−h=hcos(πh)−h=h[1cos(πn)−1]
Topper's Solved these Questions
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - PARAGRAPH QUESTIONS
Explore 5 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - ASSERTION & REASON TYPE
Explore 1 Video
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE MAIN (ARCHIVE)
Explore 53 Videos
- ELECTROSTATICSBook:VMC MODULES ENGLISHChapter:ELECTROSTATICSExercise:JEE Advanced (Archive)
Explore 89 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE ADVANCED (ARCHIVE )
Explore 111 Videos
Similar Questions
There are two regular polygons, the numbers of sides in one being the double the number in the other, and an angle of one polygon is to an angle of the other is 9 to 8, find the number of sides of each polygon.
View SolutionA sphere of mass m and radius r rolls on a horizontal plane without slipping with a speed u. Now it rolls up vertically, then maximum height it would be attain will be
View Solution
Knowledge Check
In the figure, O is the center of the circle. What of the following must be true about the perimeter of the triangle shown?
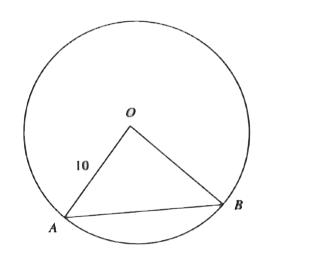 ALess than 10BGreater than 20CLess than 40DLess than 40 and greater than 20
ALess than 10BGreater than 20CLess than 40DLess than 40 and greater than 20The sum of interior angles of a regular polygon is twice the sum of its exterior angles. Find the number of sides of the polygon.
A8B7C5D9
The interior angle of a regular polygon is 1560. Find the number of sides of the polygon.
View SolutionA uniform disc of mass m and radius r rolls without slipping along a horizontal surface and ramp, as shown above. The disk has an initial velocity of υ . What is the maximum height h to which the center of mass of the disc rises ?

View SolutionEach side of the equilateral triangle shown is 2. What is the height h of the triangle?
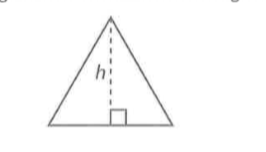
View SolutionThree identical metal balls each of radius r are placed touching each other on a horizontal surface such that an equilateral triangle is formed, when the center of three balls are joined. The center of mass of system is located at the
View SolutionThe permieter of a polygon is 24.5cm. Each side of the polygon is 3.5 cm1 in length. Find the number of sides of the polygon.
View SolutionThe interior angles of a regular polygon measure 150∘ each. The number of diagonals of the polygon is
View Solution