A small particle of mass m moving inside a heavy, hollow and straight tube along the tube axis undergoes elastic collision at two ends. The tube has no friction and it is closed at one end by a flat surface while the other end is fitted with a heavy movable flat piston as shown in figure. When the distance of the piston from closed end is L=L0 the particle speed is v=v0 The piston is moved inward at a very low speed V such that V<<dLLv0, where dL is the infinitesimal displacement of the piston. Which of the following statements(s) is(are) correct?
After each collision with the piston, the particle speed increases by 2V
If the piston moves inward by dL, the particle speed increases by 2vdLL
The rate at which the particle strikes the piston is v/L
The particle’s kinetic energy increases by a factor of 4 when the piston is moved inward from L0to12L0
The correct Answer is:A, D
To solve the problem, we need to analyze the motion of the particle and the effects of the moving piston on the particle's speed and kinetic energy. Here’s the step-by-step solution:
Step 1: Understand the System
We have a small particle of mass m moving inside a hollow tube. The tube has one end closed and the other end has a movable piston. The particle undergoes elastic collisions with both ends of the tube. Initially, when the distance of the piston from the closed end is L=L0, the particle's speed is v=v0.
Step 2: Analyze the Movement of the Piston
The piston moves inward at a very low speed V such that V≪dLLv0. This means the piston is moving slowly compared to the speed of the particle and the changes in length L.
Step 3: Calculate the Time for Collisions
The time taken for the particle to collide with the piston can be calculated as follows:
- The total length of the tube is 2L (from one end to the other).
- The time T for one complete round trip (collision with both ends) is given by:
T=2Lv0
Step 4: Determine the Number of Collisions
The number of collisions N in a time interval can be calculated by:
- The number of collisions in one second is:
N=v02L
- In a small time interval dt, the number of collisions is:
dN=v02Ldt
Step 5: Effect of Piston Movement on Particle Speed
As the piston moves inward by an infinitesimal distance dL, the speed of the particle after each collision changes. The change in speed dv after N collisions can be calculated as:
- The increment in velocity after N collisions:
Δv=2v0⋅dN=2v0⋅v02Ldt=v20Ldt
Step 6: Integrate to Find Final Speed
Integrating the change in speed gives us the final speed vf:
vf=v0+∫v20Ldt
As the piston moves inward, the effective length L decreases, leading to an increase in speed.
Step 7: Kinetic Energy Calculation
The kinetic energy K of the particle is given by:
K=12mv2
Initially, the kinetic energy is:
K0=12mv20
After the piston moves and the speed increases to vf, the new kinetic energy becomes:
Kf=12m(2v0)2=2mv20
Thus, the kinetic energy increases by a factor of 4.
Conclusion
After analyzing the system, we can conclude:
1. After each collision with the piston, the particle's speed increases.
2. The particle's kinetic energy increases by a factor of 4 when the piston moves inward.
We have a small particle of mass m moving inside a hollow tube. The tube has one end closed and the other end has a movable piston. The particle undergoes elastic collisions with both ends of the tube. Initially, when the distance of the piston from the closed end is L=L0, the particle's speed is v=v0.
The piston moves inward at a very low speed V such that V≪dLLv0. This means the piston is moving slowly compared to the speed of the particle and the changes in length L.
The time taken for the particle to collide with the piston can be calculated as follows:
- The total length of the tube is 2L (from one end to the other).
- The time T for one complete round trip (collision with both ends) is given by:
T=2Lv0
The number of collisions N in a time interval can be calculated by:
- The number of collisions in one second is:
N=v02L
- In a small time interval dt, the number of collisions is:
dN=v02Ldt
As the piston moves inward by an infinitesimal distance dL, the speed of the particle after each collision changes. The change in speed dv after N collisions can be calculated as:
- The increment in velocity after N collisions:
Δv=2v0⋅dN=2v0⋅v02Ldt=v20Ldt
Integrating the change in speed gives us the final speed vf:
vf=v0+∫v20Ldt
As the piston moves inward, the effective length L decreases, leading to an increase in speed.
The kinetic energy K of the particle is given by:
K=12mv2
Initially, the kinetic energy is:
K0=12mv20
After the piston moves and the speed increases to vf, the new kinetic energy becomes:
Kf=12m(2v0)2=2mv20
Thus, the kinetic energy increases by a factor of 4.
After analyzing the system, we can conclude:
1. After each collision with the piston, the particle's speed increases.
2. The particle's kinetic energy increases by a factor of 4 when the piston moves inward.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - PARAGRAPH QUESTIONS5videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - ASSERTION & REASON TYPE1videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE MAIN (ARCHIVE)53videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)89videos
ELECTROSTATICS
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )111videos
GASEOUS STATE & THERMODYNAMICS
Similar Questions
A string of lenth l fixed at one end carries a mass m at the other end. The strings makes 2πrevs−1 around the axis through the fixed end as shown in the figure, the tension in the string is
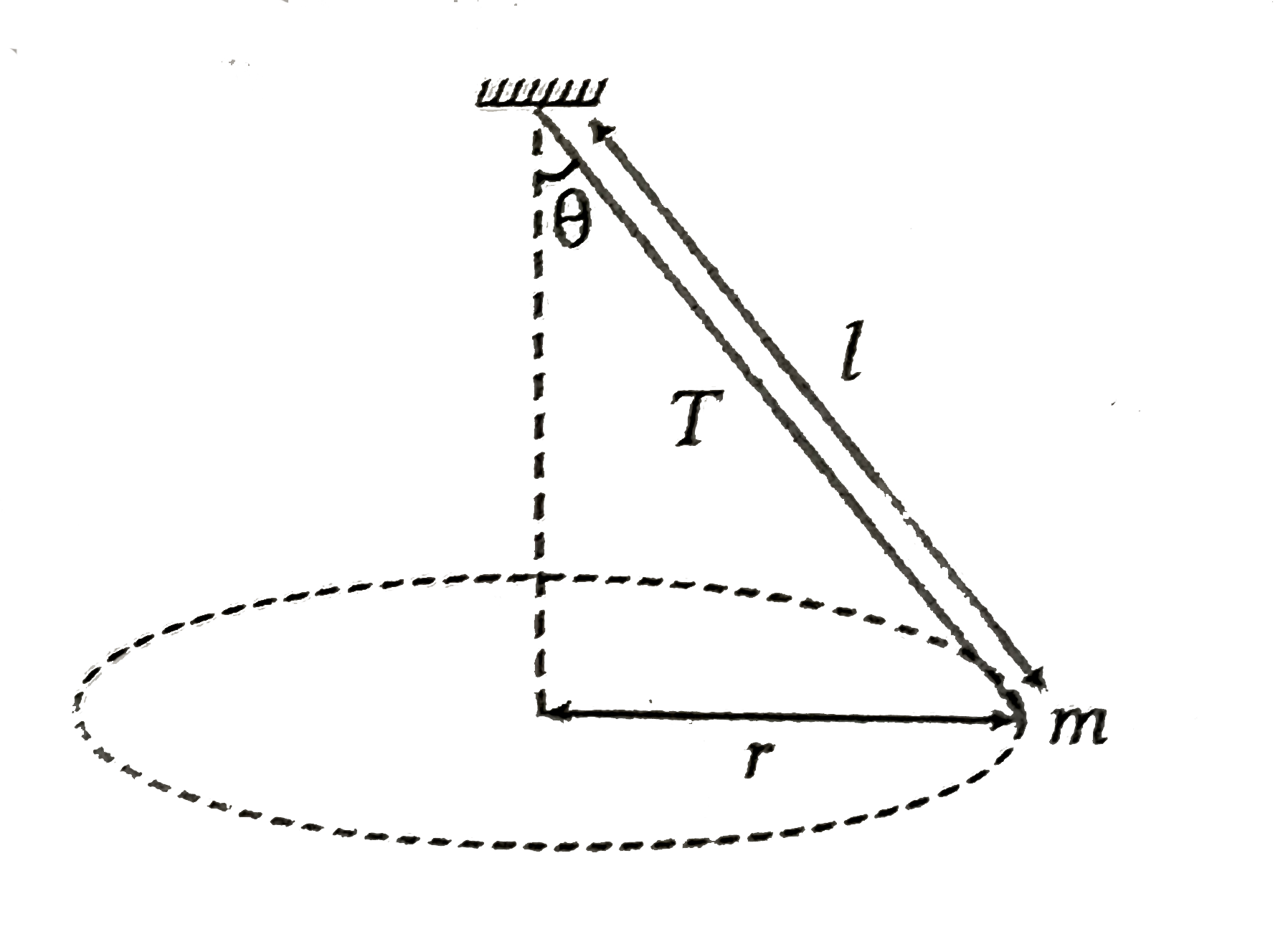 View Solution
View SolutionIn a resonace tube experiment, the first resonance is obtained when the level of the water in the tube is at 20 cm from the open end. Resonace will also be obtained when the distance of the water level from the open end is
View SolutionIt is desired to increase the fundamental resonance frequency in a tube which is closed at one end. This can be achieved by
View SolutionIt is desired to increse the fundamental resonance frequency in a tube which is closed at one end. This can be achieved by
View SolutionExplain the
Why is one end of drawing board pin broad and flat, whereas its other end is pointed and sharp?View SolutionAn organ pipe of length L is open at one end and closed at other end. The wavelengths of the three lowest resonating frequencies that can be produced by this pipe are
View SolutionA man of mass M stands at one end of a plank of length L Which lies at rest on a frictionless surface . The man walks to the other end of the plank. If the mass of the plank is 3M, the distance that the man moves relative to the ground is
View SolutionA man of mass M stands at one end of a stationary plank of length L, lying on a smooth surface. The man walks to the other end of the plank. If the mass of the plank is M/3, the distance that the man moves relative to the ground is
View SolutionA man of mass M stands at one end of a stationary plank of length L, lying on a smooth surface. The man walks to the other end of the plank. If the mass of the plank is M/3, the distance that the man moves relative to the ground is
View SolutionShow that an organ pipe of length 2l open at both ends has the same fundamental frequency as another pipe of length I closed at one end.
View Solution
