A particle is moving along the axis under the influnence of a force given by . At time , the particle is located at and having zero velocity it take second to reach the origin for the first time. The equation of motion of the particle can be respected by
The correct Answer is:D
To solve the problem step by step, we will analyze the motion of the particle under the influence of the force given and derive the equation of motion.
Step 1: Analyze the Force
The force acting on the particle is given by:
F=−5x+15
This force can be rewritten as:
F=−5(x−3)
This indicates that there is a restoring force that acts towards the position x=3. Thus, x=3 is the equilibrium position (mean position) of the particle.
Step 2: Determine the Amplitude
At t=0, the particle is at x=6 with zero velocity. Since the particle is at its extreme position (maximum displacement), the amplitude A can be calculated as:
A=|6−3|=3
So, the amplitude of the motion is 3.
Step 3: Determine the Time Period
The problem states that it takes 0.5 seconds to reach the origin (which is x=0) for the first time. This means that the time taken to go from the maximum displacement (6) to the mean position (3) and then to the origin is half of the time period T:
0.5=T2
Thus, the time period T is:
T=1 second
Step 4: Calculate Angular Frequency
The angular frequency ω is given by:
ω=2πT=2π1=2π
Step 5: Write the General Equation of Motion
The general equation of motion for a simple harmonic oscillator can be written as:
x(t)=Acos(ωt+ϕ)+x0
where A is the amplitude, ω is the angular frequency, and ϕ is the phase constant. Since we have:
- Amplitude A=3
- x0=3 (mean position)
The equation becomes:
x(t)=3+3cos(2πt+ϕ)
Step 6: Determine the Phase Constant
At t=0, the position x(0)=6:
6=3+3cos(ϕ)
Subtracting 3 from both sides gives:
3=3cos(ϕ)
Thus:
cos(ϕ)=1
This implies:
ϕ=0
Final Equation of Motion
Substituting ϕ=0 into the equation gives:
x(t)=3+3cos(2πt)
Conclusion
The equation of motion of the particle is:
x(t)=3+3cos(2πt)
The force acting on the particle is given by:
F=−5x+15
This force can be rewritten as:
F=−5(x−3)
This indicates that there is a restoring force that acts towards the position x=3. Thus, x=3 is the equilibrium position (mean position) of the particle.
At t=0, the particle is at x=6 with zero velocity. Since the particle is at its extreme position (maximum displacement), the amplitude A can be calculated as:
A=|6−3|=3
So, the amplitude of the motion is 3.
The problem states that it takes 0.5 seconds to reach the origin (which is x=0) for the first time. This means that the time taken to go from the maximum displacement (6) to the mean position (3) and then to the origin is half of the time period T:
0.5=T2
Thus, the time period T is:
T=1 second
The angular frequency ω is given by:
ω=2πT=2π1=2π
The general equation of motion for a simple harmonic oscillator can be written as:
x(t)=Acos(ωt+ϕ)+x0
where A is the amplitude, ω is the angular frequency, and ϕ is the phase constant. Since we have:
- Amplitude A=3
- x0=3 (mean position)
x(t)=3+3cos(2πt+ϕ)
At t=0, the position x(0)=6:
6=3+3cos(ϕ)
Subtracting 3 from both sides gives:
3=3cos(ϕ)
Thus:
cos(ϕ)=1
This implies:
ϕ=0
Substituting ϕ=0 into the equation gives:
x(t)=3+3cos(2πt)
The equation of motion of the particle is:
x(t)=3+3cos(2πt)
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 6-previous year question
56 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (1)
75 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)
3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
Similar Questions
A particle moves along the X-axis x=0 to x=5 m under the influence of a force given by F=10−2x+3x2. Work done in the process is
View SolutionA particle moves along the X-axis from x=0 to x=5 m under the influence of a force given by F=7−2x+3x2. Find the work done in the process.
View SolutionA particle moves along the x-axis from x=0 to x=5m under the influence of a given by F=7−2x+3x2. The work done by the applied force to the particle is.
View SolutionA particle moves along x-axis from x=0 to x=2 m under the influence of a force F (in N) given by F=3x2+2x−5. Calculate the work done
View SolutionA particle is moving along the x-axis whose acceleration is given by a=3x−4, where x is the location of the particle. At t = 0, the particle is at rest at x=4/3m. The distance travelled by the particles in 5 s is
View SolutionA particle of mass 2kg is moving of a straight line under the action of force F=(8−2x)N. It is released at rest from x=6m.
a. Is the particle moving simple harmonically.
b. Find the equilibrium position of the particle.
c. Write the equation of motion of the particle.
d. Find the time period of SHM.
View SolutionA particle is moving on X−axis. Its velocity time graph is given in the figure. Find distance travelled by the particle in 5sec.
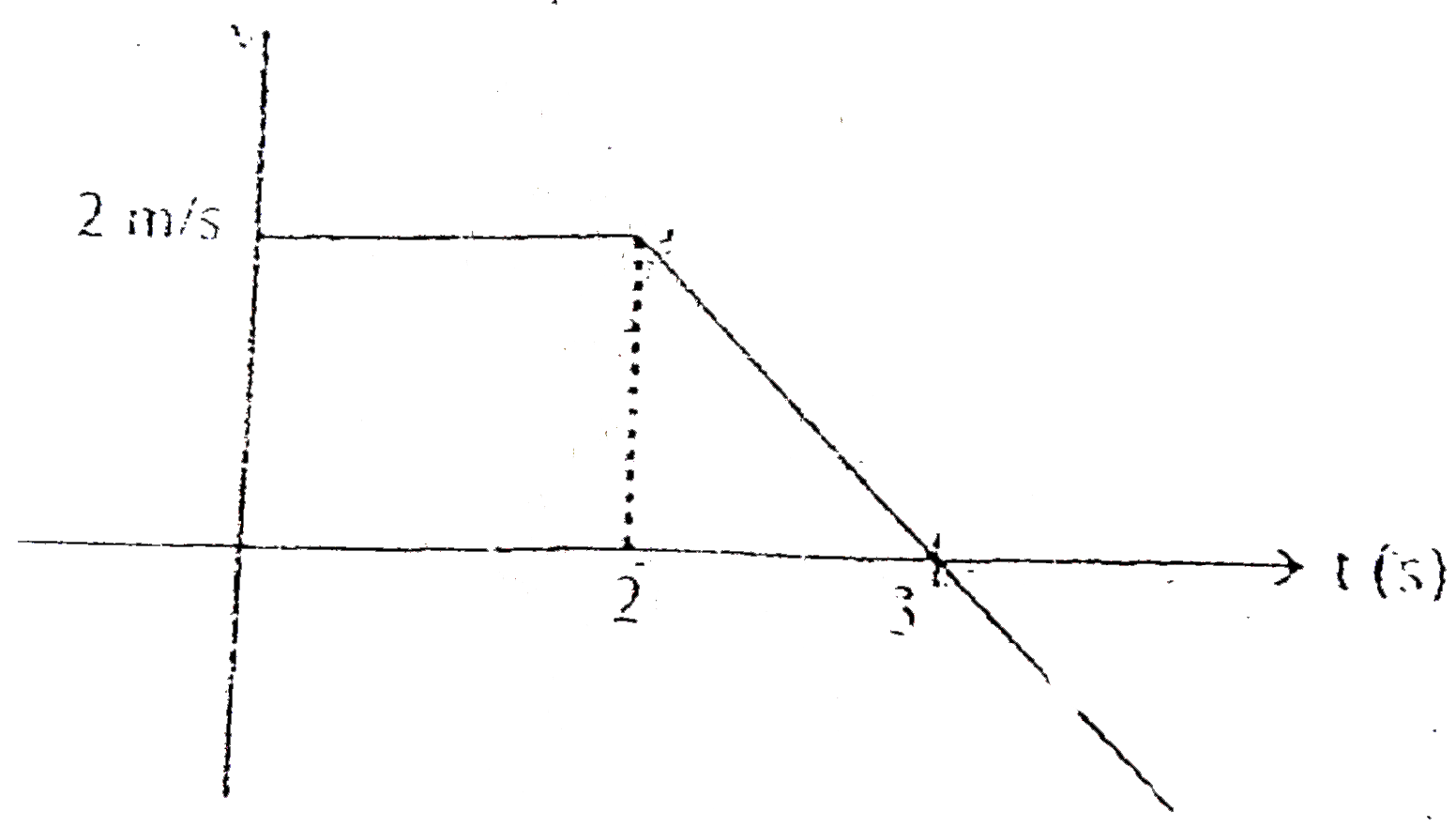
View SolutionA particle moves along x - axis under the action of a position dependent force F=(5x2−2x)N. Work done by force on the particle when it moves from origin to x = 3 m is
View SolutionA particle is moving with speed v=b√x along positive x-axis. Calculate the speed of the particle at time t=τ (assume tha the particle is at origin at t= 0).
View SolutionThe velocity of particle moving in the positive direction of x axis varies as v=α√x, where α is a positive constnat. Assuming that at moment t=0, the particle was located at the point x=0. find.
(a) the time dependence of the velocity and the acceleration of the particle.
(b) the mean velocity of the particle averaged over the time that the particle takes to cover first s meters of the path
View Solution
