The bob of a simple pendulum is displaced from its equilibrium position 'O' to a position 'Q' which is at a height 'h' above 'O' and the bob is then released. Assuming the mass of the bob to be 'm' and time period of oscillation to be 2.0s, the tension in the string when the bob passes through 'O' is
(a)m(g+2hπ2)
(b)m(g+√hπ2gh)
(c)m(g+√π22gh)
(d)m(g+√π23gh)
The correct Answer is:A
To find the tension in the string when the bob of a simple pendulum passes through its equilibrium position 'O', we can follow these steps:
Step 1: Understand the Forces Acting on the Bob
When the bob is at the lowest point (equilibrium position 'O'), two forces act on it:
1. The gravitational force (weight) acting downwards, which is mg.
2. The tension in the string T acting upwards.
Step 2: Apply Newton's Second Law
At the lowest point, the net force acting on the bob is given by:
T−mg=ma
Where a is the centripetal acceleration of the bob at point 'O'.
Step 3: Determine the Velocity at Point 'O'
When the bob is released from height 'h', it converts potential energy into kinetic energy. The potential energy at height 'h' is given by:
PE=mgh
At the lowest point 'O', this potential energy is converted into kinetic energy (KE):
KE=12mv2
Setting the potential energy equal to the kinetic energy:
mgh=12mv2
From this, we can solve for v2:
v2=2gh
Step 4: Substitute v2 into the Force Equation
Now, substituting v2 into the equation from Step 2:
T−mg=mv2L
Where L is the length of the pendulum. Substituting v2:
T−mg=m(2gh)L
Step 5: Solve for Tension T
Rearranging the equation gives:
T=mg+m(2gh)L
This can be simplified to:
T=mg+2mghL
Step 6: Using the Time Period to Find L
Given the time period T of the pendulum is 2.0s, we can use the formula for the time period of a simple pendulum:
T=2π√Lg
Squaring both sides:
T2=4π2Lg
From this, we can express L:
L=gT24π2
Step 7: Substitute L Back into the Tension Equation
Substituting L into the tension equation:
T=mg+2mgh(gT24π2)
This simplifies to:
T=mg+8π2mhT2
Step 8: Final Expression for Tension
Now, substituting T=2.0s:
T=mg+8π2mh4
T=mg+2π2mh
Conclusion
The tension in the string when the bob passes through 'O' is:
T=mg+2π2mh
When the bob is at the lowest point (equilibrium position 'O'), two forces act on it:
1. The gravitational force (weight) acting downwards, which is mg.
2. The tension in the string T acting upwards.
At the lowest point, the net force acting on the bob is given by:
T−mg=ma
Where a is the centripetal acceleration of the bob at point 'O'.
When the bob is released from height 'h', it converts potential energy into kinetic energy. The potential energy at height 'h' is given by:
PE=mgh
At the lowest point 'O', this potential energy is converted into kinetic energy (KE):
KE=12mv2
Setting the potential energy equal to the kinetic energy:
mgh=12mv2
From this, we can solve for v2:
v2=2gh
Now, substituting v2 into the equation from Step 2:
T−mg=mv2L
Where L is the length of the pendulum. Substituting v2:
T−mg=m(2gh)L
Rearranging the equation gives:
T=mg+m(2gh)L
This can be simplified to:
T=mg+2mghL
Given the time period T of the pendulum is 2.0s, we can use the formula for the time period of a simple pendulum:
T=2π√Lg
Squaring both sides:
T2=4π2Lg
From this, we can express L:
L=gT24π2
Substituting L into the tension equation:
T=mg+2mgh(gT24π2)
This simplifies to:
T=mg+8π2mhT2
Now, substituting T=2.0s:
T=mg+8π2mh4
T=mg+2π2mh
The tension in the string when the bob passes through 'O' is:
T=mg+2π2mh
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (2)40videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 6-previous year question56videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL 0 LONG ANSWER TYPE2videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)3videos
ROTATIONAL MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F10videos
SYSTEM OF A PARTICLES & ROTATIONAL MOTION
Similar Questions
A simple pendulum of length l and bob of mass m is displaced from its equilibrium position O to a position P so that hight of P above O is h . If is then released. What is the tension in the string when the bob passes through the through the equilibrium position O ? Neglect friction. v is the velocity of the bob at O
View SolutionThe string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionSuppose the amplitude of a simple pendulum having a bob of mass m is θ0. Find the tension in the string when the bob is at its extreme position.
View SolutionA simple pendulum is hanging from the roof of a trolley which is moving horizontally with acceleration g. If length of the string is L and mass of the bob is m, then time period of oscillation is
View SolutionThe bob of a simple pendulum of length /has a positive charge q on it. The pendulum is fixed to a horizontally oriented positively charged sheet as shown in the figure. The time period of the small oscillations of simple pendulum is
View SolutionThe free end of a simple pendulum is attached to the ceiling of a box. The box is taken to a height and the pendulum is oscillated. When the bob is at its lowest point the box is released to fall freely. As seen from the box during this period the bob will
View SolutionIf the inertial mass m1 of the bob of a simple pendulum of length 'l' is not equal to the gravitationalmassmg, then its period is
View SolutionThe bob A of a simple pendulum is released when the string makes an angle of 45∘ with the vertical. It hits another bob B of the same material and same mass kept at rest on the table. If the collision is elastic, then
 View Solution
View SolutionThe figure below shows a simple pendulum of mass 200 g. It is displaced from the mean position A to the extreme position B. The potential energy at the position A is zero. At the position B the pendulum bob is raised by 5 m.
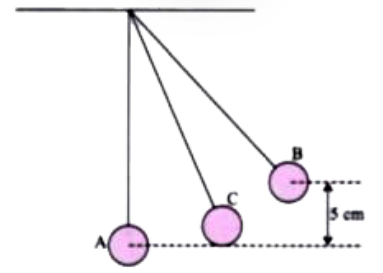
(i) What is the potential energy of the pendulum at the position B?
(ii) What is the total mechanical energy at point C?
(iii) What is the speed of the bob at the position A when released from B ?
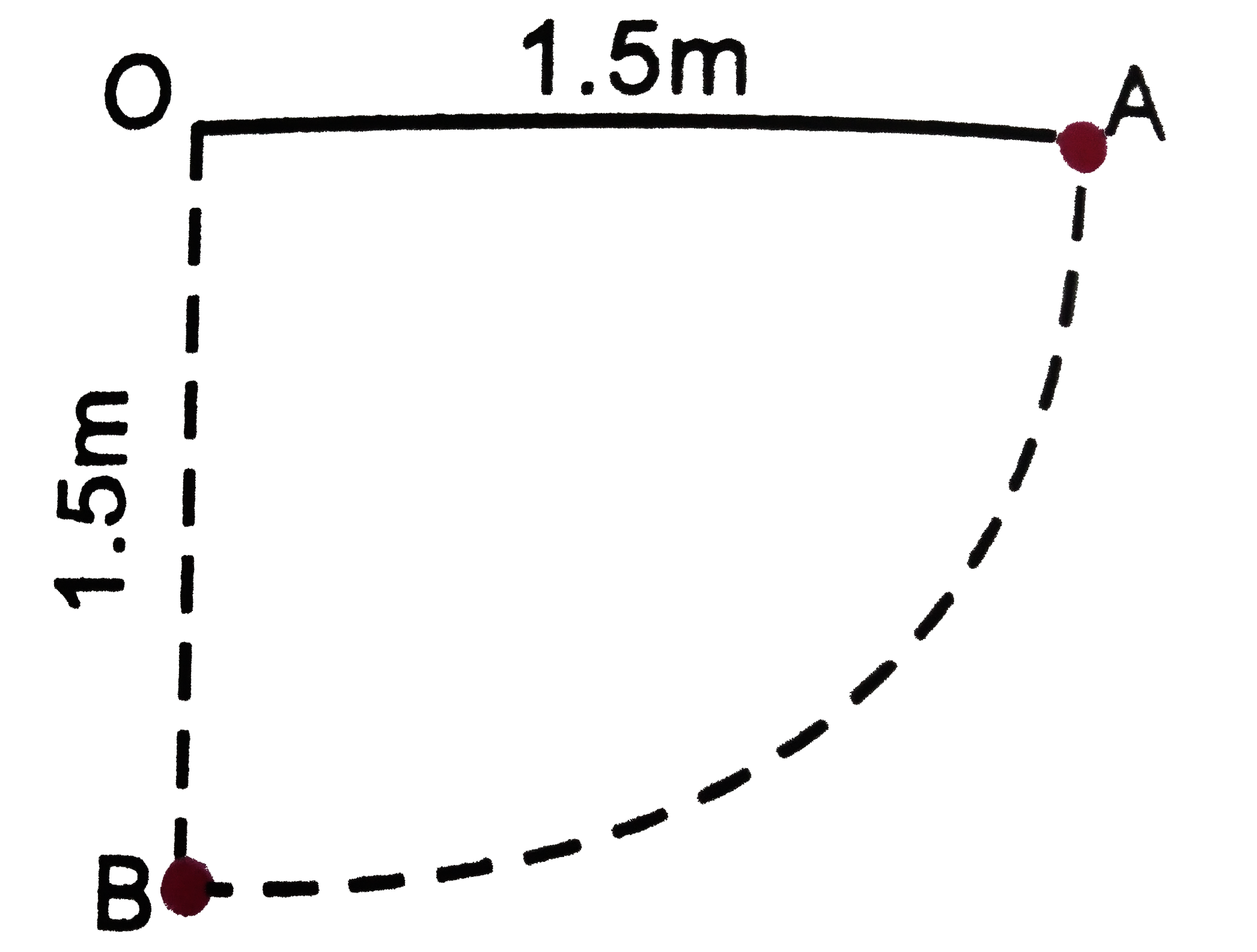
(Take g = 10ms−2 and there is no loss of energy.)View SolutionThe bob A of a simple pendulum is released from a horizontal position A as shownin in figure. If the length of the pendulum is 1.5m , what is the speed with which the bob arrives at the lowermost point B, given that it dissipates 5% of its initial energy against air resistance ?
 View Solution
View Solution
