Length of a sonometer wire is $1.21 m$ . Find the length of the three segments for fundamental frequencies to be in the ratio $1 : 2 : 3$ .

Video Solution

Text Solution

Verified by Experts

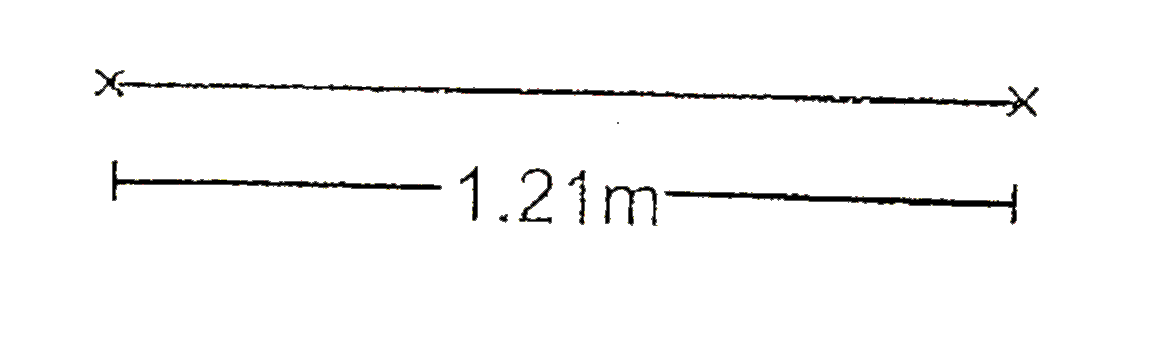
The correct Answer is: $0.66 m, 0.33 m, 0.22 m$

$n_{1} = \frac{1}{2 L_{1}} \sqrt{\frac{T}{μ}}$
$n_{2} = \frac{1}{2 L_{2}} \sqrt{\frac{T}{μ}}$
$n_{3} = \frac{1}{2 L_{3}} \sqrt{\frac{T}{μ}}$
$n_{1} : n_{2} : n_{3} : 1 : 2 : 3$
$n_{2} = 2 n_{1}$
$n_{3} = 3 n_{1}$
$L_{3} = \frac{n_{1}}{n_{2}} L_{1} = (\frac{L_{1}}{2})$
$L_{1} + L_{2} + L_{3} = 1.21$
$L_{1} (\frac{6 + 3 + 2}{6}) = 1.21$
$L_{1} (\frac{11}{6}) = 1.21$
$L_{1} = \frac{6 \times 121}{11 \times 100} = \frac{6 \times 11}{100} = 0.66 m$
$L_{2} = \frac{L_{1}}{L_{2}} = \frac{L_{1}}{2} = \frac{0.66}{20} = 0.33 m$
$L_{3} = \frac{L_{1}}{3} = \frac{0.66}{3} = 0.22 m$

Updated on:21/07/2023

|

Class 12 PHYSICS TRAVELLING WAVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The length of a sonometer wire AB is 110 cm. Where should the two bridges be placed from A to divide the wire in 3 segments whose fundamental frequencies are in the ratio of $1 : 2 : 3$ ?
A30 cm and 90 cm
B40 cm and 80 cm
C60 cm and 90 cm
D30 cm and 60 cm

RESONANCE ENGLISH-TRAVELLING WAVES-Exercise- 3 PART I

Home
Ask Doubt
Profile