A metal wire with volume density ρ and young's modulus Y is stretched between rigid supports. At temperature T, the speed of a transverse wave is found to be v1. When temperature decreases T−ΔT, the speed increases to v2. Datermine coefficient of linear expansion of wire.
To determine the coefficient of linear expansion of the wire, we will follow these steps:
Step 1: Understand the relationship between wave speed, tension, and mass density
The speed of a transverse wave in a wire is given by the formula:
v=√Fμ
where F is the tension in the wire and μ is the mass per unit length (linear density) of the wire, defined as:
μ=ρ⋅A
Here, ρ is the volume density, and A is the cross-sectional area of the wire.
Step 2: Relate tension to Young's modulus and temperature
From Hooke's Law, the tension F in the wire can be expressed as:
F=Y⋅ΔLL0⋅A
where Y is Young's modulus, ΔL is the change in length, and L0 is the original length of the wire. The change in length due to temperature change can be expressed as:
ΔL=α⋅L0⋅ΔT
where α is the coefficient of linear expansion, and ΔT is the change in temperature.
Step 3: Substitute the expression for tension into the wave speed formula
Substituting the expression for F into the wave speed formula gives:
v=
⎷Y⋅ΔLL0⋅Aρ⋅A=√Y⋅α⋅ΔTρ
This indicates that the speed of the wave depends on Young's modulus, the coefficient of linear expansion, the change in temperature, and the density.
Step 4: Analyze the change in wave speed with temperature
When the temperature decreases from T to T−ΔT, the speed of the wave changes from v1 to v2. Therefore, we can write:
v1=√Y⋅α⋅(T−T0)ρ
v2=√Y⋅α⋅(T−ΔT−T0)ρ
Step 5: Set up the equation for the change in speed
We can square both speeds and set up the equation:
v22−v21=Y⋅αρ((T−T0−ΔT)−(T−T0))
This simplifies to:
v22−v21=−Y⋅αρΔT
Step 6: Solve for the coefficient of linear expansion α
Rearranging the equation gives:
α=ρ(v22−v21)YΔT
Final Answer
Thus, the coefficient of linear expansion of the wire is given by:
α=ρ(v22−v21)YΔT
---
The speed of a transverse wave in a wire is given by the formula:
v=√Fμ
where F is the tension in the wire and μ is the mass per unit length (linear density) of the wire, defined as:
μ=ρ⋅A
Here, ρ is the volume density, and A is the cross-sectional area of the wire.
From Hooke's Law, the tension F in the wire can be expressed as:
F=Y⋅ΔLL0⋅A
where Y is Young's modulus, ΔL is the change in length, and L0 is the original length of the wire. The change in length due to temperature change can be expressed as:
ΔL=α⋅L0⋅ΔT
where α is the coefficient of linear expansion, and ΔT is the change in temperature.
Substituting the expression for F into the wave speed formula gives:
v=
⎷Y⋅ΔLL0⋅Aρ⋅A=√Y⋅α⋅ΔTρ
This indicates that the speed of the wave depends on Young's modulus, the coefficient of linear expansion, the change in temperature, and the density.
When the temperature decreases from T to T−ΔT, the speed of the wave changes from v1 to v2. Therefore, we can write:
v1=√Y⋅α⋅(T−T0)ρ
v2=√Y⋅α⋅(T−ΔT−T0)ρ
We can square both speeds and set up the equation:
v22−v21=Y⋅αρ((T−T0−ΔT)−(T−T0))
This simplifies to:
v22−v21=−Y⋅αρΔT
Rearranging the equation gives:
α=ρ(v22−v21)YΔT
Thus, the coefficient of linear expansion of the wire is given by:
α=ρ(v22−v21)YΔT
Topper's Solved these Questions
Similar Questions
A metal wire with density ρ and Young's modulus Y is stretched between rigid support At temperature T the speed of transverse wave is found to be v1. When temperature is increased to T+ΔT the speed decreases to v2<v1. If the change in cross section area of the wire is ignored then the coefficient of linear expansion of the wire is
View SolutionA string of density 7.5gcm−3 and area of cross - section 0.2mm2 is stretched under a tension of 20 N. When it is plucked at the mid-point, the speed of the transverse wave on the wire is
View Solution
Knowledge Check
The speed of sound through oxygen gas at T K is vms−1.As the temperature becomes 2T and oxygen gas dissociated into atomic oxygen, the speed of sound
A1. remains the sameB2. become 2vC3. become √2vD4. none of these
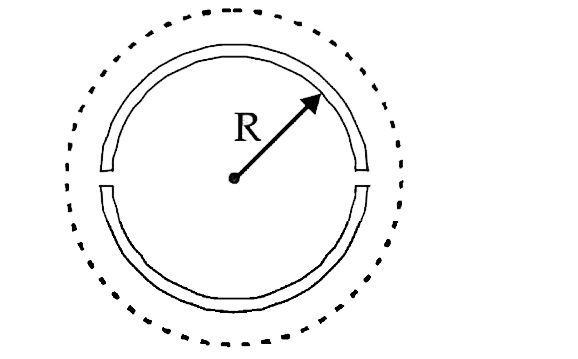
A wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
View SolutionA simple pendulum made of bob of mass m and a metallic wire of negligible mass has time period 2s at T=0∘C. If the temperature of the wire is increased and the corresponding change in its time period is plotted against its temperature, the resulting graph is a line of slope S. If the coefficient of linear expansion of metal is α then value of S is :
View SolutionA metallic wire with tension T and at temperature 30∘C vibrates with its fundamental frequency of 1kHz. The same wire with the same tension but at 10∘C temperature vibrates with a fundamental frequency of 1.001kHz. The coefficient of linear expansion of the wire is equal to 10−K.∘C. Find 2K.
View SolutionA uniform metallic disc of radius r and mass m is spinning with angular speed ω0 about an axis passing through its centre and perpendicular to plane. If its temperature is increased (slightly) by ΔT its new angular speed is (The coefficient of linear expansion of the metal is α)
View SolutionTwo rods of different materials having coefficient of thermal expansion α1 and α2 and Young's modulus Y1 and Y2 respectively are fixed between two rigid supports separately. The rods are heated such that they undergo same temperature increment. If thermal stress developed in two rods are equal, then Y1:Y2 is
View SolutionA metallic wire of length l is held between two rigid supports. If the wire is cooled through a temperature t. (Y= Young's modulus of elasticity of wire, ρ= density, α= thermal coefficient of linear expansion). Then the frequency of oscillation is proportional to
View SolutionA wire 2 mm in diameter is just stretched in between two fixed points at a temperature of 50∘C. Calculate the tension in the wire, when the temperature falls to 30∘C. Coefficient of linear expansion is 11×10−4l∘C and Young modulus is 2.1×1011Nm2
View Solution