A steel wire of length 50√3cm is connected to an aluminium wire of length 60cm and stretched between two fixed supports. The tension produced is 104N, if the cross section area of each wire is 1mm2. If a transverse wave is set up in the wire, find the lowest frequency for which standing waves with node at the joint are produced . (density of aluminimum =2.6gm/cm3 and density of steel =7.8gm/cm3).
The correct Answer is:1000/3Hz
To solve the problem step by step, we need to find the lowest frequency for which standing waves with a node at the joint of the steel and aluminum wires are produced.
Step 1: Understand the Setup
We have two wires:
- A steel wire of length L1=50√3cm
- An aluminum wire of length L2=60cm
Both wires are under the same tension T=104N and have the same cross-sectional area A=1mm2=1×10−6m2.
Step 2: Calculate the Linear Densities
The linear density μ of a wire is given by:
μ=masslength=ρ⋅AL
Where ρ is the density of the material.
- For steel:
ρsteel=7.8g/cm3=7800kg/m3
μ1=7800×1×10−60.5√3kg/m
- For aluminum:
ρaluminum=2.6g/cm3=2600kg/m3
μ2=2600×1×10−60.6kg/m
Step 3: Calculate the Velocities of Waves in Each Wire
The velocity v of a wave in a wire is given by:
v=√Tμ
- For steel:
v1=√104μ1
- For aluminum:
v2=√104μ2
Step 4: Determine the Frequencies
The frequency f of the standing wave is given by:
f=nv2L
Where n is the mode number, v is the wave velocity, and L is the length of the wire.
For the lowest frequency with a node at the joint, we have:
n1v12L1=n2v22L2
Step 5: Relate the Frequencies
From the previous relation, we can derive:
n1n2=v2L1v1L2
Step 6: Substitute Values
Substituting the expressions for v1 and v2:
n1n2=√104μ2⋅L1√104μ1⋅L2
This simplifies to:
n1n2=L1L2⋅√μ1μ2
Step 7: Calculate n1n2
Substituting the lengths and densities:
n1n2=50√360⋅√78002600=5√36⋅√3=52
Step 8: Choose the Lowest Values for n1 and n2
To find the lowest frequency, we can take n2=2 (the smallest integer) and n1=5.
Step 9: Calculate the Frequency
Using n2=2:
f=2⋅v22L2=v2L2
Substituting v2:
f=160√104μ2
Step 10: Final Calculation
Substituting the values and calculating gives:
f=160
⎷1042600×1×10−60.6
This results in:
f≈10003Hz
Final Answer
The lowest frequency for which standing waves with a node at the joint are produced is approximately:
f≈333.33Hz
We have two wires:
- A steel wire of length L1=50√3cm
- An aluminum wire of length L2=60cm
The linear density μ of a wire is given by:
μ=masslength=ρ⋅AL
Where ρ is the density of the material.
ρsteel=7.8g/cm3=7800kg/m3
μ1=7800×1×10−60.5√3kg/m
ρaluminum=2.6g/cm3=2600kg/m3
μ2=2600×1×10−60.6kg/m
The velocity v of a wave in a wire is given by:
v=√Tμ
v1=√104μ1
v2=√104μ2
The frequency f of the standing wave is given by:
f=nv2L
Where n is the mode number, v is the wave velocity, and L is the length of the wire.
n1v12L1=n2v22L2
From the previous relation, we can derive:
n1n2=v2L1v1L2
Substituting the expressions for v1 and v2:
n1n2=√104μ2⋅L1√104μ1⋅L2
This simplifies to:
n1n2=L1L2⋅√μ1μ2
Substituting the lengths and densities:
n1n2=50√360⋅√78002600=5√36⋅√3=52
To find the lowest frequency, we can take n2=2 (the smallest integer) and n1=5.
Using n2=2:
f=2⋅v22L2=v2L2
Substituting v2:
f=160√104μ2
Substituting the values and calculating gives:
f=160
⎷1042600×1×10−60.6
This results in:
f≈10003Hz
The lowest frequency for which standing waves with a node at the joint are produced is approximately:
f≈333.33Hz
Topper's Solved these Questions
Similar Questions
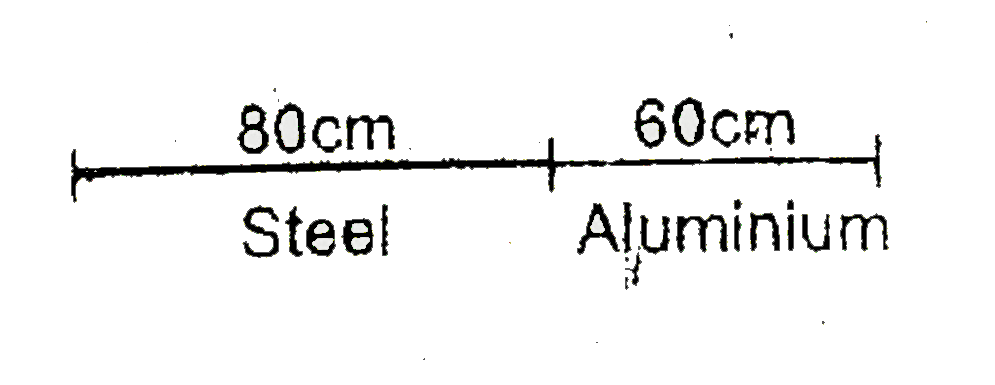
Figure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
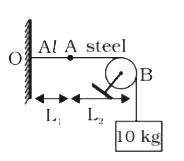
View SolutionAn aluminium wire and a steel wire of same cross-sectional of 10−2cm2 were connected together. A 10 kg block loaded on the compound wire as shown in figure. Waves are set up in the compound wire. The minimum frequency of excitation for which standing waves are observed with point A as a node is [L1=60cm,L2=86.6cm] density of aluminium =2.6g/cm3 and of steel=7.8g/cm3]
View SolutionA solid sphere of radius 1cm is melted to stretch into a wire of length 100 cm. Find the radius of the wire.
View SolutionA 40 cm wire having a mass of 3.2 g is stretched between two fixed supports 40.05 cm apart. In its fundamental mode, the wire vibrates at 220 Hz. If the area of cross section of the wire is 1.0mm2, find its Young modulus.
View SolutionA 40 cm wire having a mass of 3.2 g is stretched between two fixed supports 40.05 cm apart. In its fundamental mode, the wire vibrates at 220 Hz. If the area of cross section of the wire is 1.0mm2, find its Young modulus.
View SolutionA wire having a linear density of 0.05g/cmis stretched between two rigid supports with a tension of 450N. It is observed that the wire resonates at a frequency of 420 Hz. The next higher frequency at which the same wire resonates is 490 Hz. Find the length of the wire.
View SolutionA wire having a linear density of 0.05g/cmis stretched between two rigid supports with a tension of 450N. It is observed that the wire resonates at a frequency of 420Hz. The next higher frequency at which the same wire resonates is 490Hz. Find the length of the wire.
View SolutionWhen a wire of length 5m and diameter 1mm was stretched by a load of 5kg the elongation produced in the wire was 1mm. Find the energy stored in per unit volume of the wire?
View Solution
A steel wire of length 60 cm and area of cross section 10−6m2 is joined with a n aluminium wire of length 45 cm and are of cross section 3×10−6m2. The composite string is stretched by a tension of 80 N. Density of steel is 7800kgm−3 and that of aluminium is 2600kgm−3 the minimum frequency of tuning fork. Which can produce standing wave in it with node at joint is
View SolutionAn aluminium wire of cross-sectional area (10−6)m2 is joined to a steel wire of the same cross-sectional area. This compound wire is stretched on a sonometer pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m of which the aluminium wire is 0.6m and the rest is steel wire. Transverse vibrations are setup in the wire by using an external source of variable frequency. Find the lowest frequency of excitation for which the standing waves are formed such that the joint in the wire is a node. What is the total number of nodes at this frequency? The density of aluminium is 2.6×(103)kg/m3 and that of steel is 1.04×104kg/m2(g=10m/s2)
View Solution
