A paricle of mass 30kg executes a simple harmonic motion. The restorting force is provided by a spring of spring constant 80N/m. Find the time period.
To find the time period of a particle executing simple harmonic motion (SHM) when attached to a spring, we can follow these steps:
1. Identify the Given Values:
- Mass of the particle (m) = 30 kg
- Spring constant (k) = 80 N/m
2. Recall the Formula for Time Period (T):
The time period (T) of a mass-spring system undergoing simple harmonic motion is given by the formula:
T=2π√mk
3. Substitute the Given Values into the Formula:
Plugging in the values of mass and spring constant into the formula:
T=2π√30kg80N/m
4. Simplify the Expression Inside the Square Root:
Calculate the fraction:
3080=38
Thus, we have:
T=2π√38
5. Calculate the Square Root:
Now, we compute the square root:
√38=√3√8=√32√2=√32⋅2√2/2=√34√2=√68
6. Final Calculation of Time Period:
Substitute back into the time period formula:
T=2π⋅√68=π√64
7. Conclusion:
The time period of the particle executing simple harmonic motion is:
T≈1.225seconds(using π≈3.14)
- Mass of the particle (m) = 30 kg
- Spring constant (k) = 80 N/m
The time period (T) of a mass-spring system undergoing simple harmonic motion is given by the formula:
T=2π√mk
Plugging in the values of mass and spring constant into the formula:
T=2π√30kg80N/m
Calculate the fraction:
3080=38
Thus, we have:
T=2π√38
Now, we compute the square root:
√38=√3√8=√32√2=√32⋅2√2/2=√34√2=√68
Substitute back into the time period formula:
T=2π⋅√68=π√64
The time period of the particle executing simple harmonic motion is:
T≈1.225seconds(using π≈3.14)
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Solved Miscellaneous Problems
Explore 9 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Board Level Exercise
Explore 24 Videos
- SEMICONDUCTORSBook:RESONANCE ENGLISHChapter:SEMICONDUCTORSExercise:Exercise 3
Explore 88 Videos
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PHYSICS
Explore 784 Videos
Similar Questions
A particle of mass 200g executes a simple harmonic motion. The restoring force is provided by a spring of spring constant 80N/m. Find the time period.
View SolutionA particle of mass 200 g executes a simpel harmonit motion. The resrtoring force is provided by a spring of spring constant 80Nm−1. Find the time period.
View Solution
Knowledge Check
A particle executing simple harmonic motion with an amplitude A. The distance travelled by the particle in one time period is
AzeroBAC2AD4A
A particle executes simple harmonic motion under the restoring force provided by a spring. The time period is T. If the spring is divided in two equal parts and one part is used to continue the simple harmonic motion, the time period will
View SolutionA block of mass 5 kg executes simple harmonic motion under the restoring force of a spring. The amplitude and the time period of the motion are 0.1 m and 3.14 s respectively. Find the maximum force exerted by the spring on the block.
View SolutionA particle moving along the X-axis executes simple harmonic motion, then the force acting on it is given by
where, A and K are positive constants.
View SolutionA 1kg block is executing simple harmonic motion of amplitude 0.1m on a smooth horizontal surface under the restoring force of a spring of spring constant 100N/m. A block of mass 3kg is gently placed on it at the instant it passes through the mean position. Assuming that the two blocks move together. Find the frequency and the amplitude of the motion.
View SolutionA particle of mass 0.50 kg executes a simple harmonic motion under a force F=−(50Nm−1)x. If it crosses the centre of oscillation with a speed of 10ms−1, find the amplitude of the motion.
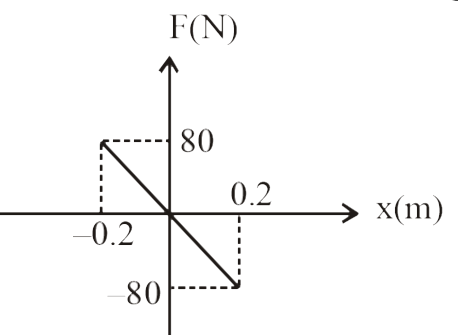
View SolutionA body of mass 0.01 kg executes simple harmonic motion about x = 0 under the influence of a force as shown in figure. The time period of SHM is
View SolutionA horizontal spring block system of mass M executes simple harmonic motion. When the block is passing through its equilibrium position, an object of mass m is put on it and the two move together. Find the new amplitude and frequency of vibration. Given, k is the spring constant of the system.
View Solution
