Consider two perpendicular mirrors M1 and M2 and a point object O. Taking origin at the point of intersection of the mirrros and the coordinates of object as (x,y), find the position and number of images.
Rays 'a' and 'b' strike mirror M1 only and these rays will from image I1 at (x,−y) such that O and I10 are equidisant form mirror M1. These rays do not form further image because they do not strike any mirror again. Similarly rays 'd' and 'e' strike any mirror M2 only and these rays will form image I2 at (−x,y) such that O and I2 are equidistant form mirror M2.

Now consider those rays whic strike mirror M2 first and then the mirror M1
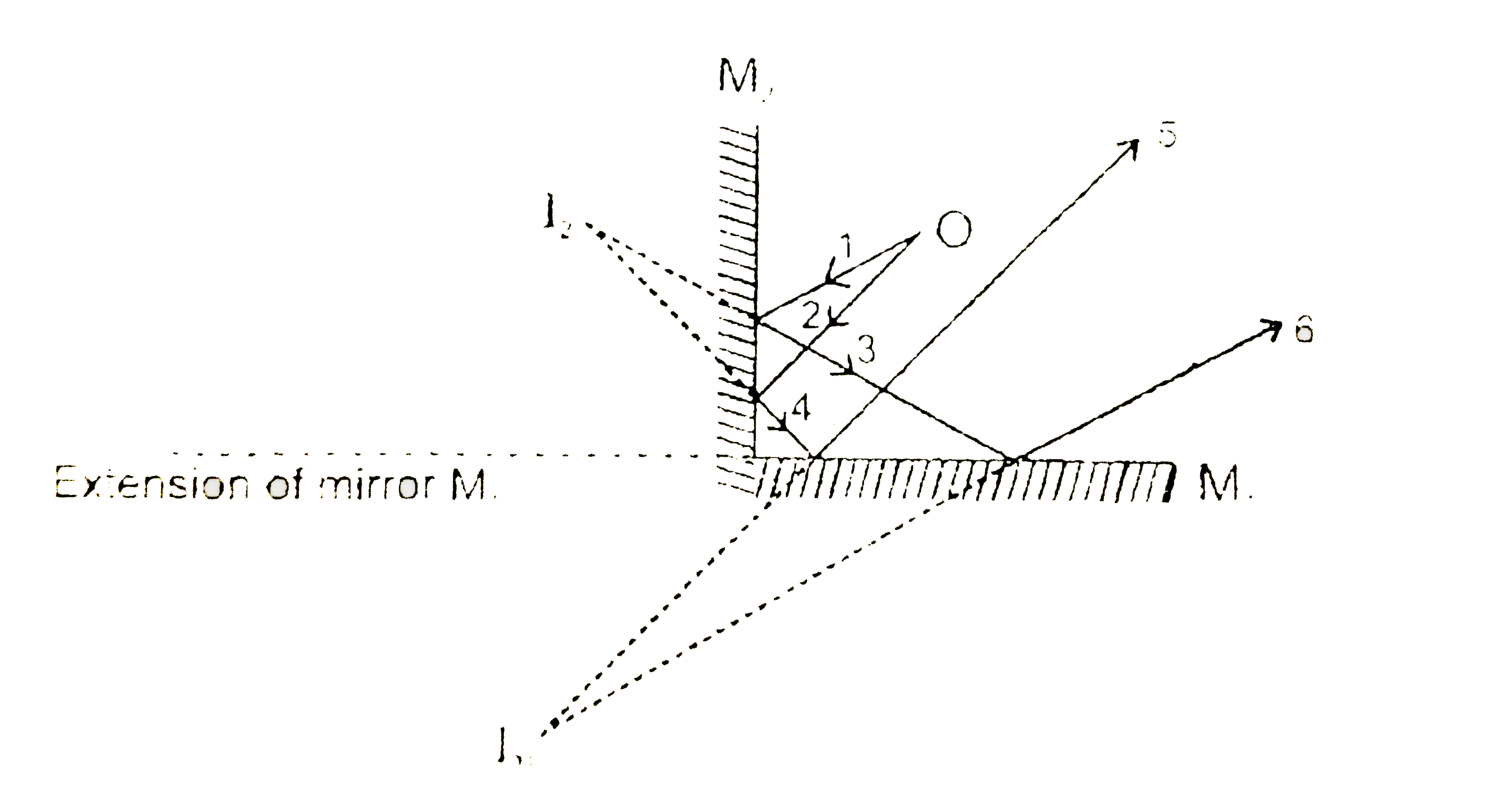
For incident rays 1,2 object is O, and reflect rays 3,4 from image I2
Now rays 3,4 incident on M1 (object is I2) which reflect as rays 5,6 and form image I2. Rays 5,6 do not strike any mirror, so image formation stops.
I2 and I21, are equildisatant form M1. To summarize see the following figure.
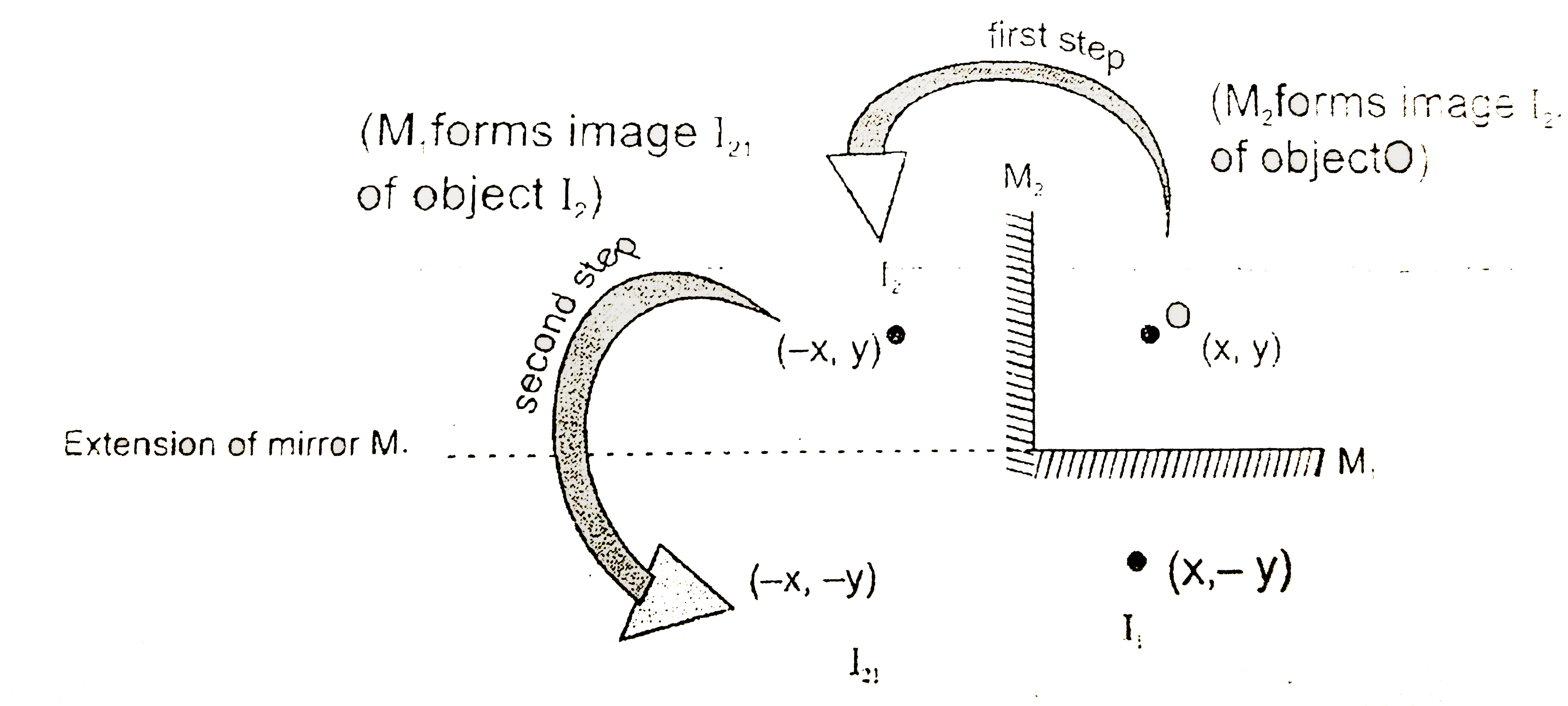
For rays reflecting first form M1 and then form M2 first image I1 (at (x,−y) ) will be formed adn this will funcion as object for mirror M2 and then its image I12 (at (x,−y)) will be formed I12 and I21 coincide.
Three images are formed.
Topper's Solved these Questions
- GEOMATRICAL OPTICS Book:RESONANCE ENGLISHChapter:GEOMATRICAL OPTICS Exercise:Problem
Explore 33 Videos
- GEOMATRICAL OPTICS Book:RESONANCE ENGLISHChapter:GEOMATRICAL OPTICS Exercise:Exercise-1
Explore 141 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCE ENGLISHChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
- GRAVITATIONBook:RESONANCE ENGLISHChapter:GRAVITATIONExercise:HIGH LEVEL PROBLEMS
Explore 16 Videos
Similar Questions
If the straight lines joining the origin and the points of intersection of y=mx+1andx2+y2=1 are perpendicular to each other, then find the value of m.
View SolutionA concave mirror forms an image I corresponding to a point object O . The equation of the circle intercepted by the xy plane on the mirror is
_S01_051_Q01.png)
View SolutionFind the point of intersection of the following pairs of lines: y=m1x+am1 and y=m2x+am2.
View SolutionIf the straight lines joining origin to the points of intersection of the line x+y=1 with the curve x2+y2+x−2y−m=0 are perpendicular to each other , then the value of m should be
View SolutionThe diagram shows a point object P in front of a plane mirror MM1.
In the diagram, mark the position of eye to see the image.
View SolutionFind the image of the point (1,−2) with respect to the line mirror 2x−y+1=0
View SolutionFind the locus of the point of intersection of the lines x=am2andy=2am, where m is a parameter.
View SolutionDraw the graphs of y=x and y=−x in the same graph. Also, find the coordinates of the point where the two lines intersect.
View SolutionDraw the graphs of y=x and y=−x in the same graph. Also, find the coordinates of the point where the two lines intersect.
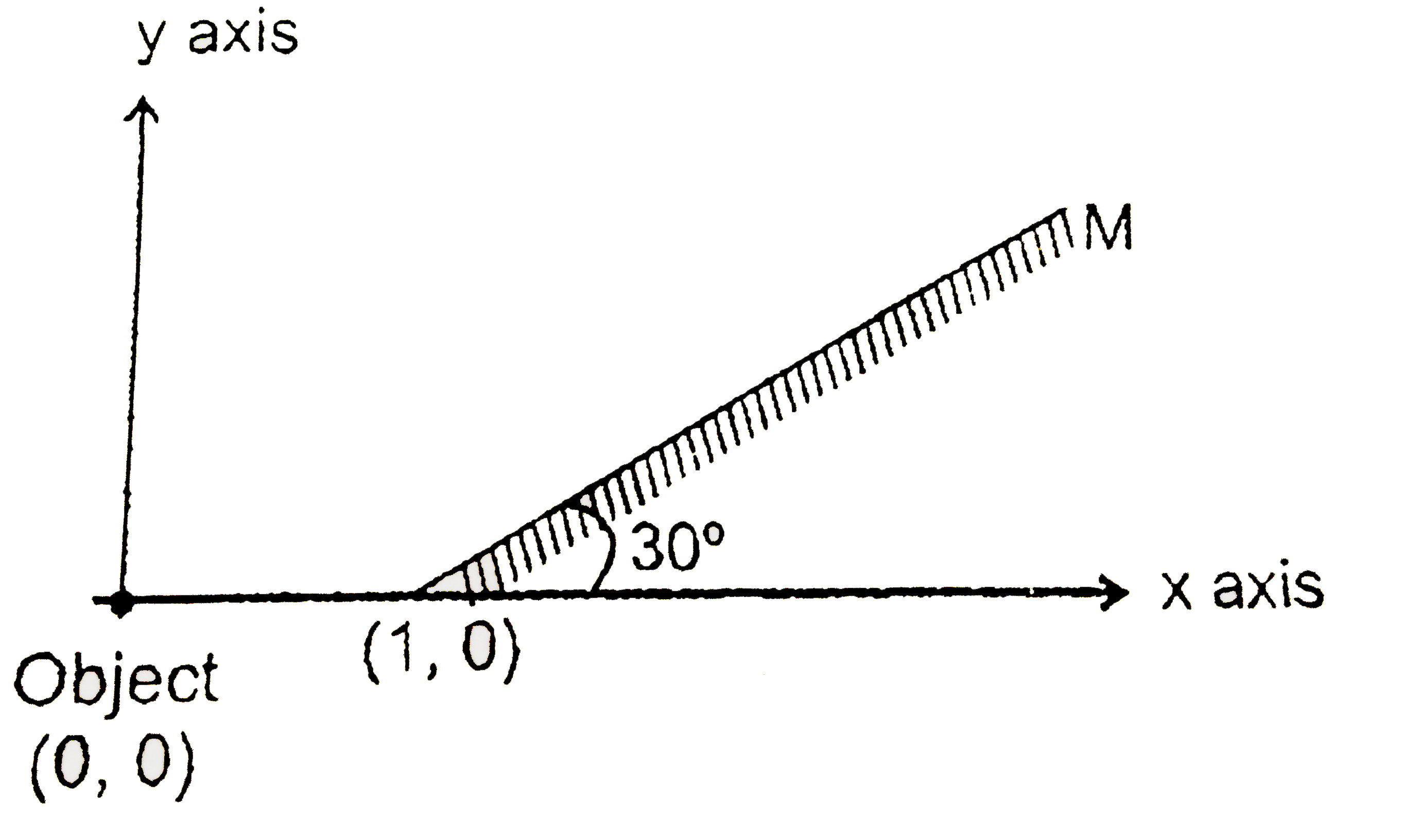
View SolutionA point object is placed (0,0) and a plane mirror 'M' is placed, inclined 30∘ with the x axis.
Find the position of image.
View Solution