A particle having a charge20μC and mass 20μg moves along a circle of radius 5.0 cm under the action of a magnetic field 5.0 cm under the action of a magnetic field B = 1.0 T. When the particle is at a point P, a uniform electric field is switched on and it is found that the particla continues on the tangent through P qith a uniform velocity. Find the electric field.
0.1 V/m
0.5 V/m
10.0 V/m
100 V/m
The correct Answer is:B
To solve the problem step by step, we will follow the principles of motion in magnetic and electric fields.
Step 1: Identify the given values
- Charge of the particle, Q=20μC=20×10−6C
- Mass of the particle, m=20μg=20×10−9kg
- Radius of the circular path, R=5.0cm=5.0×10−2m
- Magnetic field strength, B=1.0T
Step 2: Use the formula for the radius of circular motion in a magnetic field
The radius R of the circular path of a charged particle moving in a magnetic field is given by the formula:
R=mvBQ
Where:
- v is the velocity of the particle.
Step 3: Rearrange the formula to find the velocity
Rearranging the formula to solve for v:
v=R⋅B⋅Qm
Step 4: Substitute the known values into the equation
Substituting the values we have:
v=(5.0×10−2)⋅(1.0)⋅(20×10−6)20×10−9
Step 5: Calculate the velocity
Calculating the above expression:
v=(5.0×10−2)⋅(1.0)⋅(20×10−6)20×10−9=(5.0×20)×10−820×10−9=100×10−820×10−9=10020×101=5.0m/s
Step 6: Find the electric field E
When the electric field is switched on, the particle continues to move with uniform velocity along the tangent. This means the electric force must balance the magnetic force acting on the particle.
The magnetic force FB is given by:
FB=QvB
The electric force FE is given by:
FE=QE
Since these forces are equal when the particle moves with uniform velocity:
QE=QvB
Step 7: Solve for the electric field E
Cancelling Q from both sides (since Q≠0):
E=vB
Step 8: Substitute the values to find E
Substituting the values we calculated:
E=(5.0)⋅(1.0)=5.0V/m
Step 9: Final result
Thus, the electric field E is:
E=5.0V/m
- Charge of the particle, Q=20μC=20×10−6C
- Mass of the particle, m=20μg=20×10−9kg
- Radius of the circular path, R=5.0cm=5.0×10−2m
- Magnetic field strength, B=1.0T
The radius R of the circular path of a charged particle moving in a magnetic field is given by the formula:
R=mvBQ
Where:
- v is the velocity of the particle.
Rearranging the formula to solve for v:
v=R⋅B⋅Qm
Substituting the values we have:
v=(5.0×10−2)⋅(1.0)⋅(20×10−6)20×10−9
Calculating the above expression:
v=(5.0×10−2)⋅(1.0)⋅(20×10−6)20×10−9=(5.0×20)×10−820×10−9=100×10−820×10−9=10020×101=5.0m/s
When the electric field is switched on, the particle continues to move with uniform velocity along the tangent. This means the electric force must balance the magnetic force acting on the particle.
FB=QvB
FE=QE
QE=QvB
Cancelling Q from both sides (since Q≠0):
E=vB
Substituting the values we calculated:
E=(5.0)⋅(1.0)=5.0V/m
Thus, the electric field E is:
E=5.0V/m
Topper's Solved these Questions
Similar Questions
A particle having a charge20μC and mass 20μg moves along a circle of radius 5.0 cm under the action of a magnetic field B = 1.0 T. When the particle is at a point P, a uniform electric field is switched on and it is found that the particle continues on the tangent through P with a uniform velocity. Find the electric field.
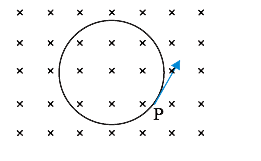
View SolutionA particle having charge of 20μC and mass 20μg moves along as circle of radius 5cm under the action of a magnetif field B=0.1 tesla. When the particle is at P, uniform transverse electric field is switched on an it is found that the particle continues along the tangent with a uniform velocity. Find the electric field
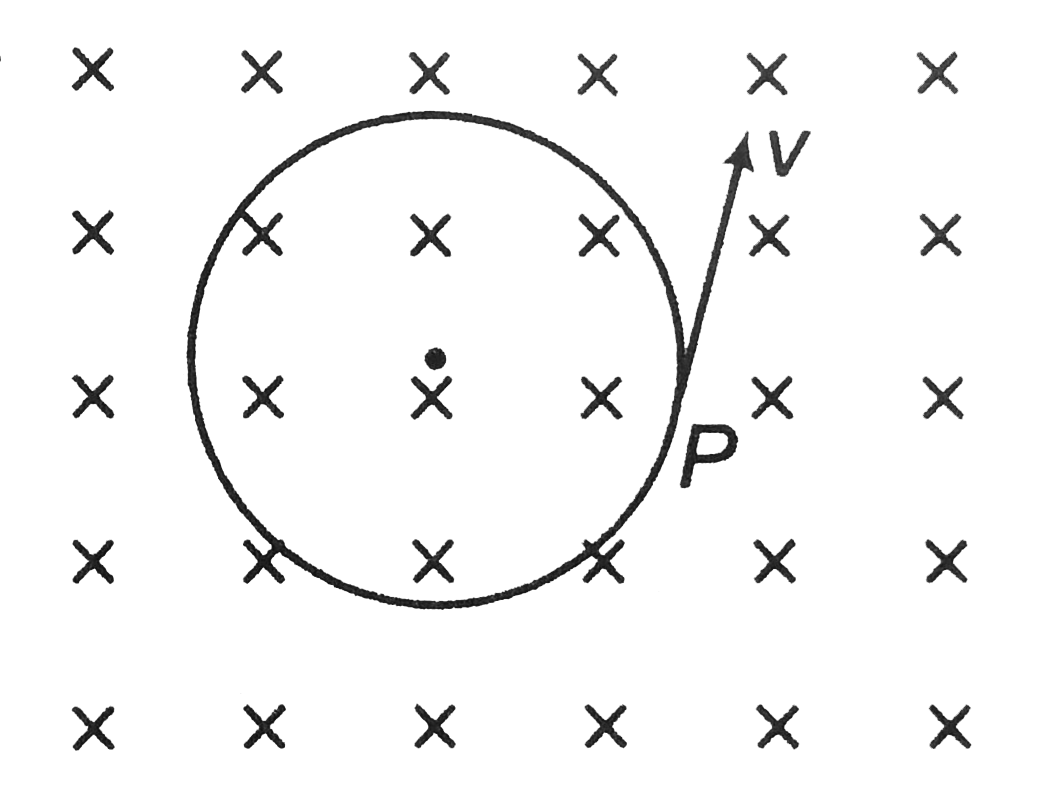
View SolutionA particle having a charge of 10.0μC and mass 1μg moves in a circle of radius 10 cm under the influence of a magnetic field of induction 0.1T. When the particle is at a point P, a uniform electric field is switched on so that the particle starts moving along the tangent with a uniform velocity. The electric field is
View SolutionIf a charged particle is a plane perpendicular to a uniform magnetic field with a time period T Then
View SolutionWhen a charged particle enters a uniform magnetic field its kinetic energy
View SolutionA particle moves in a circle of diamater 1.0 cm under the action of magnetic field of 0.40 T, An electric field of 200Vm−1 makes the path straight. Find the charges/mass ratio of the particle.
View SolutionA charged particle moves in a uniform magnetic field B=(2ˆi−3ˆj) T
View SolutionA charged particle moves along a circle under the action of possible constant electric and magnetic fields. Which of the following are possible?
View SolutionIf a charged particle enters perpendicular in the uniform magnetic field then
View SolutionA charged particle of charge 1 mC and mass 2g is moving with a speed of 5m/s in a uniform magnetic field of 0.5 tesla. Find the maximum acceleration of the charged particle
View Solution