Question

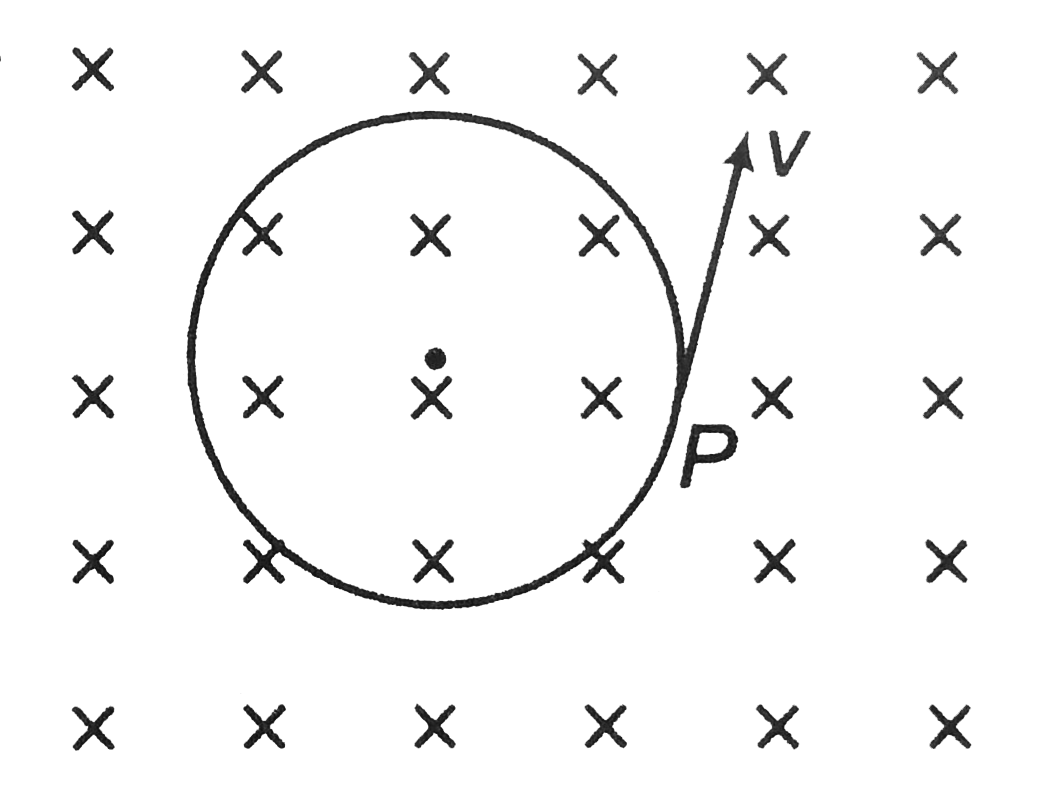
A particle having a charge$20\mu C$ and mass $20\mu g$ moves along a circle of radius 5.0 cm under the action of a magnetic field B = 1.0 T. When the particle is at a point P, a uniform electric field is switched on and it is found that the particle continues on the tangent through P with a uniform velocity. Find the electric field.

Explanation

To solve the problem step by step, we will follow the principles of motion in magnetic and electric fields.

Step 1: Understand the forces acting on the particle
The particle is moving in a circular path due to the magnetic force, and when the electric field is applied, it continues to move tangentially. This implies that the magnetic force acting on the particle is equal to the electric force when the electric field is switched on.

Step 2: Write the expressions for the forces
The magnetic force \( F_m \) acting on a charged particle moving in a magnetic field is given by:
\( F_m = Q \cdot V \cdot B \)
where:
- \( Q \) is the charge of the particle,
- \( V \) is the velocity of the particle,
- \( B \) is the magnetic field strength.

The electric force \( F_e \) acting on the charged particle in an electric field is given by:
\( F_e = Q \cdot E \)
where:
- \( E \) is the electric field strength.

Step 3: Set the forces equal to each other
Since the particle continues to move with uniform velocity when the electric field is applied, we can set the two forces equal:
\( F_m = F_e \)
Thus,
\( Q \cdot V \cdot B = Q \cdot E \)
We can simplify this equation (assuming \( Q \neq 0 \)):
\( V \cdot B = E \)

Step 4: Find the velocity \( V \) of the particle
The velocity of a particle moving in a circular path under the influence of a magnetic field can be expressed as:
\( R = \frac{mV}{QB} \)
Rearranging this gives:
\( V = \frac{QRB}{m} \)
where:
- \( R \) is the radius of the circular path,
- \( m \) is the mass of the particle.

Step 5: Substitute the values into the velocity equation
Given:
- \( Q = 20 \mu C = 20 \times 10^{-6} C \)
- \( B = 1.0 T \)
- \( R = 5.0 cm = 5.0 \times 10^{-2} m \)
- \( m = 20 \mu g = 20 \times 10^{-9} kg \)

Substituting these values into the velocity equation:
\( V = \frac{(20 \times 10^{-6} C)(5.0 \times 10^{-2} m)(1.0 T)}{20 \times 10^{-9} kg} \)

Step 6: Calculate the velocity \( V \)
Calculating the above expression:
\( V = \frac{(20 \times 10^{-6})(5.0 \times 10^{-2})(1.0)}{20 \times 10^{-9}} = \frac{(1.0 \times 10^{-6})}{20 \times 10^{-9}} = 50 \, m/s \)

Step 7: Substitute \( V \) back into the electric field equation
Now, substituting \( V \) back into the equation \( E = V \cdot B \):
\( E = (50 \, m/s)(1.0 \, T) = 50 \, V/m \)

Conclusion
The electric field \( E \) required to maintain the particle's motion along the tangent is:
\( E = 50 \, V/m \)