Question

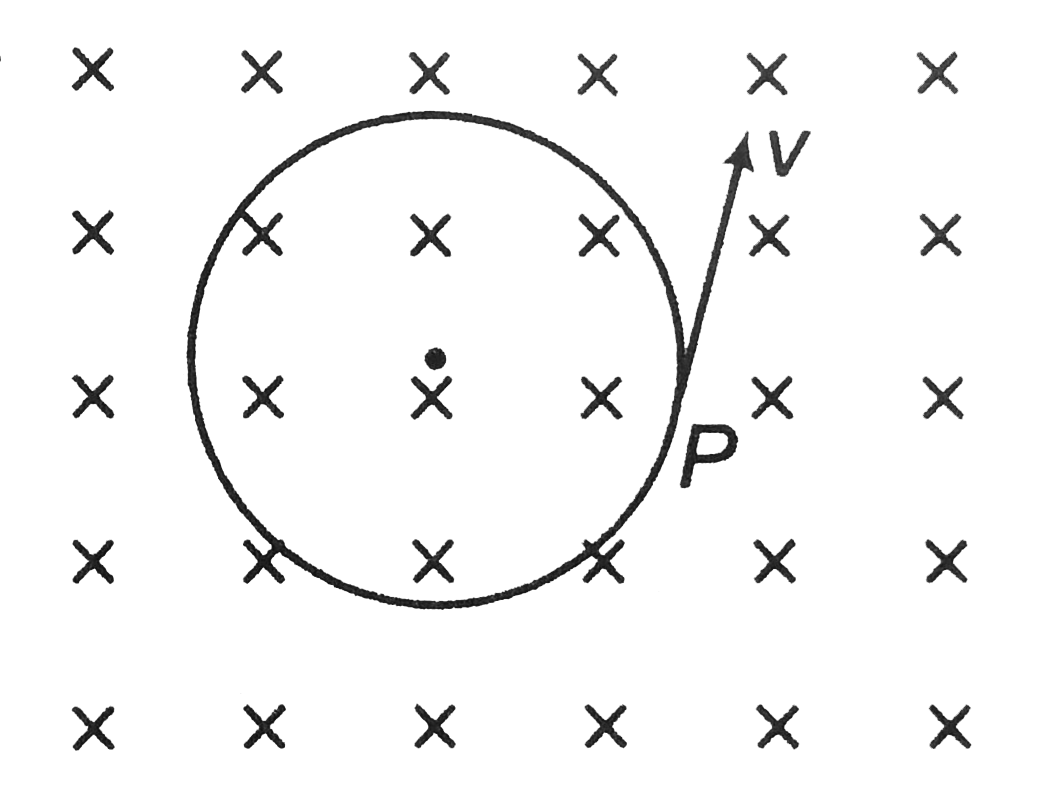
A particle moves in a circle of diamater 1.0 cm under the action of magnetic field of 0.40 T, An electric field of $200V{m}^{-1}$ makes the path straight. Find the charges/mass ratio of the particle.

Explanation

To find the charge-to-mass ratio (Q/m) of a particle moving in a circular path under the influence of a magnetic field and an electric field, we can follow these steps:

1. Identify Given Values:
- Diameter of the circular path, \( d = 1.0 \, \text{cm} = 0.01 \, \text{m} \)
- Radius of the circular path, \( R = \frac{d}{2} = \frac{0.01}{2} = 0.005 \, \text{m} \)
- Magnetic field strength, \( B = 0.40 \, \text{T} \)
- Electric field strength, \( E = 200 \, \text{V/m} \)

2. Determine the Speed of the Particle:
- When the electric field is present, the particle moves in a straight line, which means the electric force is balanced by the magnetic force.
- The relationship between the electric field (E), magnetic field (B), and speed (v) of the particle is given by:
\( v = \frac{E}{B} \)
- Substituting the values:
\( v = \frac{200 \, \text{V/m}}{0.40 \, \text{T}} = 500 \, \text{m/s} \)

3. Use the Circular Motion Equation:
- The radius of the circular path in a magnetic field is given by:
\( R = \frac{mv}{qB} \)
- Rearranging this equation to find the charge-to-mass ratio \( \frac{q}{m} \):
\( \frac{q}{m} = \frac{v}{RB} \)

4. Substituting the Known Values:
- Substitute \( v = 500 \, \text{m/s} \), \( R = 0.005 \, \text{m} \), and \( B = 0.40 \, \text{T} \):
\( \frac{q}{m} = \frac{500}{0.005 \times 0.40} \)

5. Calculating the Charge-to-Mass Ratio:
- Calculate the denominator:
\( 0.005 \times 0.40 = 0.002 \)
- Now, calculate \( \frac{q}{m} \):
\( \frac{q}{m} = \frac{500}{0.002} = 250000 \, \text{C/kg} \)

6. Final Result:
- Therefore, the charge-to-mass ratio of the particle is:
\( \frac{q}{m} = 2.5 \times 10^5 \, \text{C/kg} \)