A 0.5m long straight wire in which a current of 1.2A is flowing is kept a right angles to a uniform magnetic field of 2.0 tesla. The force acting on the wire will be-
2N
2.4N
1.2N
3N
The correct Answer is:C
To find the force acting on a straight wire carrying a current in a magnetic field, we can use the formula:
F=I⋅L⋅B⋅sin(θ)
Where:
- F is the force acting on the wire (in Newtons),
- I is the current flowing through the wire (in Amperes),
- L is the length of the wire (in meters),
- B is the magnetic field strength (in Teslas),
- θ is the angle between the direction of the current and the magnetic field.
1. Identify the given values:
- Length of the wire, L=0.5m
- Current, I=1.2A
- Magnetic field strength, B=2.0T
- Angle θ=90∘ (since the wire is at right angles to the magnetic field)
2. Substitute the values into the formula:
F=I⋅L⋅B⋅sin(θ)
F=1.2A⋅0.5m⋅2.0T⋅sin(90∘)
3. Calculate sin(90∘):
sin(90∘)=1
4. Plug in the value of sin(90∘):
F=1.2⋅0.5⋅2.0⋅1
5. Perform the multiplication:
- First, calculate 1.2⋅0.5=0.6
- Then, calculate 0.6⋅2.0=1.2
6. Final result:
F=1.2N
Thus, the force acting on the wire is 1.2 Newtons.
- F is the force acting on the wire (in Newtons),
- I is the current flowing through the wire (in Amperes),
- L is the length of the wire (in meters),
- B is the magnetic field strength (in Teslas),
- θ is the angle between the direction of the current and the magnetic field.
- Length of the wire, L=0.5m
- Current, I=1.2A
- Magnetic field strength, B=2.0T
- Angle θ=90∘ (since the wire is at right angles to the magnetic field)
F=I⋅L⋅B⋅sin(θ)
F=1.2A⋅0.5m⋅2.0T⋅sin(90∘)
sin(90∘)=1
F=1.2⋅0.5⋅2.0⋅1
- First, calculate 1.2⋅0.5=0.6
- Then, calculate 0.6⋅2.0=1.2
F=1.2N
Topper's Solved these Questions
Similar Questions
A wire carrying a current i is placed in a uniform magnetic field in the form of the curve y=asin(πxL)0≤x≤2L. The force acting on the wire is
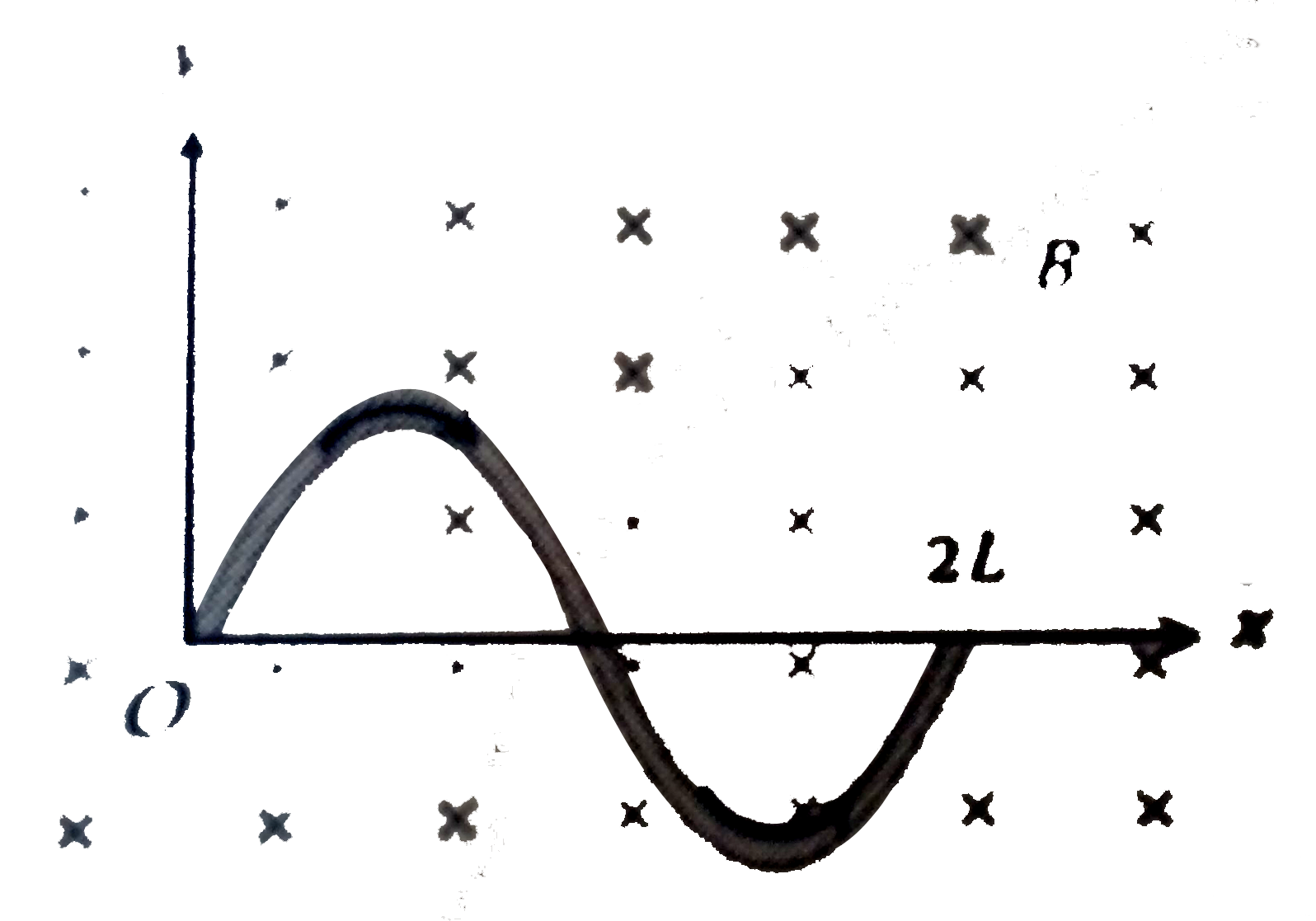
View SolutionA unifrom conducting wire ABC has a mass of 10g. A current of 2A flows through it. The wire is kept in a unifrom magnetic field B=2T. The accleration of the wire will be

View SolutionA current of 1 A flows a wire of length 0.1 m in a magnetic field of 0.5 T. The force acting on the wire when it makes an angle of 90∘ with the field is
View Solution1.2 amp current is flowing in a wire of 0.3 m length, It is placed perpendicular to the magnetic field (identical to 2T). The force acting on the wire will be:
View SolutionA current of 2 amp. flows in a long, straight wire of radius 2mm. The intensity of magnetic field on the axis of the wire is
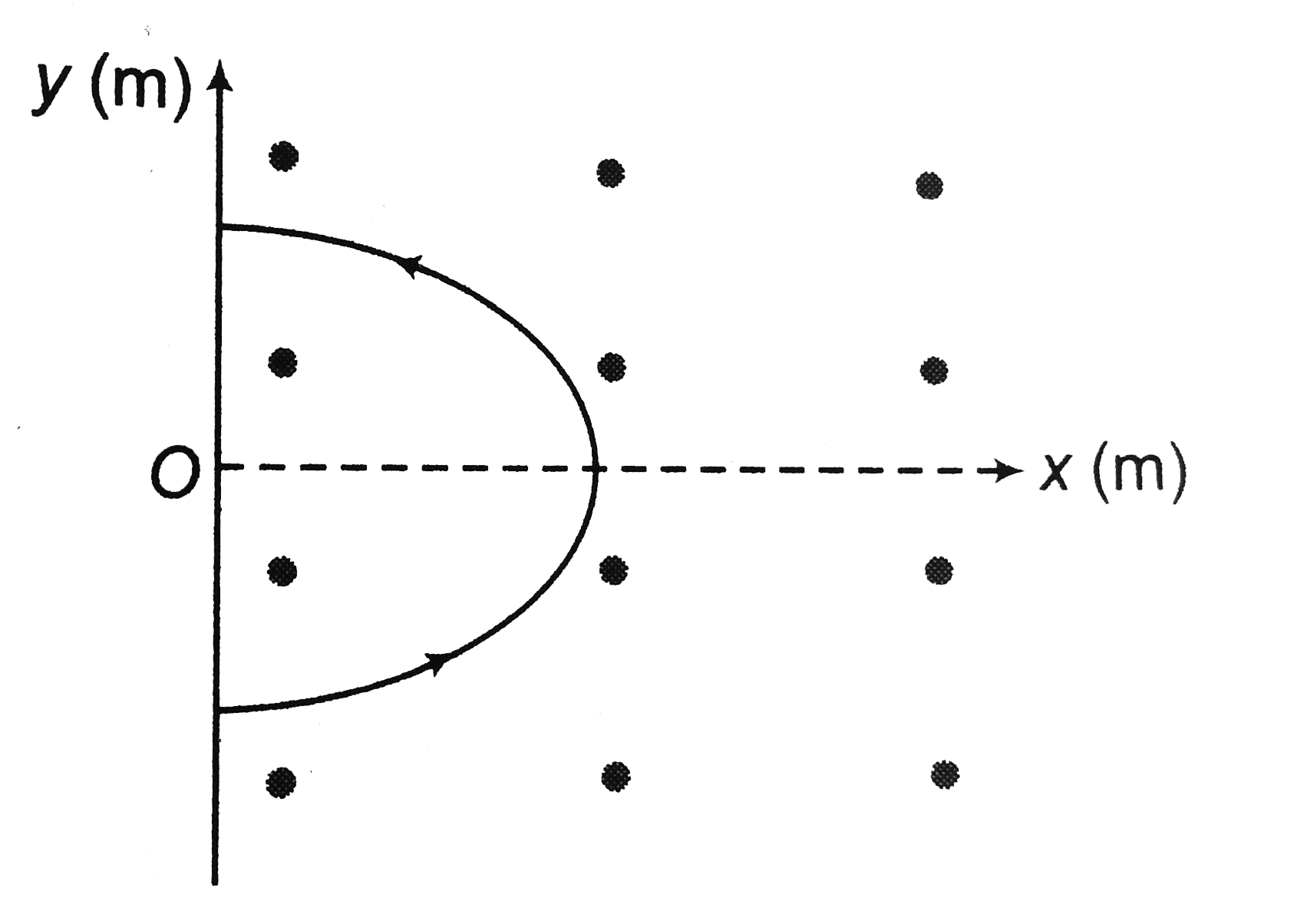
View SolutionA wire carrying a current of 3A is bent in the form of a parabola y2=4−x as shown in figure, where x and y are in metre. The wire is placed in a uniform magnetic field B=5ˆk tesla. The force acting on the wire is
View SolutionA long straight wire carrying current of 30A is placed in an external uniform magnetic field of induction 4×10−4T. The magnetic field is acting parallel to the direction of current. The magnitude of the resultant magnetic induction in tesla at a point 2.0cm away from the wire is
View SolutionA wire bent in the form a right angled triangle PQR, carries a current 1A. It is placed in a region of a uniform magnetic field B=0.2T. If PR=1m, the net force on the wire is

View SolutionA long straight wire carrying current of 30A is placed in an external unifrom magnetic field of induction 4×104T. The magnetic field is acting parallel to the direction of current. The maggnetic of the resultant magnetic inuduction in tesla at a point 2.0cm away form the wire is
View SolutionA straight wire of length 0.5 metre and carrying a current of 1.2 ampere is placed in a uniform magnetic field of induction 2 tesla. If the magnetic field is perpendicular to the length of the wire , the force acting on the wire is
View Solution
