A conductor in the form of a right angle ABC with AB=3cm and BC=4cm carries a current of 10A.There is a uniform magnetic field of 5T perpendicular to the palne of the conductor. The force on the conductor will be
1.5N
2.0N
2.5N
3.5N
The correct Answer is:C
To find the force on the conductor in the form of a right angle ABC, we can follow these steps:
Step 1: Identify the Given Data
- Length of AB = 3 cm = 0.03 m (convert to meters)
- Length of BC = 4 cm = 0.04 m (convert to meters)
- Current, I = 10 A
- Magnetic field, B = 5 T
Step 2: Calculate the Force on Each Segment of the Conductor
The force on a straight conductor carrying current in a magnetic field can be calculated using the formula:
\( F = B \cdot I \cdot L \)
where:
- \( F \) = force on the conductor
- \( B \) = magnetic field strength
- \( I \) = current
- \( L \) = length of the conductor segment
Force on Segment AB
- Length of AB = 0.03 m
- Using the formula:
\( F_{AB} = B \cdot I \cdot L_{AB} = 5 \, \text{T} \cdot 10 \, \text{A} \cdot 0.03 \, \text{m} \)
\( F_{AB} = 5 \cdot 10 \cdot 0.03 = 1.5 \, \text{N} \)
Force on Segment BC
- Length of BC = 0.04 m
- Using the formula:
\( F_{BC} = B \cdot I \cdot L_{BC} = 5 \, \text{T} \cdot 10 \, \text{A} \cdot 0.04 \, \text{m} \)
\( F_{BC} = 5 \cdot 10 \cdot 0.04 = 2.0 \, \text{N} \)
Step 3: Determine the Direction of Forces
- The force on segment AB will be directed out of the plane of the paper (using the right-hand rule).
- The force on segment BC will be directed into the plane of the paper.
Step 4: Calculate the Net Force
Since the forces on segments AB and BC are in opposite directions, we can find the net force by subtracting the smaller force from the larger force:
\( F_{net} = F_{BC} - F_{AB} = 2.0 \, \text{N} - 1.5 \, \text{N} = 0.5 \, \text{N} \)
Conclusion
The total force acting on the conductor is \( 0.5 \, \text{N} \).
- Length of AB = 3 cm = 0.03 m (convert to meters)
- Length of BC = 4 cm = 0.04 m (convert to meters)
- Current, I = 10 A
- Magnetic field, B = 5 T
The force on a straight conductor carrying current in a magnetic field can be calculated using the formula:
\( F = B \cdot I \cdot L \)
where:
- \( F \) = force on the conductor
- \( B \) = magnetic field strength
- \( I \) = current
- \( L \) = length of the conductor segment
- Length of AB = 0.03 m
- Using the formula:
\( F_{AB} = B \cdot I \cdot L_{AB} = 5 \, \text{T} \cdot 10 \, \text{A} \cdot 0.03 \, \text{m} \)
\( F_{AB} = 5 \cdot 10 \cdot 0.03 = 1.5 \, \text{N} \)
- Length of BC = 0.04 m
- Using the formula:
\( F_{BC} = B \cdot I \cdot L_{BC} = 5 \, \text{T} \cdot 10 \, \text{A} \cdot 0.04 \, \text{m} \)
\( F_{BC} = 5 \cdot 10 \cdot 0.04 = 2.0 \, \text{N} \)
- The force on segment AB will be directed out of the plane of the paper (using the right-hand rule).
- The force on segment BC will be directed into the plane of the paper.
Since the forces on segments AB and BC are in opposite directions, we can find the net force by subtracting the smaller force from the larger force:
\( F_{net} = F_{BC} - F_{AB} = 2.0 \, \text{N} - 1.5 \, \text{N} = 0.5 \, \text{N} \)
The total force acting on the conductor is \( 0.5 \, \text{N} \).
Topper's Solved these Questions
Similar Questions
A straight conductor of length 32 cm carries a current of 30A. Magnetic induction at a point which is in air at a perpendicular distance of 12cm from the mid point of the conductor is
View Solutiona. A conductor in the shape of a square of edge length l=0.4m carries a current i=10.0A. Calculate the magnitude and direction of magnetic field at the centre of the square.
b. If this conductor is formed into a single circular turn and carries the same current. what is the value of the magnetic field at the centre.

View Solution
Knowledge Check
An 8 cm long wire carrying a current of 10 A is placed inside a solenoid perpendicular to its axis. If the magnetic field inside the solenoid is 0.3 T, then magnetic force on the wire is
A0.14NB0.24NC0.34ND0.44N
ΔABC is right-angled at C. If AC=5cmandBC=12cm find the length of AB.
View SolutionFind magnetic field at O due to the current carrying conductor as shown in the figure.
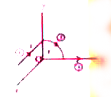
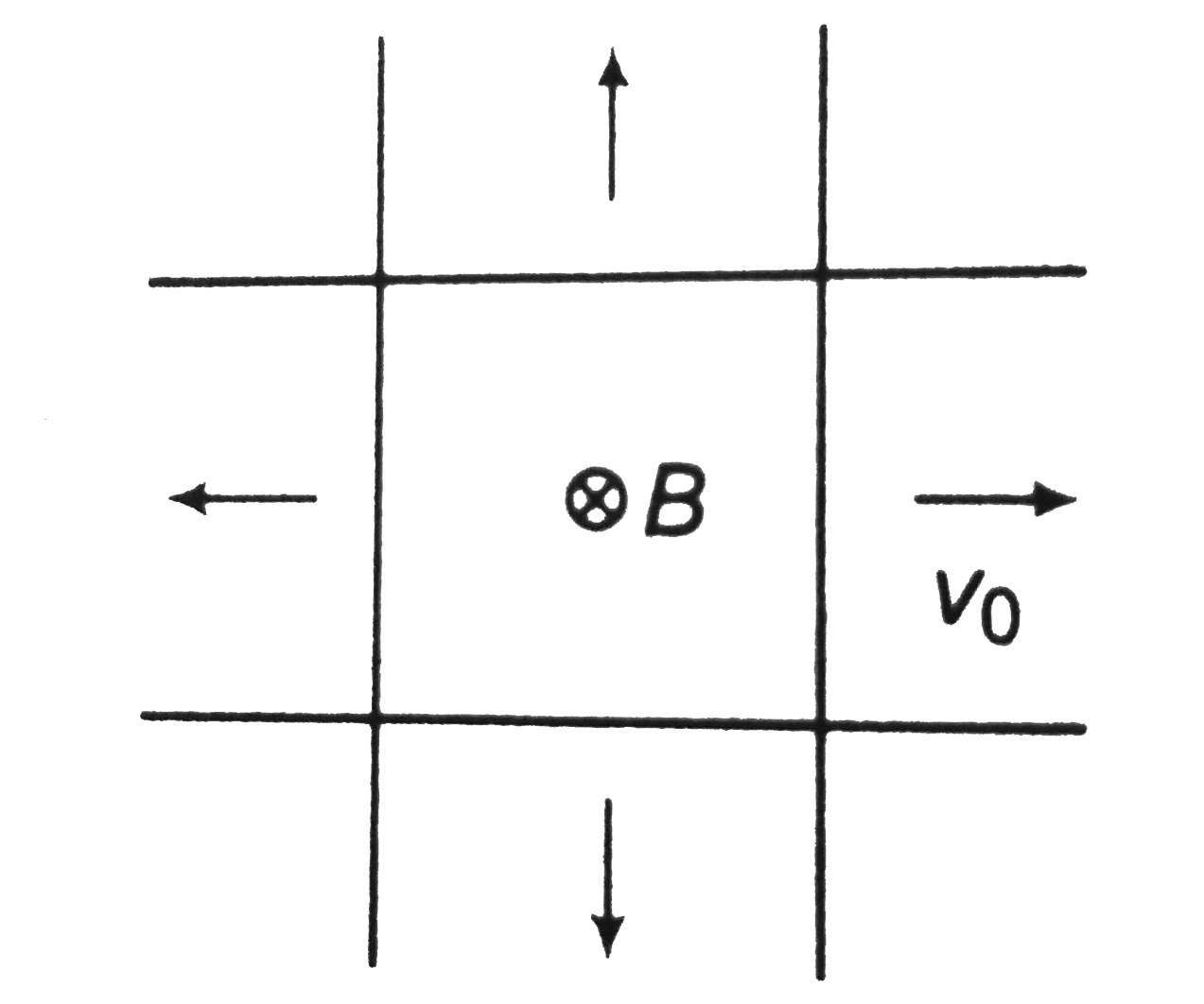
View SolutionTwo parallel long straight conductors lie on a smooth plane surface. Two other parallel conductors rest on them at right angle so as to form a square of side a. A uniform magnetic field B exists at right angle to the plane containing the conductors. Now, conductors starts moving outward witha constant velocity v0 at t=0. Then, induced current in the loop at any time is (λ is resistance per unit length of the conductors)
View SolutionConstruct a right angled ΔABC in which AB=4.2cm,BC=4.5cmand∠B=90∘.
View Solution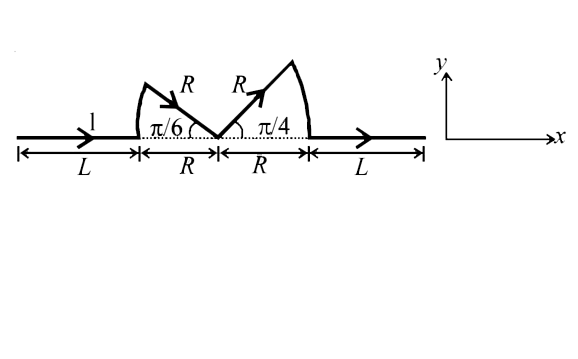
A conductor (shown in the figure) carrying constant current I is kept in the x-y plane in a uniform magnetic field B. If F is the magnitude of the total magnetic force acting on the conductor, then the correct statement is/are
View SolutionA conductor (shown in the figure) carrying constant current I is kept in the x−y plane in a uniform magnetic field →B. If →F is the magnitude of the total magnetic force acting on the conductor, then the correct statement(s) is (are)

View SolutionConstruct a right triangle ABC in which ∠B=90∘,BC=3.6cmandCA=5.4cm,
View Solution
