A fresh dry cell of 1.5 volt and two resistors of 10kΩ each are connected in series. An analog voltmeter measures a voltage of 0.5 volt across each of the resistor. A 100μF capacitor is fully charged using the same source. The same voltmeter is now used to measure the voltage across It. The initial value of the current and the time in which the voltmeter reading falls to 0.5 volt are respectively.
60μA,11s
120μA,15s
150μA,m15s
150μA,11s
The correct Answer is:D
To solve the problem step by step, we will analyze the circuit and use the relevant formulas.
Step 1: Understand the Circuit Configuration
We have a fresh dry cell of 1.5 volts connected in series with two resistors, each of 10 kΩ. The total resistance in the circuit is:
Rtotal=R1+R2=10kΩ+10kΩ=20kΩ
Step 2: Calculate the Total Current in the Circuit
Using Ohm's law, the total current I in the circuit can be calculated as:
I=VRtotal=1.5V20kΩ
Converting 20kΩ to ohms:
20kΩ=20,000Ω
Now substituting the values:
I=1.520000=0.000075A=75μA
Step 3: Analyze the Voltmeter Reading
The voltmeter measures 0.5 volts across each resistor. This indicates that the voltmeter is also part of the circuit and has some internal resistance. The reading across each resistor confirms that the circuit is functioning correctly.
Step 4: Charging the Capacitor
A capacitor of 100μF is charged using the same source. When the capacitor is fully charged, it will have the same voltage as the source, which is 1.5 volts.
Step 5: Determine the Initial Current through the Capacitor
When the capacitor is first connected, it behaves like a short circuit, and the initial current I0 can be calculated using:
I0=VR
Where R is the total resistance in the circuit, which is still 20kΩ:
I0=1.5V20kΩ=75μA
Step 6: Calculate the Time for the Voltage to Fall to 0.5 Volts
The voltage across a charging capacitor can be described by the equation:
V(t)=V0(1−e−tRC)
Where:
- V0=1.5V (initial voltage)
- V(t)=0.5V (voltage after time t)
- R=20kΩ=20000Ω
- C=100μF=100×10−6F
First, calculate RC:
RC=20000Ω×100×10−6F=2seconds
Now, substituting into the voltage equation:
0.5=1.5(1−e−t2)
Rearranging gives:
0.51.5=1−e−t2
13=1−e−t2
e−t2=1−13=23
Taking the natural logarithm:
−t2=ln(23)
t=−2ln(23)
Calculating t:
t≈−2×(−0.4055)≈0.811seconds
Final Results
The initial current is 75μA and the time for the voltmeter reading to fall to 0.5 volts is approximately 0.811seconds.
We have a fresh dry cell of 1.5 volts connected in series with two resistors, each of 10 kΩ. The total resistance in the circuit is:
Rtotal=R1+R2=10kΩ+10kΩ=20kΩ
Using Ohm's law, the total current I in the circuit can be calculated as:
I=VRtotal=1.5V20kΩ
Converting 20kΩ to ohms:
20kΩ=20,000Ω
Now substituting the values:
I=1.520000=0.000075A=75μA
The voltmeter measures 0.5 volts across each resistor. This indicates that the voltmeter is also part of the circuit and has some internal resistance. The reading across each resistor confirms that the circuit is functioning correctly.
A capacitor of 100μF is charged using the same source. When the capacitor is fully charged, it will have the same voltage as the source, which is 1.5 volts.
When the capacitor is first connected, it behaves like a short circuit, and the initial current I0 can be calculated using:
I0=VR
Where R is the total resistance in the circuit, which is still 20kΩ:
I0=1.5V20kΩ=75μA
The voltage across a charging capacitor can be described by the equation:
V(t)=V0(1−e−tRC)
Where:
- V0=1.5V (initial voltage)
- V(t)=0.5V (voltage after time t)
- R=20kΩ=20000Ω
- C=100μF=100×10−6F
RC=20000Ω×100×10−6F=2seconds
0.5=1.5(1−e−t2)
Rearranging gives:
0.51.5=1−e−t2
13=1−e−t2
e−t2=1−13=23
−t2=ln(23)
t=−2ln(23)
t≈−2×(−0.4055)≈0.811seconds
The initial current is 75μA and the time for the voltmeter reading to fall to 0.5 volts is approximately 0.811seconds.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.57
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.58
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.55
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
Two resistor 400Ω and 800Ω connected in series across a 6 V battery . Reading of Voltmeter of 10kΩ across 400Ω resistor is close to :
View SolutionRedraw the circuit of Q.12, putting in an ammeter to measure the current through the resistors and a voltmeter to measure the voltage across the 12ohm resistor. What would be the readings in the ammeter and the voltmeter ?
View SolutionVoltmeter V and Ammeter A are used to measure the total potential difference of the circuit and the current through each resistor. Which is the correct circuit ?
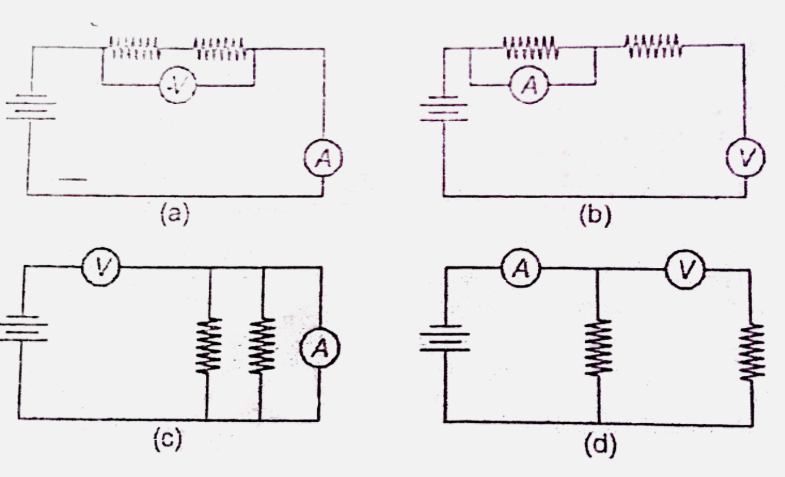
View SolutionTwo resistors of 4Ωand6Ω are connected in parallel to a cell to draw 0.5 A current from the cell.
Calculate the current in each resistor.
View SolutionA : parctically a voltmeter will measure the voltage across the battery but not its EMF.
R : EMF of a cell is measured with the help of a potentiometer .
View SolutionA voltmeter with resistance 500Ω is used to measure the emf of a cell of internal resistance 4Ω. The percentage error in the reading of the voltmeter will be
View SolutionThe resistance of galvanometer is g ohm and the range is 1 volt. The value of resistance used to convert it into a voltmeter of ragne 10 volt is:-
View SolutionWhen a battery connected across a resistor of 16Ω, the voltage across the resistor is 12 V. When the same battery is connected across a resistor of 10Ω, voltage across it is 11 V. The internal resistance of the battery (in ohm) is
View SolutionFor the circuit shown in figure, determine the voltage across 4Ω resistors in volt ?
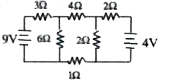
View SolutionTwo resistances of 100 ohm and 200 ohm are connected in series with a battery of emf 4 volt and negligible internal resistance. A voltmeter of resistance 200 ohm is used to measure voltage across the two resistances separately. Calculate the voltage indicated.
View Solution
