An elevator of height 'h' ascends with constant acceleration 'a'. When it corsses a plalform bolt drops from the top of the elevator. If the time for the bolt to hit the floor of the elevator is √λhg+a then find 'λ'.
The correct Answer is:2
To solve the problem, we need to analyze the motion of the bolt dropped from the elevator. The elevator is ascending with a constant acceleration 'a', and we need to find the time it takes for the bolt to hit the floor of the elevator.
1. Identify the Situation:
- The elevator has a height 'h' and is moving upwards with an acceleration 'a'.
- The bolt is dropped from the top of the elevator when it crosses a platform.
2. Determine the Forces Acting on the Bolt:
- Once the bolt is dropped, it is only influenced by gravity. The acceleration due to gravity is 'g' acting downwards.
- The elevator continues to accelerate upwards with 'a'.
3. Calculate the Relative Acceleration:
- The relative acceleration of the bolt with respect to the elevator can be calculated as:
arelative=g+a
- Here, 'g' is positive (downward) and 'a' is also considered positive (upward), hence the total effective acceleration acting on the bolt relative to the elevator is g+a.
4. Use the Equation of Motion:
- The distance the bolt falls relative to the elevator is 'h'. Using the second equation of motion:
h=12arelativet2
- Substituting the relative acceleration:
h=12(g+a)t2
5. Rearranging the Equation:
- Rearranging the equation to solve for time 't':
t2=2hg+a
- Taking the square root gives:
t=√2hg+a
6. Comparing with Given Time Expression:
- The problem states that the time 't' is also given by:
t=√λhg+a
- Setting the two expressions for 't' equal to each other:
√2hg+a=√λhg+a
7. Squaring Both Sides:
- Squaring both sides to eliminate the square root:
2hg+a=λhg+a
8. Canceling Common Terms:
- Since h and g+a are positive and non-zero, we can cancel them:
2=λ
9. Final Result:
- Therefore, the value of λ is:
λ=2
Conclusion:
The value of λ is 2.
---
- The elevator has a height 'h' and is moving upwards with an acceleration 'a'.
- The bolt is dropped from the top of the elevator when it crosses a platform.
- Once the bolt is dropped, it is only influenced by gravity. The acceleration due to gravity is 'g' acting downwards.
- The elevator continues to accelerate upwards with 'a'.
- The relative acceleration of the bolt with respect to the elevator can be calculated as:
arelative=g+a
- Here, 'g' is positive (downward) and 'a' is also considered positive (upward), hence the total effective acceleration acting on the bolt relative to the elevator is g+a.
- The distance the bolt falls relative to the elevator is 'h'. Using the second equation of motion:
h=12arelativet2
- Substituting the relative acceleration:
h=12(g+a)t2
- Rearranging the equation to solve for time 't':
t2=2hg+a
- Taking the square root gives:
t=√2hg+a
- The problem states that the time 't' is also given by:
t=√λhg+a
- Setting the two expressions for 't' equal to each other:
√2hg+a=√λhg+a
- Squaring both sides to eliminate the square root:
2hg+a=λhg+a
- Since h and g+a are positive and non-zero, we can cancel them:
2=λ
- Therefore, the value of λ is:
λ=2
The value of λ is 2.
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PHYSICS
Explore 784 Videos
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PHYSICS
Explore 784 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
An elevator car whose floor to ceiling distance is equal to 2.7m starts ascending with constant acceleration 1.2m/s2. 2 s after the start, a bolt begins falling from the ceiling of the car. Find
(a)the time after which bolt hits the floor of the elevator.
(b)the net displacement and distance travelled by the bolt, with respect to earth. (Take g=9.8m/s2)
View SolutionAn elevator (lift) ascends with an upward acceleration of 1.2ms−2. At the instant when its upward speed is 2.4ms−1, a loose bolt drops from the ceiling of the elevator 2.7m above the floor of the elevator. Calculate (a) the time of flight of the bolt from the ceiling to the floor and (b) the distance it has fallen relaative to the elevator shaft.
View SolutionAn elevator ascends an upward acceleration of 0.2 m/s2. At the instant it upwards speed in 3m/sec a loose bolt 5 m high form the floor drops from the ceiling of the elevator.Find the time until the bolt strikes the floor and the displacement it has fallen .
View SolutionAn elevator is descending with uniform acceleration.To measure the acceleration, a person in the elevator drops a coin at momen the elevator strts. The coin is 6 ft asbove the floor of the elevator at the time it is dropped. The person observes that the coin strikes the floor in 1 second. Calculate these dta the acceleration of the elevator.
View SolutionAn elevator whose floor-to-ceiling destance is 2.50m starts ascending with a constant acceleration of 1.25ms−2 On second after the start, a bolt begins falling from the elevator. Calculate the free fall time of the bolt
View SolutionAn elevator without a ceiling is ascending with a constant speed of 10 m//s. A boy on the elevator shoots a ball directly upward, from a height of 2.0 m above the elevator floor. At this time the elevator floor is 28 m above the ground. The initial speed of the ball with respect to the elevator is 20 m//s. (Take g=9.8m/s2)
(a) What maximum height above the ground does the ball reach?
(b) How long does the ball take to return to the elevator floor?
View SolutionA lift, initially at rest on the ground, starts ascending with constant acceleration 8m/s2 After 0.5 seconds, a bolt falls off the floor of the lift. The velocity of the lift at the instant the bolt hits the ground is m/s .[Take g=10m/s2]
View SolutionThe angle of elevation of the sun when the length of the shadow of a pole is √3 times the height of the pole is
View SolutionAn elevator without a ceiling is ascending up with an acceleration of 5ms−2. A boy on the elevator shoots a ball in vertical upward direction from a height of 2 m above the floor of elevator. At this instant the elevator is moving up with a velocity of 10ms−1 and floor of the elevator is at a height of 50 m from the ground. The initial speed of the ball is 15ms−1 with respect to the elevator. Consider the duration for which the ball strikes the floor of elevator in answering following questions. (g=10ms−2)
1. The time in which the ball strikes the floor of elevator is given by
View Solution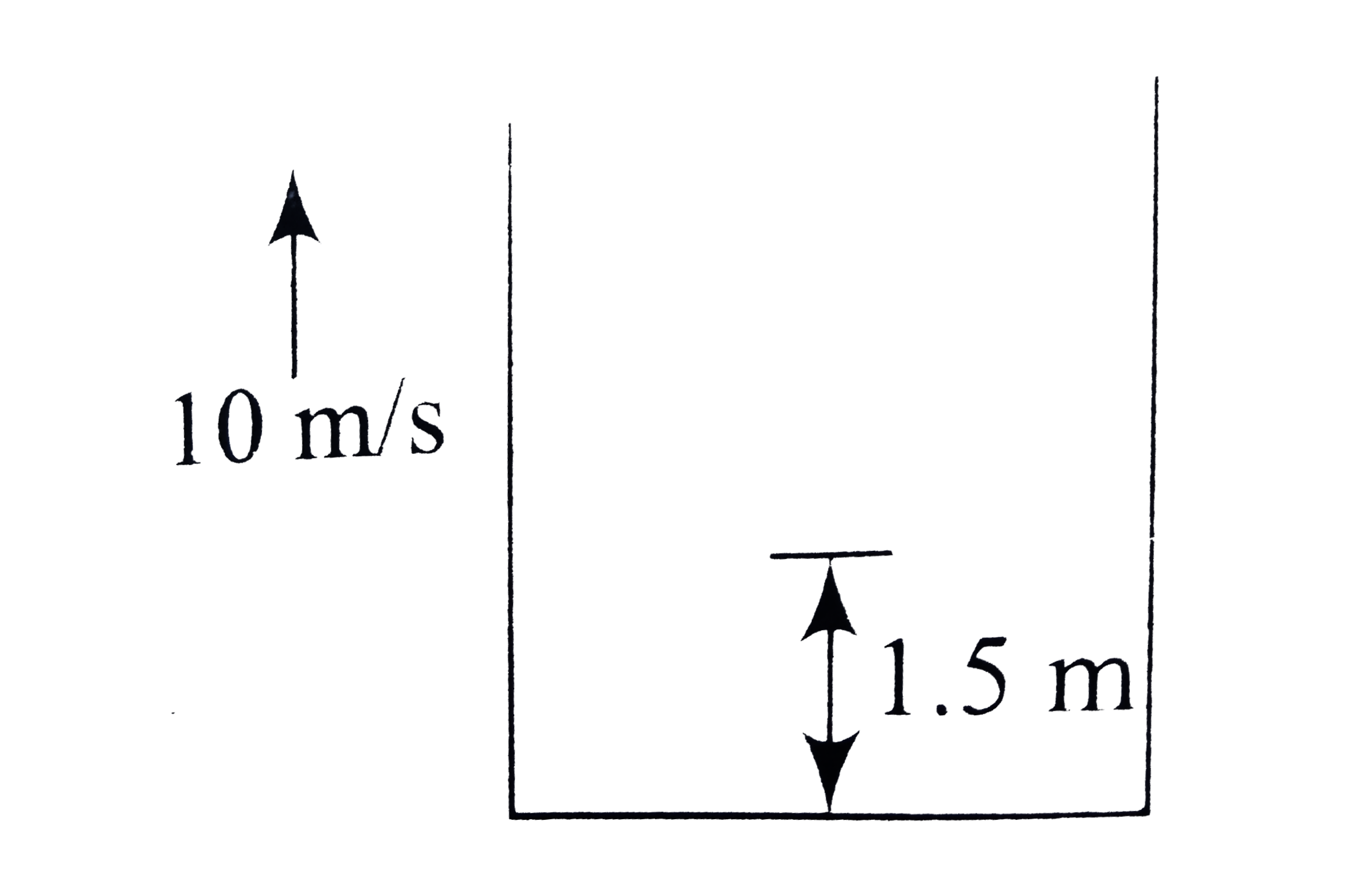
A boy in the elevator shoots a bullet in a vertical upward direction from a height of 1.5 m above the floor of the elevator. There is no roof in the elevator. The floor of the elevator is at 50 m from ground at the instant when velocity of the elevator is 10 m/s in upward direction. The bullet strikes the floor of the elevator in 2 seconds. The initial speed of the bullet is 15 m/s relative to the elevator.
Q. Find the acceleration of the elevator in upward direction, (mss2):
View Solution

