Question

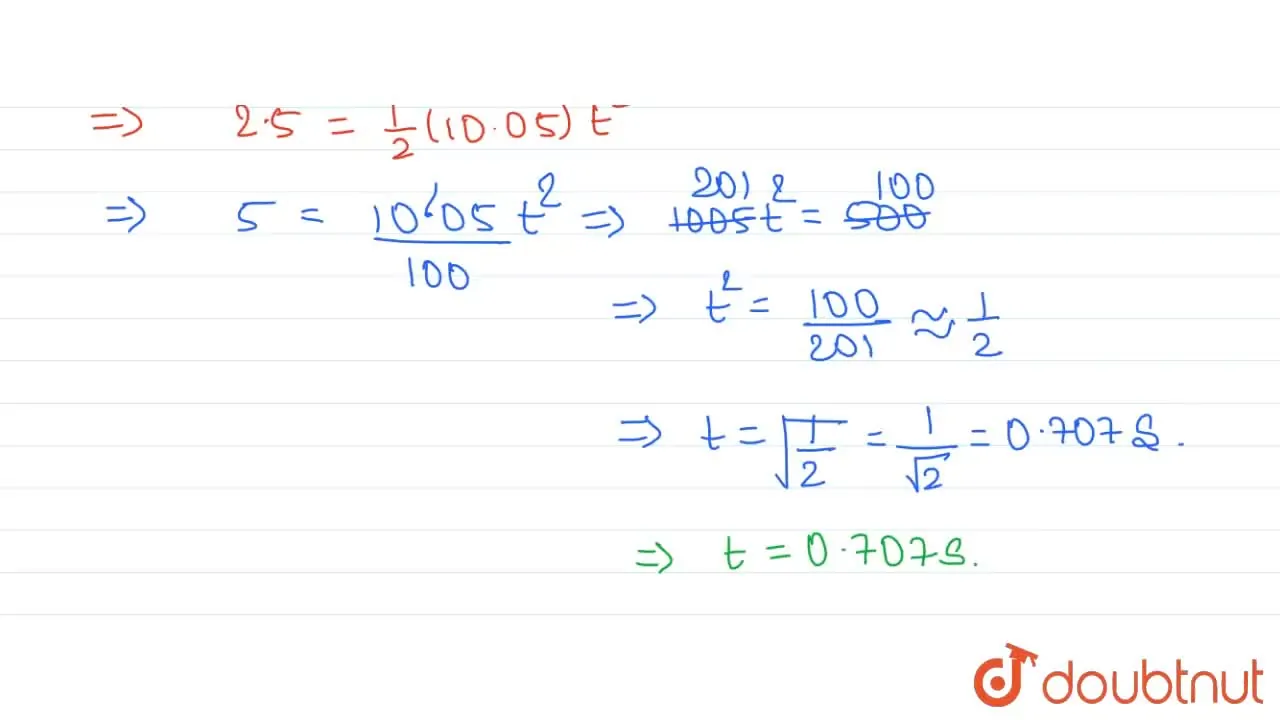
An elevator whose floor-to-ceiling destance is ${\displaystyle 2.50m}$ starts ascending with a constant acceleration of ${\displaystyle 1.25m{s}^{-2}}$ On second after the start, a bolt begins falling from the elevator. Calculate the free fall time of the bolt

Answer

`(2)/(3)`;
`(25)/(18)m; (445)/(288) m`

Explanation

To solve the problem step-by-step, we will analyze the situation of the bolt falling from the elevator and apply the principles of kinematics.

Step 1: Understand the scenario
The elevator is ascending with a constant acceleration of \( a = 1.25 \, \text{m/s}^2 \). The distance from the ceiling to the floor of the elevator is \( s = 2.50 \, \text{m} \). The bolt starts falling from the ceiling of the elevator one second after the elevator starts moving.

Step 2: Determine the effective acceleration of the bolt
When the bolt begins to fall, it is subjected to two forces:
1. The gravitational force acting downward, \( mg \), where \( g \approx 9.8 \, \text{m/s}^2 \).
2. A pseudo force acting upward due to the elevator's acceleration, which is \( ma \), where \( a = 1.25 \, \text{m/s}^2 \).

The net acceleration of the bolt in the downward direction can be calculated as:
\( a_{\text{net}} = g + a = 9.8 \, \text{m/s}^2 + 1.25 \, \text{m/s}^2 = 11.05 \, \text{m/s}^2 \)

Step 3: Set up the kinematic equation
We will use the kinematic equation for uniformly accelerated motion:
\( s = ut + \frac{1}{2} a t^2 \)
Where:
- \( s = 2.50 \, \text{m} \) (the distance the bolt falls)
- \( u = 0 \, \text{m/s} \) (initial velocity of the bolt when it starts falling)
- \( a = 11.05 \, \text{m/s}^2 \) (the effective acceleration)
- \( t \) is the time we want to find.

Substituting the known values into the equation:
\( 2.50 = 0 \cdot t + \frac{1}{2} \cdot 11.05 \cdot t^2 \)
This simplifies to:
\( 2.50 = \frac{11.05}{2} t^2 \)
\( 2.50 = 5.525 t^2 \)

Step 4: Solve for \( t^2 \)
Rearranging the equation gives:
\( t^2 = \frac{2.50}{5.525} \)
Calculating the right side:
\( t^2 \approx 0.452 \)

Step 5: Calculate \( t \)
Taking the square root of both sides:
\( t \approx \sqrt{0.452} \approx 0.672 \, \text{s} \)

Conclusion
The time taken by the bolt to fall from the ceiling to the floor of the elevator is approximately \( 0.672 \, \text{s} \).