The distance travelled by a particle is proportional to the square of time, then the particle travels with
the velocity is constant
the velocity is variable
the acceleration is constant
the acceleration is variable
The correct Answer is:B, C
s∝t2
∴s=ct where c=constant
(i)v=dsdt=2ct
∴V∝t
(ii)a=dvdt=2c
so, a=constant
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PHYSICS
Explore 784 Videos
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PHYSICS
Explore 784 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
The distance travelled by a particle is proportional to the squares of time, then the particle travels with
View SolutionThe displacement of a particle starts from rest is proportional to the square of time, then the particle travels with
View SolutionThe distance travelled by a freely falling body is proportional to
View SolutionIn a straight line motion the distance travelled is proportional to the square root of the time taken The acceleration of the particle is proportional to :-
View SolutionA particle moves in one dimension from rest under the influence of a force that varies with the distance travelled by the particle as shown in the figure. The kinetic energy of the particle after it has travelled 3m is:
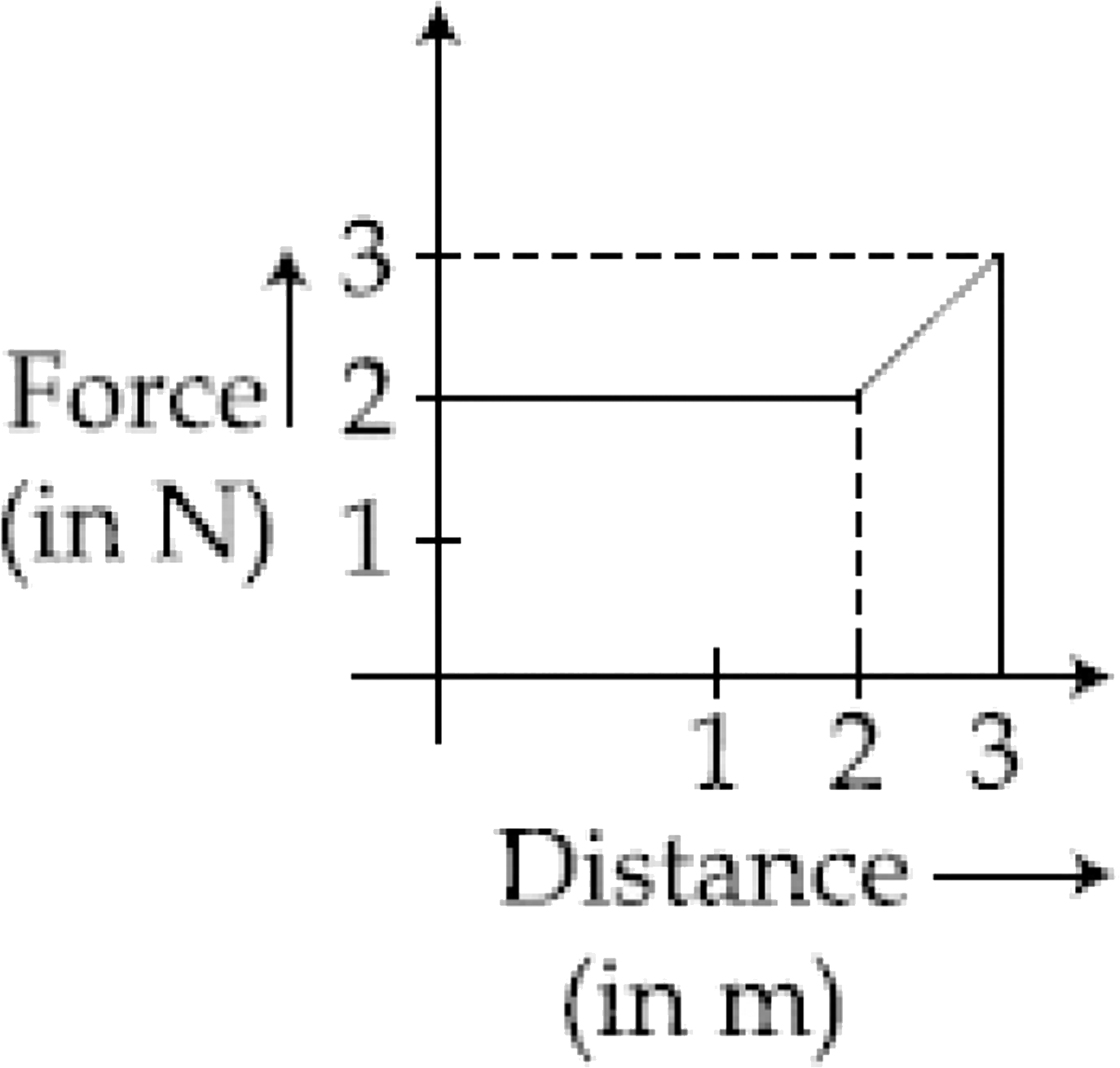
View SolutionName
Distance travelled in unit time
View SolutionA particle of mass m initially moving with speed v.A force acts on the particle f=kx where x is the distance travelled by the particle and k is constant. Find the speed of the particle when the work done by the force equals W.
View SolutionA particle is projected with velocity v0 along x - axis. The deceleration on the particle is proportional to the square of the distance from the origin i.e. a=−x2. The distance at which particle stops is -
View SolutionThe distance travelled by a particle starting from rest and moving with an acceleration 3ms−2, in the 5th second is.
View SolutionIf a particle is moving such that the velocity acquired is proportional to the square root of the distance covered, then its acceleration is
View Solution