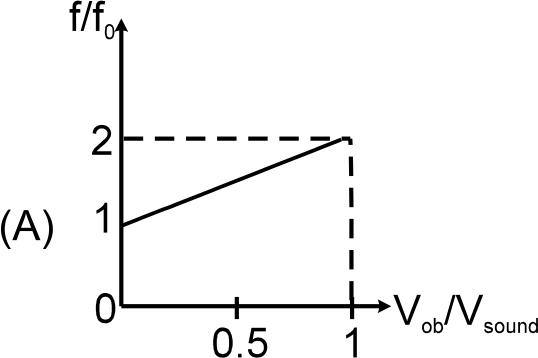
A curve is plotted to represenet the dependence of the ratio of the received frequency f to the frequency f0 emitted by the source on the ratio of the speed of obsever Vob to the speed of sound Vsound in a situation in which an observer is moving towards a stationary sound source. The curve is best represnted by :
To solve the problem, we need to analyze the relationship between the received frequency f and the emitted frequency f0 when an observer is moving towards a stationary sound source. We will derive the equation and identify the correct curve representation.
1. Understanding the Doppler Effect:
When an observer moves towards a stationary sound source, the frequency of the sound received by the observer is higher than the frequency emitted by the source. This phenomenon is described by the Doppler effect.
2. Doppler Effect Formula:
The formula for the frequency received by the observer when moving towards a stationary source is given by:
f=f0(Vsound+VobVsound)
Here, Vob is the speed of the observer, and Vsound is the speed of sound.
3. Expressing the Ratio:
We can express the ratio of the received frequency to the emitted frequency:
ff0=Vsound+VobVsound
4. Simplifying the Expression:
This can be simplified to:
ff0=1+VobVsound
Let x=VobVsound. Therefore, we can rewrite the equation as:
ff0=1+x
5. Identifying the Curve:
The equation ff0=1+x indicates a linear relationship between ff0 (y-axis) and VobVsound (x-axis). The slope of this line is 1 and the y-intercept is also 1.
6. Conclusion:
The best representation of the curve based on the derived equation is a straight line with a slope of 1 and a y-intercept of 1. Therefore, the correct option is the one that represents this linear relationship.
When an observer moves towards a stationary sound source, the frequency of the sound received by the observer is higher than the frequency emitted by the source. This phenomenon is described by the Doppler effect.
The formula for the frequency received by the observer when moving towards a stationary source is given by:
f=f0(Vsound+VobVsound)
Here, Vob is the speed of the observer, and Vsound is the speed of sound.
We can express the ratio of the received frequency to the emitted frequency:
ff0=Vsound+VobVsound
This can be simplified to:
ff0=1+VobVsound
Let x=VobVsound. Therefore, we can rewrite the equation as:
ff0=1+x
The equation ff0=1+x indicates a linear relationship between ff0 (y-axis) and VobVsound (x-axis). The slope of this line is 1 and the y-intercept is also 1.
The best representation of the curve based on the derived equation is a straight line with a slope of 1 and a y-intercept of 1. Therefore, the correct option is the one that represents this linear relationship.
Topper's Solved these Questions
Similar Questions
When source of sound moves towards a stationary observer, the wavelength of sound received by him
View Solutionif the speed of a sound source is equal to the speed fo sound and the source is moving away from the observer, then the ratio of apparent frequency to the original frequency is
View Solution
Knowledge Check
An observer moves towards a stationary source of sound. The percentage change in the apparent frequency is
AzeroB0.05C0.1D0.2To a stationary man the frequency of a sound source moving towards the man appears to be
Alower than the original frequencyBsame as the original frequencyChigher than the original frequencyDlower as well as higher than the original frequency depending upon the speed of the source.A sound source is moving towards a stationary observer with 1/10 of the speed of sound. The ratio of apparent to real frequency is
A109B(109)2C(1110)2D1110
A listener and a source of sound are moving with the same speed in the same direction. The ratio of the frequency of the source and the frequency which is heard by the listerner is
View SolutionA source of sound is travelling towards a stationary observer. The frequency of sound heard by the observer is 25% more that the actual frequency. If the speed of sound is v, that of the source is
View SolutionA car moves towards a hill with speed vc.It blows a horn of frequency f which is heard by an observer following the car with speed v0.The speed of sound in air is v.
View SolutionA detector is released from rest over a source of sound of frequency f0=103Hz. The frequency observed by the detector at time t is plotted in the graph. The speed of sound in air is (g=10m/s2)
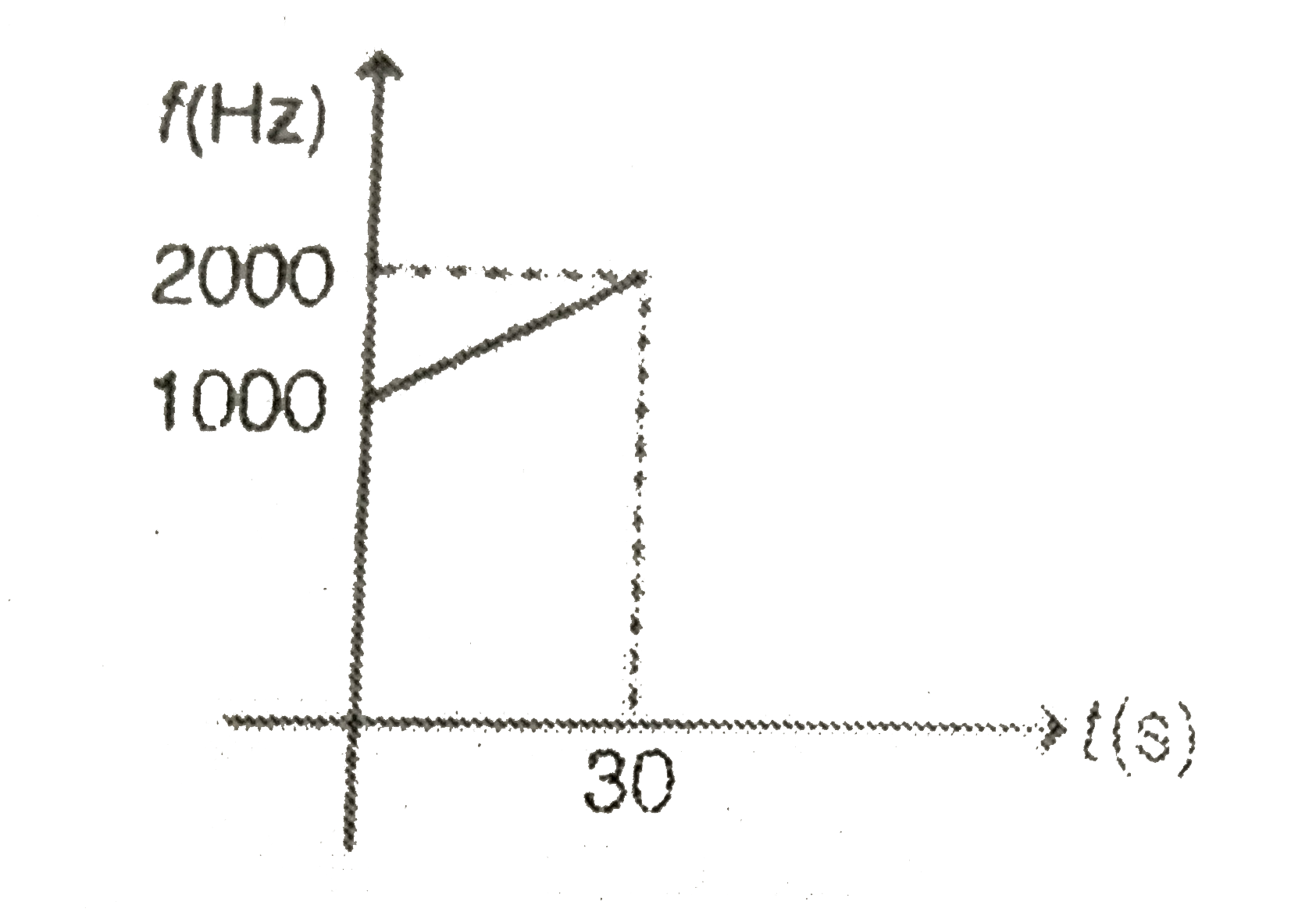
View SolutionA train is moving towards a stationary observer. Which of the following curve best represents the frequency recived by observer f as a function of time?
View Solution
