Question

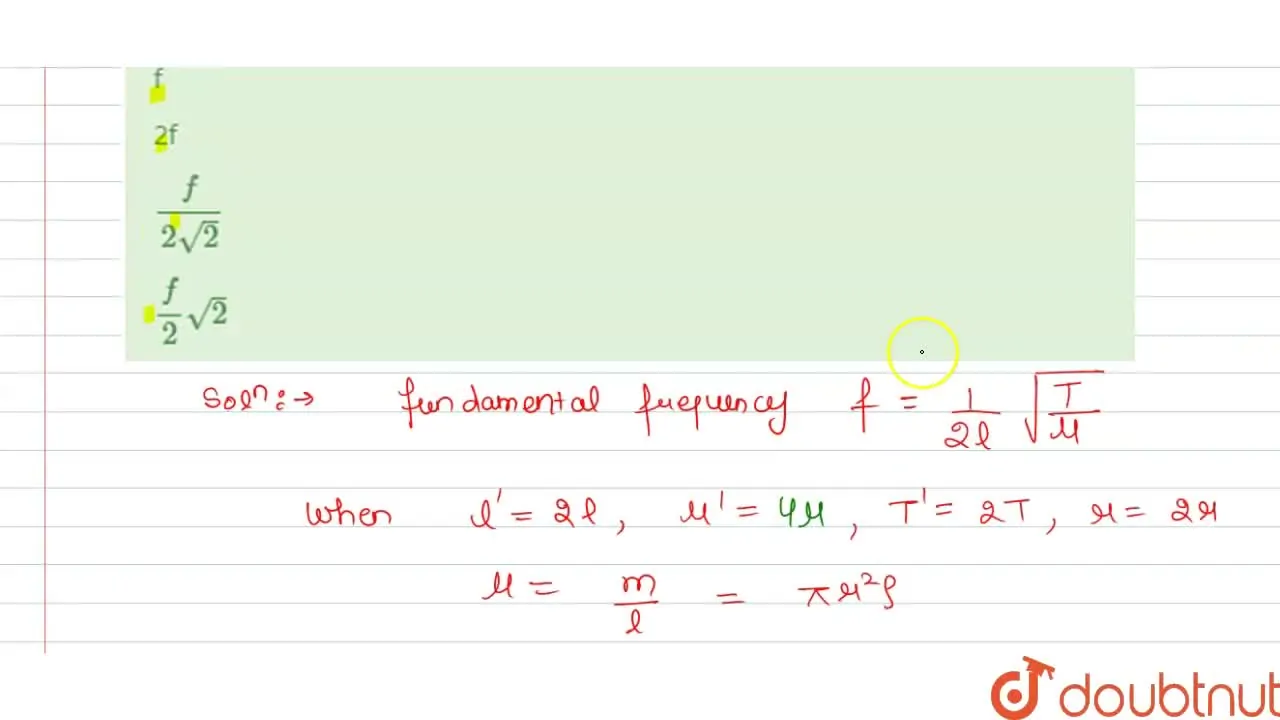
A wire of length l having tension T and radius $r$ vibrates with fundamental frequency $f$. Another wire of the same metal with length $2l$ having tension $2T$ and radius $2r$ will vibrate with fundamental frequency :

Options

A. f
B. 2f
C. `f/(2sqrt2)`
D. `f/2sqrt2`

Explanation

To solve the problem, we need to determine the fundamental frequency of the second wire based on the properties of the first wire.

1. Understand the formula for fundamental frequency:
The fundamental frequency \( f \) of a wire is given by the formula:
\( f = \frac{1}{2L} \sqrt{\frac{T}{\mu}} \)
where \( L \) is the length of the wire, \( T \) is the tension, and \( \mu \) is the mass per unit length of the wire.

2. Identify parameters for the first wire:
For the first wire:
- Length \( L = l \)
- Tension \( T = T \)
- Radius \( r = r \)
- Fundamental frequency \( f = f \)

The mass per unit length \( \mu \) can be expressed as:
\( \mu = \rho \cdot A = \rho \cdot \pi r^2 \)
where \( \rho \) is the density of the material and \( A \) is the cross-sectional area.

3. Identify parameters for the second wire:
For the second wire:
- Length \( L' = 2l \)
- Tension \( T' = 2T \)
- Radius \( r' = 2r \)

4. Calculate mass per unit length for the second wire:
The mass per unit length for the second wire \( \mu' \) is:
\( \mu' = \rho \cdot \pi (r')^2 = \rho \cdot \pi (2r)^2 = \rho \cdot \pi \cdot 4r^2 = 4\mu \)

5. Substitute parameters into the frequency formula for the second wire:
Using the fundamental frequency formula for the second wire:
\( f' = \frac{1}{2L'} \sqrt{\frac{T'}{\mu'}} \)
Substituting the values:
\( f' = \frac{1}{2(2l)} \sqrt{\frac{2T}{4\mu}} = \frac{1}{4l} \sqrt{\frac{2T}{4\mu}} = \frac{1}{4l} \cdot \frac{1}{2} \sqrt{\frac{T}{\mu}} = \frac{1}{8l} \sqrt{\frac{T}{\mu}} \)

6. Relate \( f' \) to \( f \):
Since \( f = \frac{1}{2l} \sqrt{\frac{T}{\mu}} \), we can express \( f' \) in terms of \( f \):
\( f' = \frac{1}{4} f \)

Final Answer:
Thus, the fundamental frequency of the second wire is:
\( f' = \frac{f}{2\sqrt{2}} \)