One face of a rectangular glass plate 6 cm thick is silvered. An object held 8 cm in front of the first face, forms an image 12 cm behind the silvered face. The refractive index of the glass is
To solve the problem, we need to find the refractive index of the glass slab given the distances of the object and the image. Let's break down the solution step by step.
Step 1: Understand the Setup
We have a rectangular glass plate that is 6 cm thick, with one face silvered. An object is placed 8 cm in front of the first face, and an image is formed 12 cm behind the silvered face.
Step 2: Define Distances
- Let the thickness of the glass slab be t=6 cm.
- The object distance u=−8 cm (the negative sign is used because the object is in front of the mirror).
- The image distance v=+12 cm (the positive sign is used because the image is formed behind the silvered face).
Step 3: Use the Concept of Apparent Height
When light travels from air into the glass, it bends according to Snell's law. The apparent height (or distance) can be calculated using the refractive index μ.
The distance of the object from the first face of the glass is 8 cm, and the distance of the image from the silvered face is 12 cm.
Step 4: Calculate the Total Distance
The total distance from the object to the image can be expressed as:
Total Distance=Distance to the first face+Thickness of the glass+Distance from the silvered face to the image
This gives us:
8+6+12=26 cm
Step 5: Relate Distances Using Refractive Index
Using the concept of apparent distance, we can set up the equation:
8μ1+6+12μ=26
This simplifies to:
8μ+6+12μ=26
Step 6: Rearranging the Equation
Rearranging gives us:
8μ+12μ=20
Multiplying through by μ to eliminate the fraction:
8μ2−20μ+12=0
Step 7: Solve the Quadratic Equation
Now we can solve the quadratic equation 8μ2−20μ+12=0 using the quadratic formula:
μ=−b±√b2−4ac2a
Here, a=8, b=−20, and c=12:
μ=20±√(−20)2−4⋅8⋅122⋅8
Calculating the discriminant:
μ=20±√400−38416
μ=20±√1616
μ=20±416
Calculating the two possible values:
μ1=2416=1.5andμ2=1616=1
Step 8: Determine the Valid Refractive Index
Since the refractive index of glass is typically greater than 1, we take:
μ=1.5
Final Answer
The refractive index of the glass slab is μ=1.5.
We have a rectangular glass plate that is 6 cm thick, with one face silvered. An object is placed 8 cm in front of the first face, and an image is formed 12 cm behind the silvered face.
- Let the thickness of the glass slab be t=6 cm.
- The object distance u=−8 cm (the negative sign is used because the object is in front of the mirror).
- The image distance v=+12 cm (the positive sign is used because the image is formed behind the silvered face).
When light travels from air into the glass, it bends according to Snell's law. The apparent height (or distance) can be calculated using the refractive index μ.
The total distance from the object to the image can be expressed as:
Total Distance=Distance to the first face+Thickness of the glass+Distance from the silvered face to the image
This gives us:
8+6+12=26 cm
Using the concept of apparent distance, we can set up the equation:
8μ1+6+12μ=26
This simplifies to:
8μ+6+12μ=26
Rearranging gives us:
8μ+12μ=20
Multiplying through by μ to eliminate the fraction:
8μ2−20μ+12=0
Now we can solve the quadratic equation 8μ2−20μ+12=0 using the quadratic formula:
μ=−b±√b2−4ac2a
Here, a=8, b=−20, and c=12:
μ=20±√(−20)2−4⋅8⋅122⋅8
Calculating the discriminant:
μ=20±√400−38416
μ=20±√1616
μ=20±416
Calculating the two possible values:
μ1=2416=1.5andμ2=1616=1
Since the refractive index of glass is typically greater than 1, we take:
μ=1.5
The refractive index of the glass slab is μ=1.5.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - EXERCISE
45 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - A)
39 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Assignment (Section - J) Aakash Challengers Questions
7 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
- SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)
10 Videos
Similar Questions
Onc face of a rectangular glass plate 6 cm thick is silcerd. An object held 8 cm in front of the first face, froms an image 12 cm behind the silvered face. The refractive index of the glass is
View SolutionOne face of a rectangular glass plate 6cm thick is silvered. An object held 8cm in front of the unsilvered face forms an image 10cm behind the silvered face.Find the refractive index of glass. Consider all the three steps.
View SolutionLight from a luminious point on the lower face of a rectangular glass slab , 2 cm thick, strikes the upper face and the totally reflected rays outline a circle of 3.2 cm radius on the lower face. Find the refractive index of the glass.
View SolutionAn object O is placed at 8cm in front of a glass slab, whose one face is silvered as shown in Figure. The thickness of the slab is 6cm. If the image formed 10 cm behind the silvered face, find the refractive index of glass.
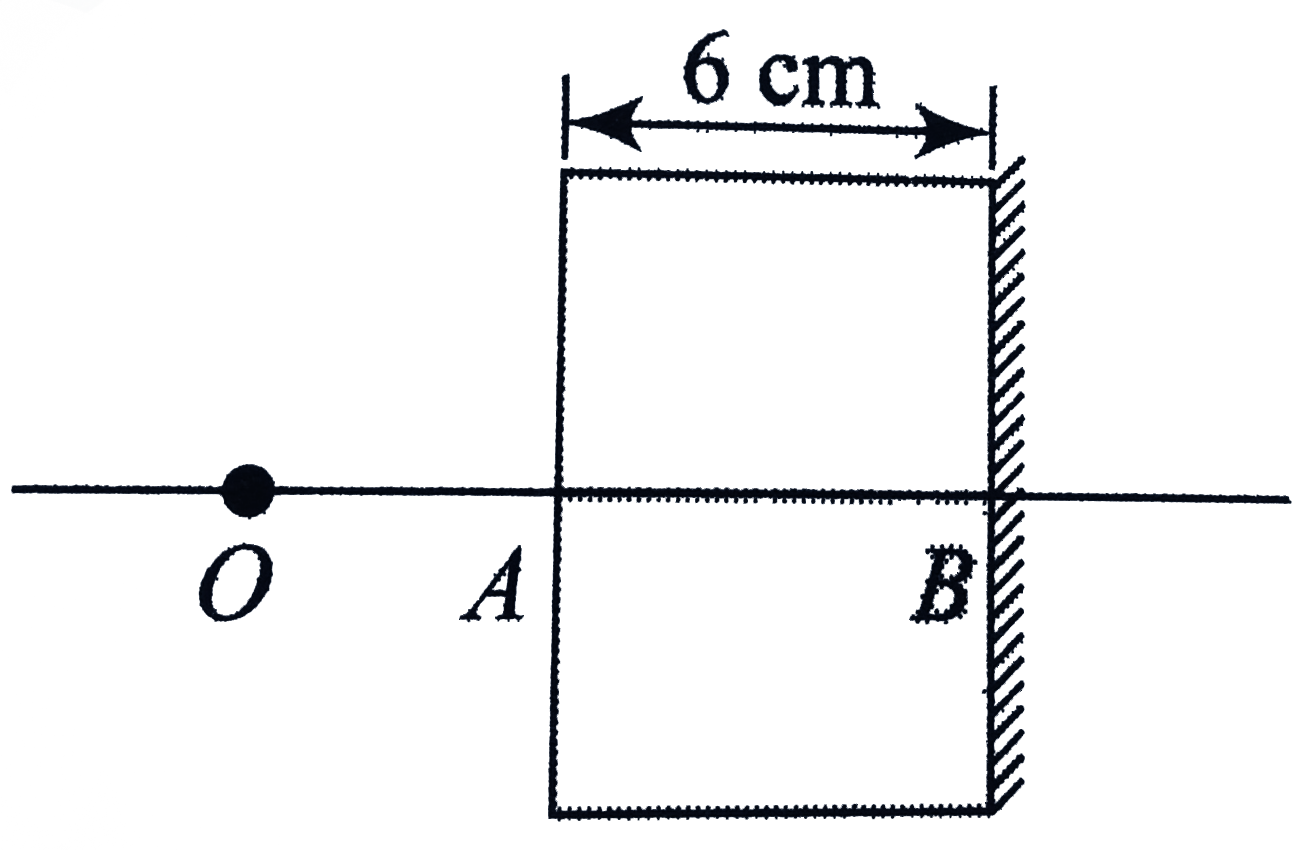
View SolutionA small object is placed 20 cm in front of a block of glass 10 cm thick and its farther side silvered. The image is formed 22 cm behind the silvered face. Find the refractive index of glass.
View SolutionA beam of parallel rays of width b cm propagates in glass at an angle θ to its plane face. What would the beam width b1 be after it goes over to air thrugh this face? (The refractive index of the glass is μ)
View SolutionA plane mirror is made of glass slab (μg=1.5) 2.5 cm thick and silvered on back. A point object is placed 5 cm in front of the unsilvered face of the mirror. What will be the position of final image ?
View SolutionAn object is at a distance of d=2.5cm from the surface of a glass sphere with a radius R=10cm. Find the position of the final image produced by the sphere. The refractive index of glass is μ=1.5.
View SolutionAn isosceles glass prism has one of its faces silvered. A light ray is incident normally on the other face which is identical in size to the silvered face. The light ray is reflected twice on the same sized faces and emerges through the base of the prism perpendicularly. Find the minimum value of refractive index of the material of the prism.
View SolutionA ray of light is incident on one face of a transparent glass slab of thickness 9cm at an angle of incidence 60∘. Lateral displacement of the ray on emerging from the opposite parallel face is 3√3cm. Refractive index of the material of slab is:
View Solution
