Water from a tap emerges vertically downwards with an initial velocity V0. Assume pressure is constant throughout the stream of water and the flow is steady. Find the distance form the tap at which cross-sectional area of stream is half of the cross-sectional area of stream at the tap.
V20/2g
3V20/2g
2V20/g
5V20/2g
The correct Answer is:B
To solve the problem step by step, we will use the principles of fluid mechanics, specifically the equations of motion and the continuity equation.
Step 1: Understand the Problem
Water is flowing from a tap vertically downward with an initial velocity V0. We need to find the distance h from the tap where the cross-sectional area of the stream is half of the area at the tap.
Step 2: Use the Continuity Equation
The continuity equation states that the product of the cross-sectional area and the velocity of the fluid must remain constant along the flow. Mathematically, this is expressed as:
A1V1=A2V2
Where:
- A1 = cross-sectional area at the tap
- V1 = initial velocity V0
- A2 = cross-sectional area at distance h
- V2 = velocity at distance h
Given that A2=12A1, we can substitute this into the continuity equation:
A1V0=(12A1)V2
This simplifies to:
V2=2V0
Step 3: Use the Equation of Motion
Next, we apply the equation of motion to find the relationship between the velocity and the height. The relevant equation is:
V22=V20+2gh
Substituting V2=2V0 into this equation gives:
(2V0)2=V20+2gh
This simplifies to:
4V20=V20+2gh
Step 4: Rearranging the Equation
Now, we can rearrange the equation to solve for h:
4V20−V20=2gh
3V20=2gh
h=3V202g
Final Answer
The distance from the tap at which the cross-sectional area of the stream is half of the cross-sectional area at the tap is:
h=3V202g
---
Water is flowing from a tap vertically downward with an initial velocity V0. We need to find the distance h from the tap where the cross-sectional area of the stream is half of the area at the tap.
The continuity equation states that the product of the cross-sectional area and the velocity of the fluid must remain constant along the flow. Mathematically, this is expressed as:
A1V1=A2V2
Where:
- A1 = cross-sectional area at the tap
- V1 = initial velocity V0
- A2 = cross-sectional area at distance h
- V2 = velocity at distance h
A1V0=(12A1)V2
This simplifies to:
V2=2V0
Next, we apply the equation of motion to find the relationship between the velocity and the height. The relevant equation is:
V22=V20+2gh
Substituting V2=2V0 into this equation gives:
(2V0)2=V20+2gh
This simplifies to:
4V20=V20+2gh
Now, we can rearrange the equation to solve for h:
4V20−V20=2gh
3V20=2gh
h=3V202g
The distance from the tap at which the cross-sectional area of the stream is half of the cross-sectional area at the tap is:
h=3V202g
Topper's Solved these Questions
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Multipe Correct
Explore 15 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Assertion-Reasoning
Explore 8 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Subjective
Explore 25 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Integer
Explore 2 Videos
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
Water from a tap emerges vertically downwards with an initial spped of 1.0ms−1. The cross-sectional area of the tap is 10−4m2. Assume that the pressure is constant throughout the stream of water, and that the flow is steady. The cross-sectional area of the stream 0.15 m below the tap is
View SolutionWater from a tap emerges vertically downward with an initial speed of 3.0 m/s. The cross-sectional area of the tap is 10−4m2 . Assume that the pressure is constant throughout the stream of water and that the flow is steady. The cross-sectional area of the stream 2 m below the tap is:
View SolutionWater from a tap emerges vertically downwards with initial velocity 4ms−1. The cross-sectional area of the tap is A. The flow is steady and pressure is constant throughout the stream of water. The distance h vertically below the tap, where the cross-sectional area of the stream becomes (23)A is (g=10m/s2)
View SolutionThe water flowing through the same tube having two different cross section areas has different flow rates .
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
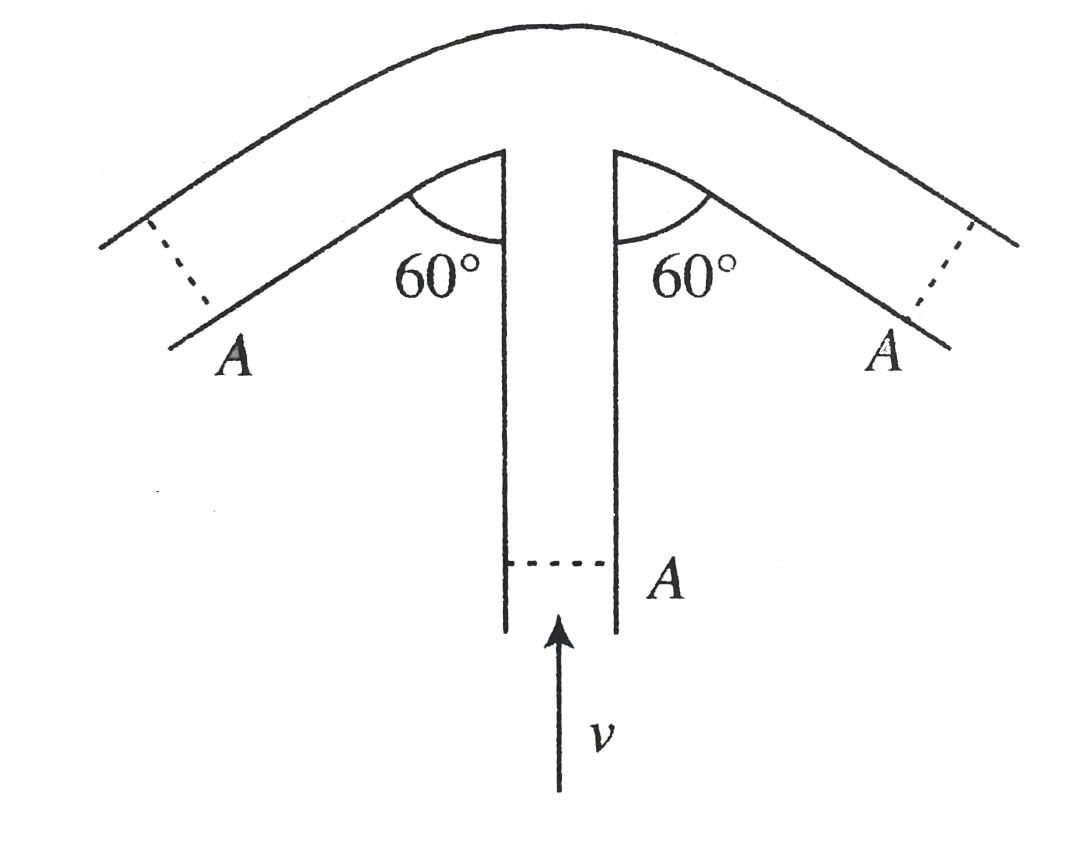
View SolutionAn ideal liquid of density ρ is pushed with velocity v through the central limb of the tube shown in fig. What force does the liquid exert on the tube? The cross sectional areas of the three limbs are equal to A each. Assume stream-line flow.
View SolutionThe ends of a long bar are maintained at different temperatures and there is no loss of heat from the sides of the bar due to conduction or radiation. The graph of temperature against distance of the bar when it has attained steady state is shown here. The graph shows (i) the temperature gradient is not uniform.
(ii) the bar has uniform cross-sectional area.
(iii) the cross-sectional area of the bar increases as the distance from the hot end increases.
(iv) the cross-sectional area of the bar becreases as the distance from the hot end increases
View SolutionA large cylindrical tank has a hole of area A at its bottom. Water is oured in the tank by a tube of equal cross sectional area A ejecting water at the speed v.
View SolutionWater is filled to height h in a fixed vertical cylinder placed on horizontal surface. At time t=0 a small hole a drilled at a height h/4 from bottom of cylinder as shown. The cross section area of hole is a and the cross-section area of cylinder is A such that A>>a.
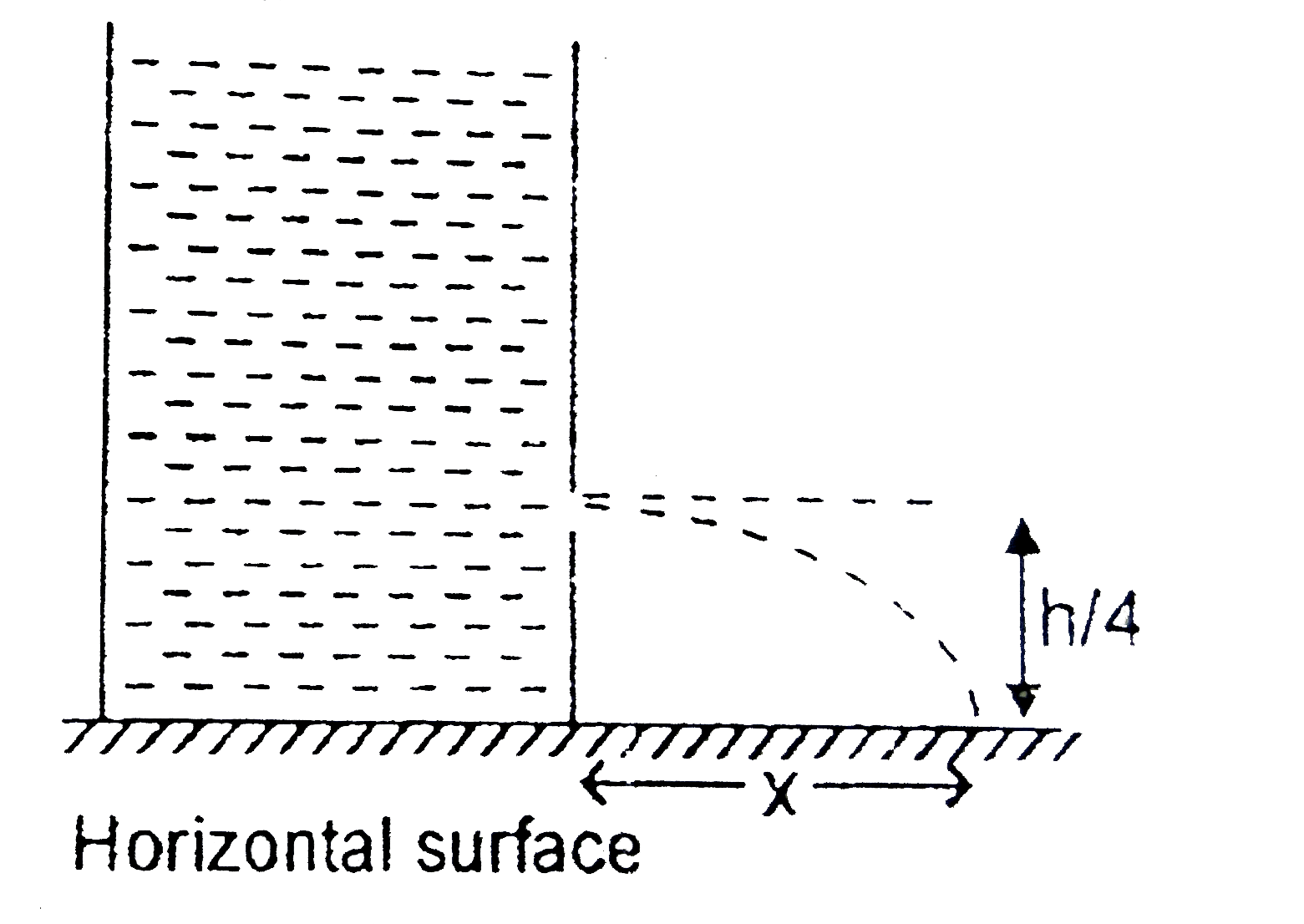
Let the value of horizontal distance of point where the4 water fall on horizontal surface from bottom of cylinder be x as shown. Then from time t=0 till water comes out of hole, pick the correct statement:
View Solution