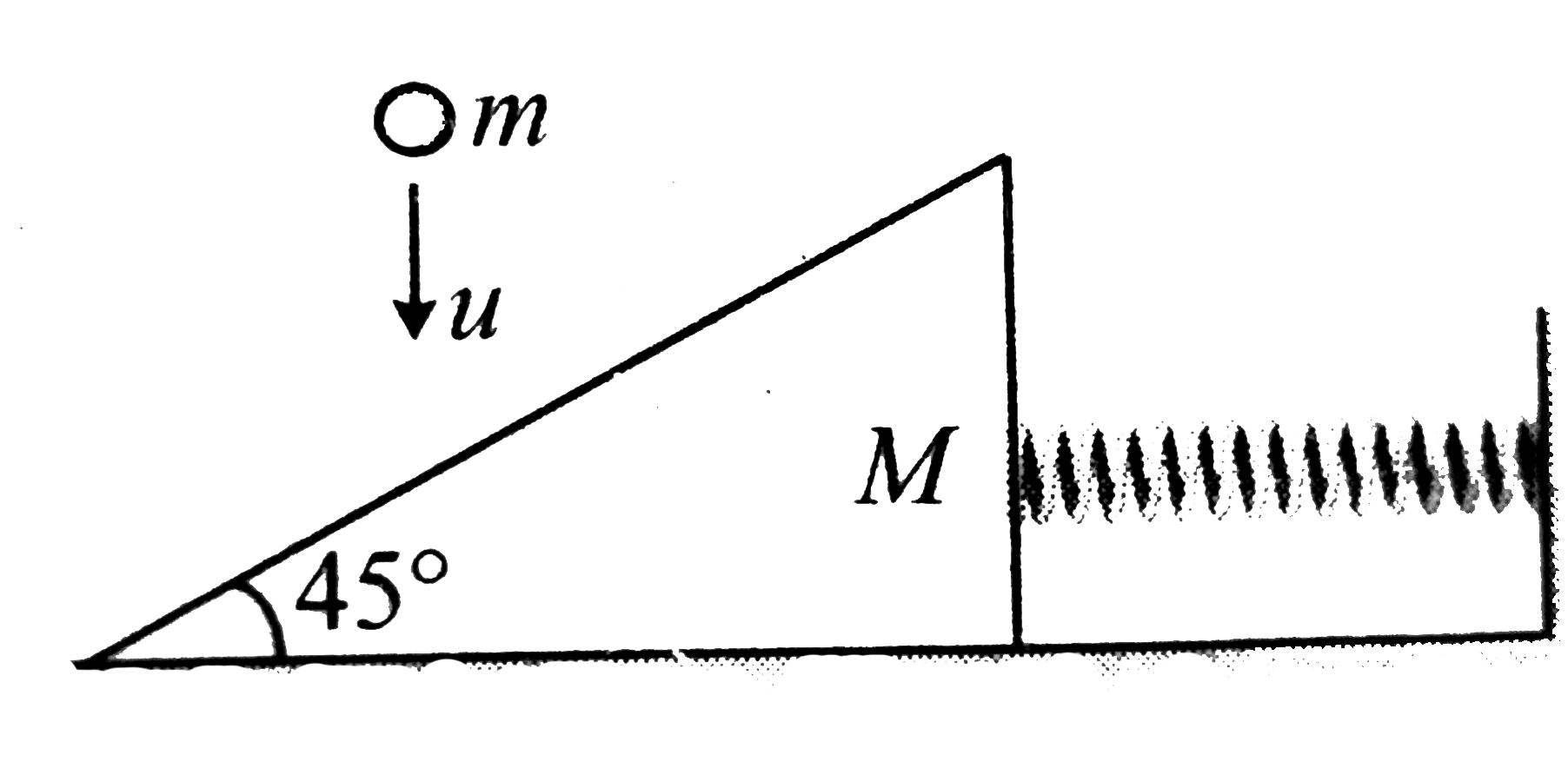
A ball of mass m hits a wedge of mass M vertically with speed u, which is placed, on a smooth horizontal surface. Find the maximum compression in the spring, if the collision is perfectly elastic and no friction any where. Spring constant of spring is K.
The correct Answer is:√MK
Let after collision, the ball has velocity v1 and wedge v2 as shown.
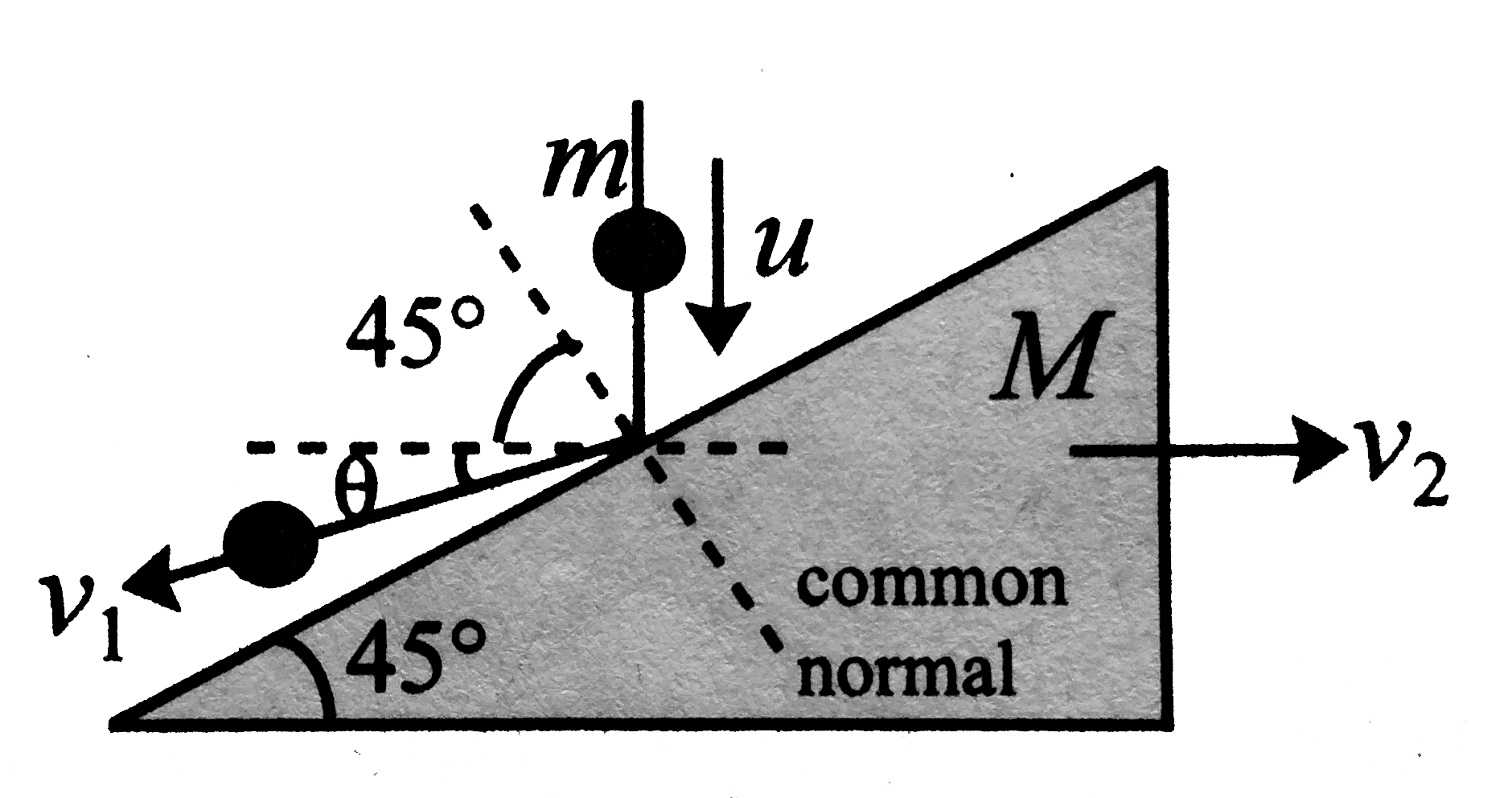
Aplying conservation of momentum in horizontal direction
mv1cosθ=Mv2 ……….i
From Newton's experimental law:
e=1=v2sin45∘−[−v1cos(45+θ)]ucos45∘
⇒u=v2+v1cosθ−v1θ ...........ii
Velocity component of the ball along the surfasce of wedge will remain the same as during collision there is no force on the ball in this direction So
usin45∘=v1cos(45−θ)⇒u=v1cosθ+v1sinθ...iii
From i and ii and iii
v2=2mum+2M
To find maximum compression in the sprig, after collision apply conservation of energy for the system of wedge and spring.
12Mv22=12Kx2
⇒x=√Mxv2⇒x(2mum+2M)√MK
Topper's Solved these Questions
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Single Correct
Explore 141 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Multiple Correct
Explore 25 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Exercise 1.3
Explore 24 Videos
- CALORIMETRYBook:CENGAGE PHYSICS ENGLISHChapter:CALORIMETRYExercise:Solved Example
Explore 13 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Integer
Explore 2 Videos
Similar Questions
A block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. The maximum compression in spring is

View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

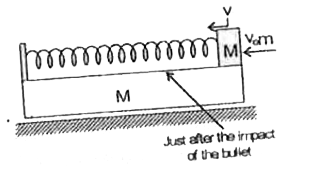
View SolutionIn the figure shown, initially the spring is in relaxed position. A bullet of mass m hits the block 'A' with horizontal speed v0 and gets embedded into it. Find the maximum compression in the spring. Stiffness of the spring is K. Neglect any friction and deformation in the spring during collision.
View SolutionA block of mass m is relesed from rest from a height h onto a smooth surface of mass M fitted with an ideal spring of stiffness k. find the maximum compression in the spring.

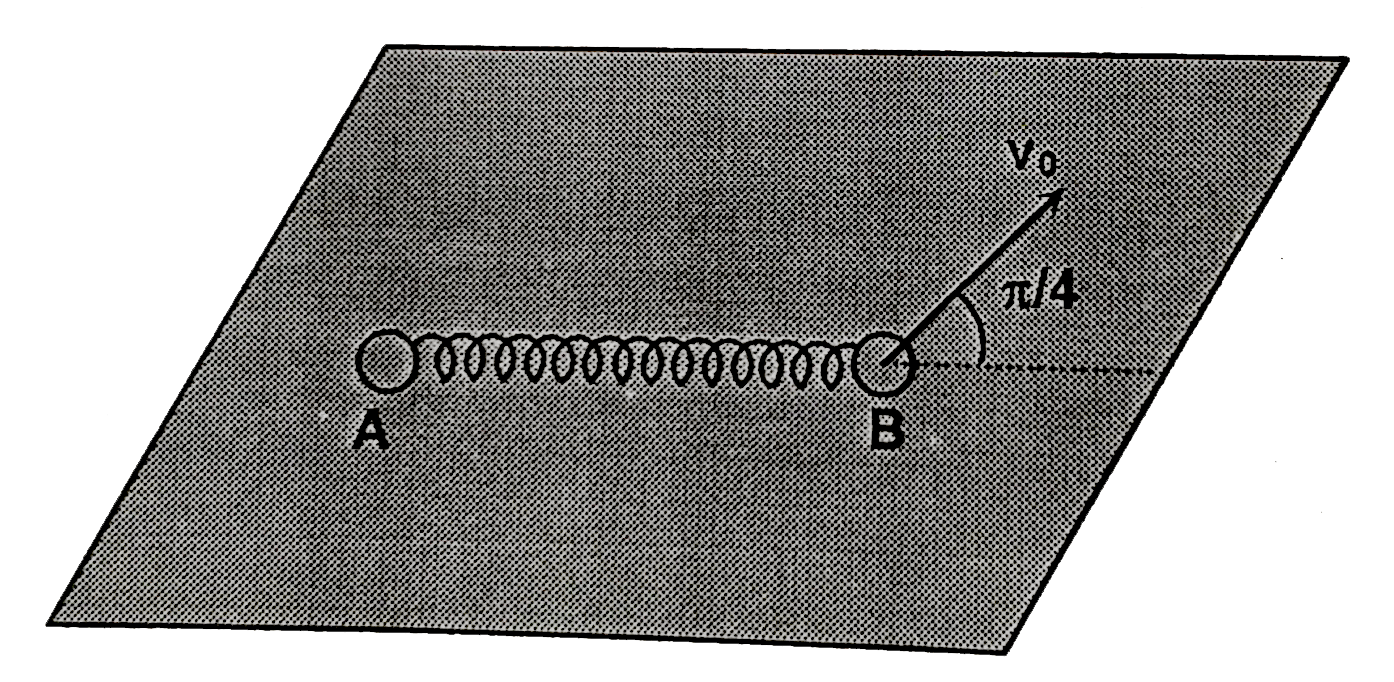
View SolutionA dumbbell consists of two balls A and B of mass m=1 kg and connected by a spring. The whole system is placed on a smooth horizontal surface as shown in the figure. Initially the spring is at its natural length, the ball B is imparted a velocity v0=8√7m/s in the direction shown. The spring constant of the spring is adjusted so that the length of the spring at maximum elongation is twice that of the natural, length of the spring. Find the maximum potential energy stored (in Joule) in the spring during the motion.
View SolutionA ball of mass m is projected with a speed v into the barrel of a spring gun of mass M initially at rest lying on a frictionless surface. The mass sticks in the barrel at the point of maximum compression in the spring. The fraction of kinetic energy of the ball stored in the spring is
View SolutionThe block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionA block of mass m strikes a light pan fitted with a vertical spring after falling through a distance h. If the stiffness of the spring is k, find the maximum compression of the spring.
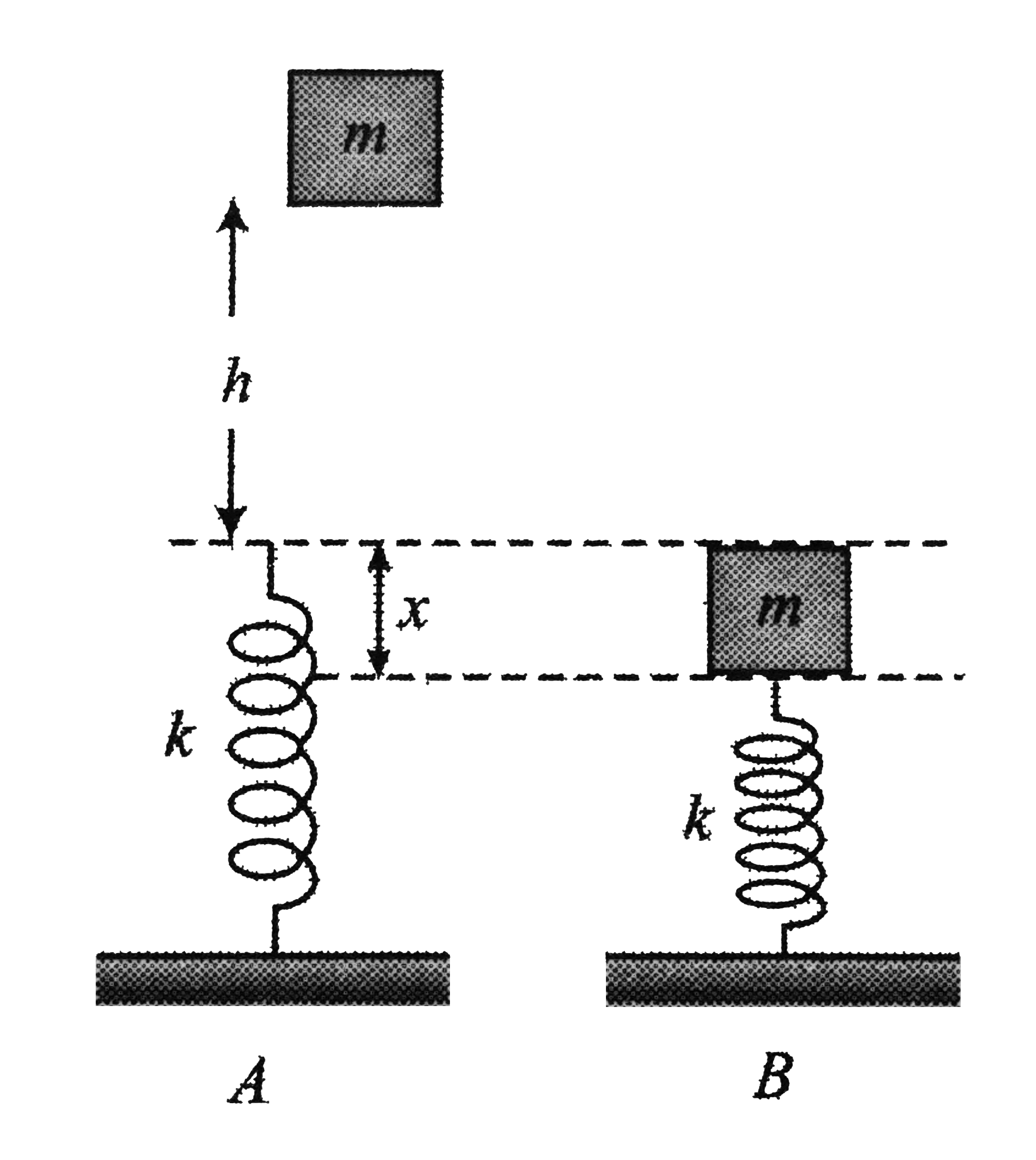
View SolutionA block of mass m is released from a height h from the top of a smooth surface. There is an ideal spring of spring constant k at the bottom of the track. Find the maximum compression in the spring (Wedge is fixed)

View SolutionA block of 10 g slides on smooth horizontal surface with 20 m/s towards a spring of spring constant 100 N/m placed horizontally (as shown in figure). The maximum compression in spring is

View Solution
