Two wooden plank of mass smooth surface. A bullet of mass strikes the block and pierces through it, then strikes the plank and sticks to its. Consequently both the planks move with equal velocities. Find the percentage change in speed of the bullet when it escapes from the first block.
The correct Answer is:
To solve the problem, we will use the principle of conservation of momentum. Let's break down the solution step by step.
Step 1: Understand the system and initial conditions
We have two wooden planks, M1=1kg and M2=2.98kg, and a bullet of mass m=20g=0.02kg. The bullet strikes plank M1 and then pierces through it, subsequently hitting plank M2 and sticking to it.
Step 2: Apply conservation of momentum for the bullet and plank M1
When the bullet strikes plank M1, we can write the conservation of momentum as:
mu=M1v+mu1(1)
where:
- u = initial velocity of the bullet,
- v = velocity of plank M1 after the bullet passes through,
- u1 = velocity of the bullet after passing through plank M1.
Step 3: Apply conservation of momentum for the bullet and plank M2
After the bullet exits plank M1, it strikes plank M2 and sticks to it. The conservation of momentum for this scenario is:
mu1=(M2+m)V(2)
where V is the common velocity of both planks after the bullet sticks to plank M2.
Step 4: Relate the velocities
From equation (2), we can express V:
V=mu1M2+m
Step 5: Substitute V into equation (1)
Substituting V into equation (1):
mu=M1v+mu1
We can express v in terms of u and u1:
v=mu−mu1M1
Step 6: Find the relationship between u and u1
We can express u1 in terms of u:
u1=M1u+mu−M1Vm
Substituting V from step 4 into this equation gives us a relationship between u and u1.
Step 7: Calculate the percentage change in speed
The percentage change in speed of the bullet is given by:
Percentage Change=u1−uu×100
Step 8: Substitute values and simplify
Substituting the values:
- M1=1kg
- M2=2.98kg
- m=0.02kg
We find:
Percentage Change=−M1M2+m×100
Calculating this gives:
Percentage Change=−12.98+0.02×100=−13×100≈−33.33%
Thus, the percentage change in speed of the bullet when it escapes from the first block is approximately 33.33%.
We have two wooden planks, M1=1kg and M2=2.98kg, and a bullet of mass m=20g=0.02kg. The bullet strikes plank M1 and then pierces through it, subsequently hitting plank M2 and sticking to it.
When the bullet strikes plank M1, we can write the conservation of momentum as:
mu=M1v+mu1(1)
where:
- u = initial velocity of the bullet,
- v = velocity of plank M1 after the bullet passes through,
- u1 = velocity of the bullet after passing through plank M1.
After the bullet exits plank M1, it strikes plank M2 and sticks to it. The conservation of momentum for this scenario is:
mu1=(M2+m)V(2)
where V is the common velocity of both planks after the bullet sticks to plank M2.
From equation (2), we can express V:
V=mu1M2+m
Substituting V into equation (1):
mu=M1v+mu1
We can express v in terms of u and u1:
v=mu−mu1M1
We can express u1 in terms of u:
u1=M1u+mu−M1Vm
Substituting V from step 4 into this equation gives us a relationship between u and u1.
The percentage change in speed of the bullet is given by:
Percentage Change=u1−uu×100
Substituting the values:
- M1=1kg
- M2=2.98kg
- m=0.02kg
Percentage Change=−M1M2+m×100
Calculating this gives:
Percentage Change=−12.98+0.02×100=−13×100≈−33.33%
Topper's Solved these Questions
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Single Correct
Explore 141 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Multiple Correct
Explore 25 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:Exercise 1.3
Explore 24 Videos
- CALORIMETRYBook:CENGAGE PHYSICS ENGLISHChapter:CALORIMETRYExercise:Solved Example
Explore 13 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Integer
Explore 2 Videos
Similar Questions
A 20g bullet pierces through a plate of mass M1=1kg and then comes to rest inside a second plate of mass M2=2.98kg as shown in Fig. It is found that the two plates, initially at rest, now move with equal velocities. Find the percentage loss in the initial velocity of the bullet when it is between M1 and M2. Neglect any loss of material of the plates due to the action of bullet.
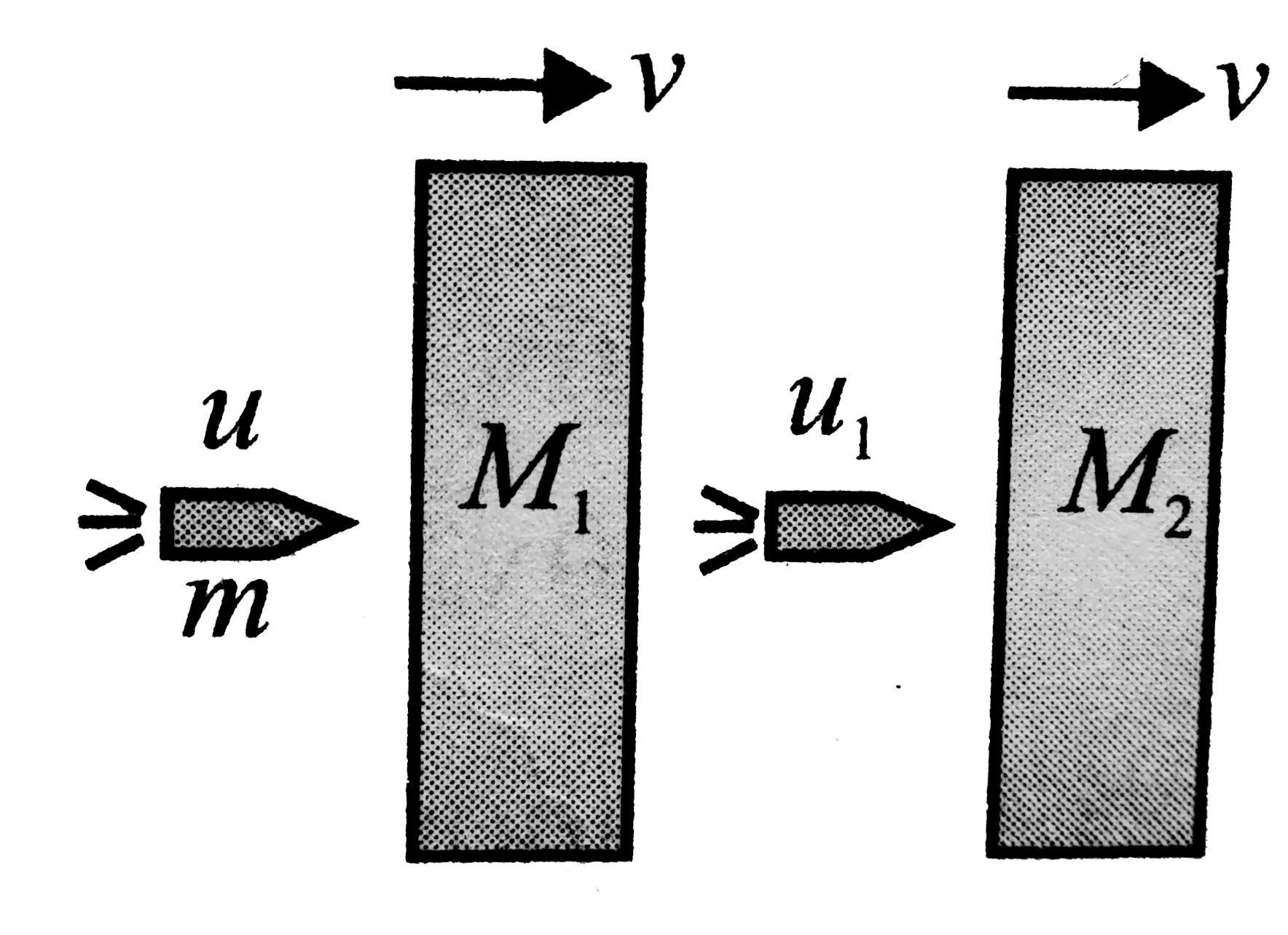
View SolutionA bullet moving at 20 m/sec. strikes a wooden plank and penetrates 4 cm before coming to stop. The time taken to stop is
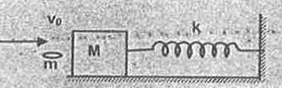
View SolutionA bullet of mass m strikes a block of mass M connected to a light spring of stiffness k, with a speed v0 and gets into it. Find the loss of K.E. of the bullet.
View SolutionA wooden plank of mass 20kg is resting on a smooth horizontal floor. A man of mass 60kg starts moving from one end of the plank to the other end. The length of the plank is 10m. Find the displacement of the plank over the floor when the man reaches the other end of the plank.

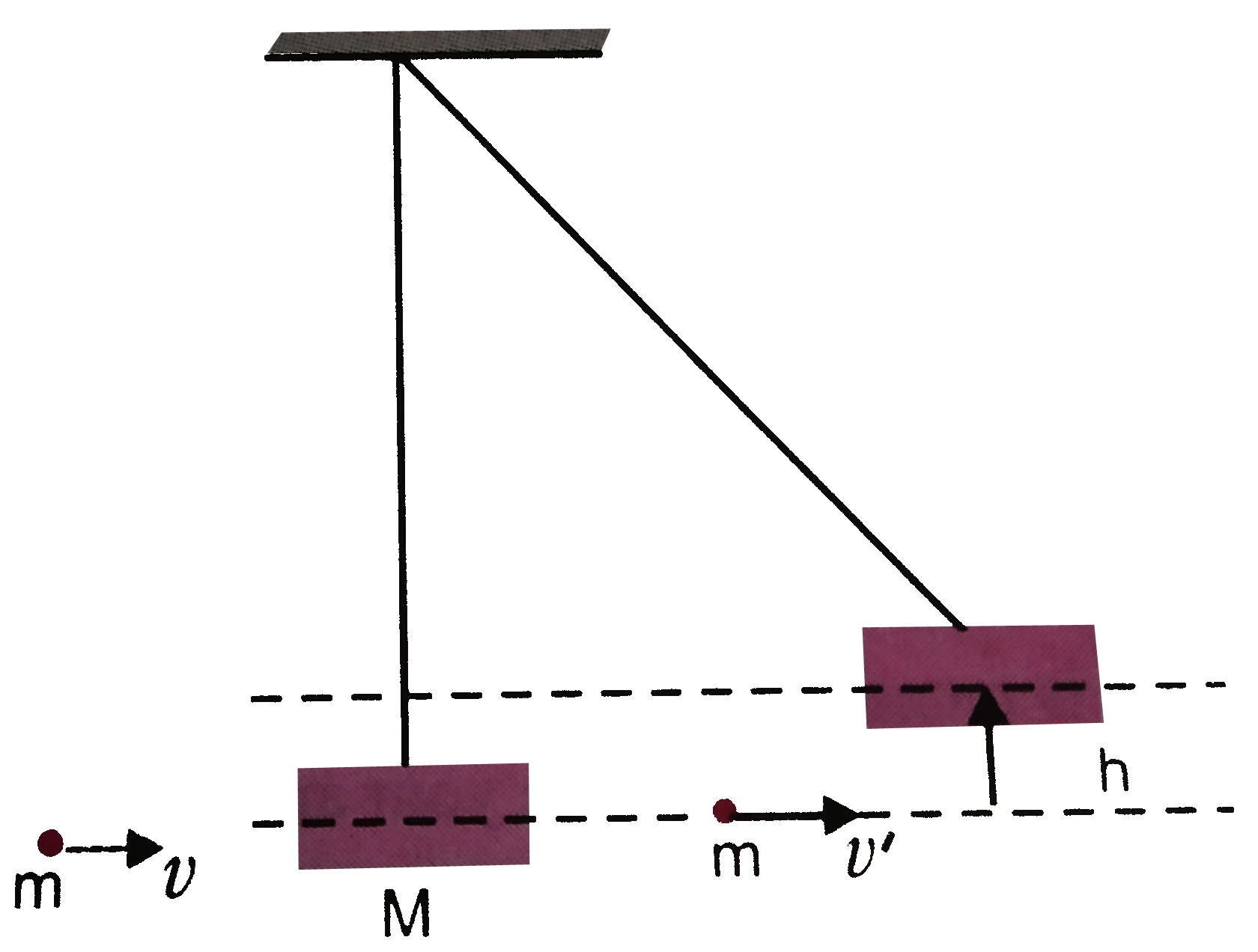
View SolutionA bullet of mass 0.01kg and travelling at a speed of 500ms−1 strikes a block of mass 2kg which is suspended by a string of length 5m. The centre of gravity of the block is found to raise a vertical distance of 0.2m. What is the speed of the bullet after it emerges from the block?
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionA bullet of mass 0.01 kg and travelling at a speed of 500 m/s strikes a block of mass 2 kg which is suspended by a string of length 5m. The center of gravity of the block is found to rise a vertical distance of 0.1m, figure. What is the speed of the bullet after it emerges from the block ? (g=9.8m/s2).
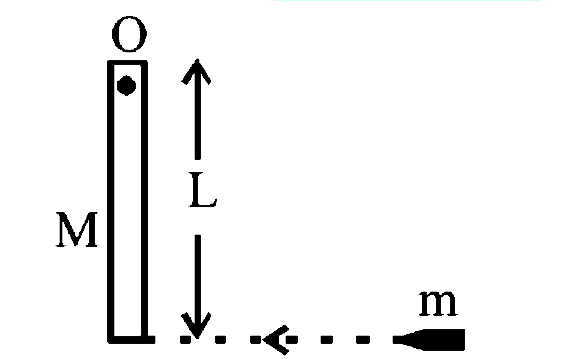
View SolutionA wooden log of mass M and length L is hinged by a frictionless nail at O.A bullet of mass m strikes with velocity v and sticks to it. Find angular velocity of the system immediately after the collision aboutO.
View SolutionA bullet of mass m moving with velocity υ1 strikes a suspended wooden block of mass M as shown in the figure and sticks to it. If the block rises to a height y. the initial of the bullet is

View SolutionA plank of mass 5 kg in kept on a smooth surface. A block of 1 kg is kept on it and is given a velcoity of 12 m/s. There is friction between the block and the plank. Assuming the plank to be very long, find the magnitude of work done (in Joules) by friction on block till the relative sliding between them stops.

View Solution