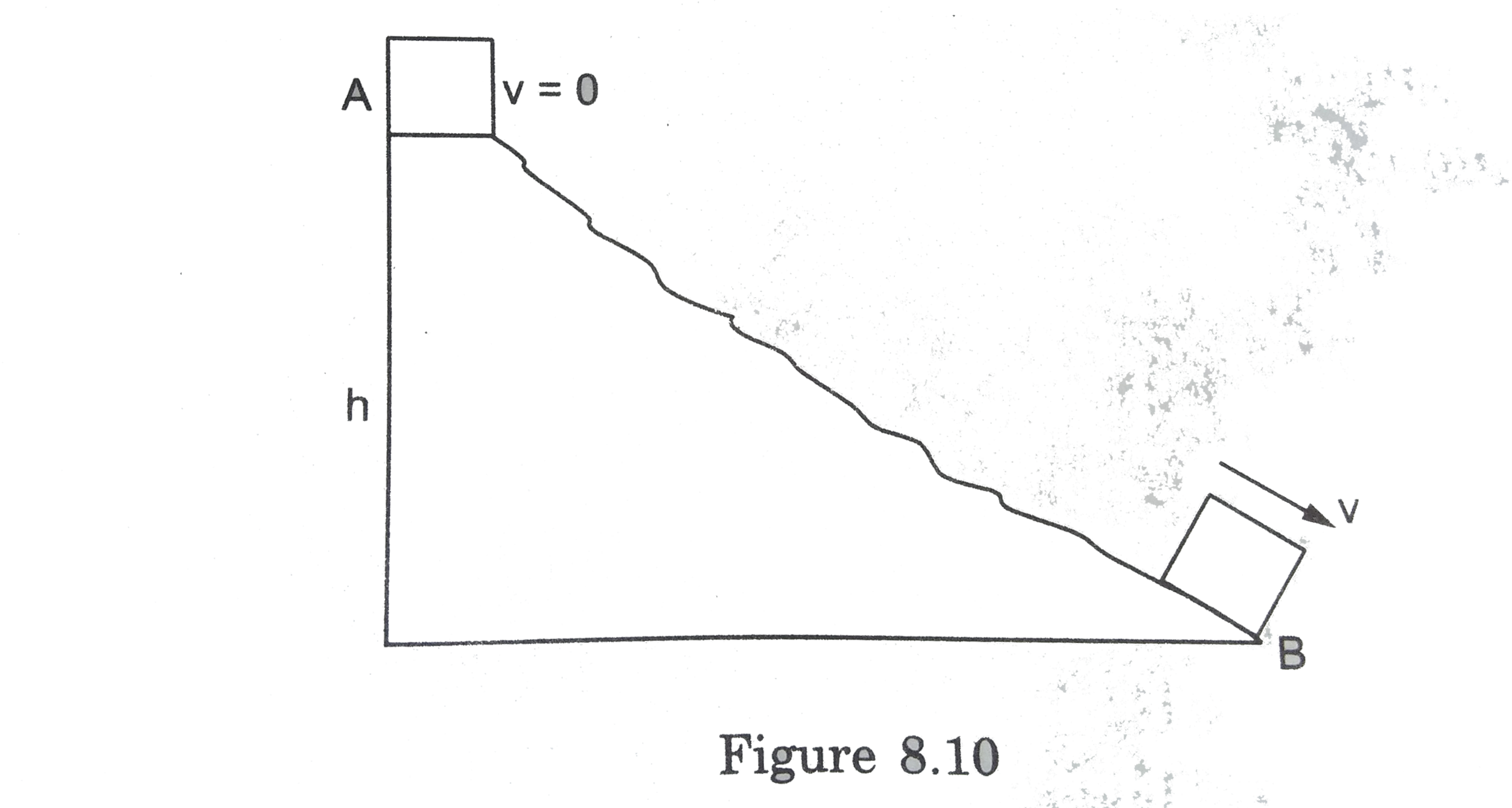
Block of mass m are released from rest and they slide down the inclined surface as shown in figure. Show that the work done by the frictional forces on the blocks are same in all the cases. Also calculate the speeds with which the blocks reach point B. The coefficient of friction for all the surface is μk.
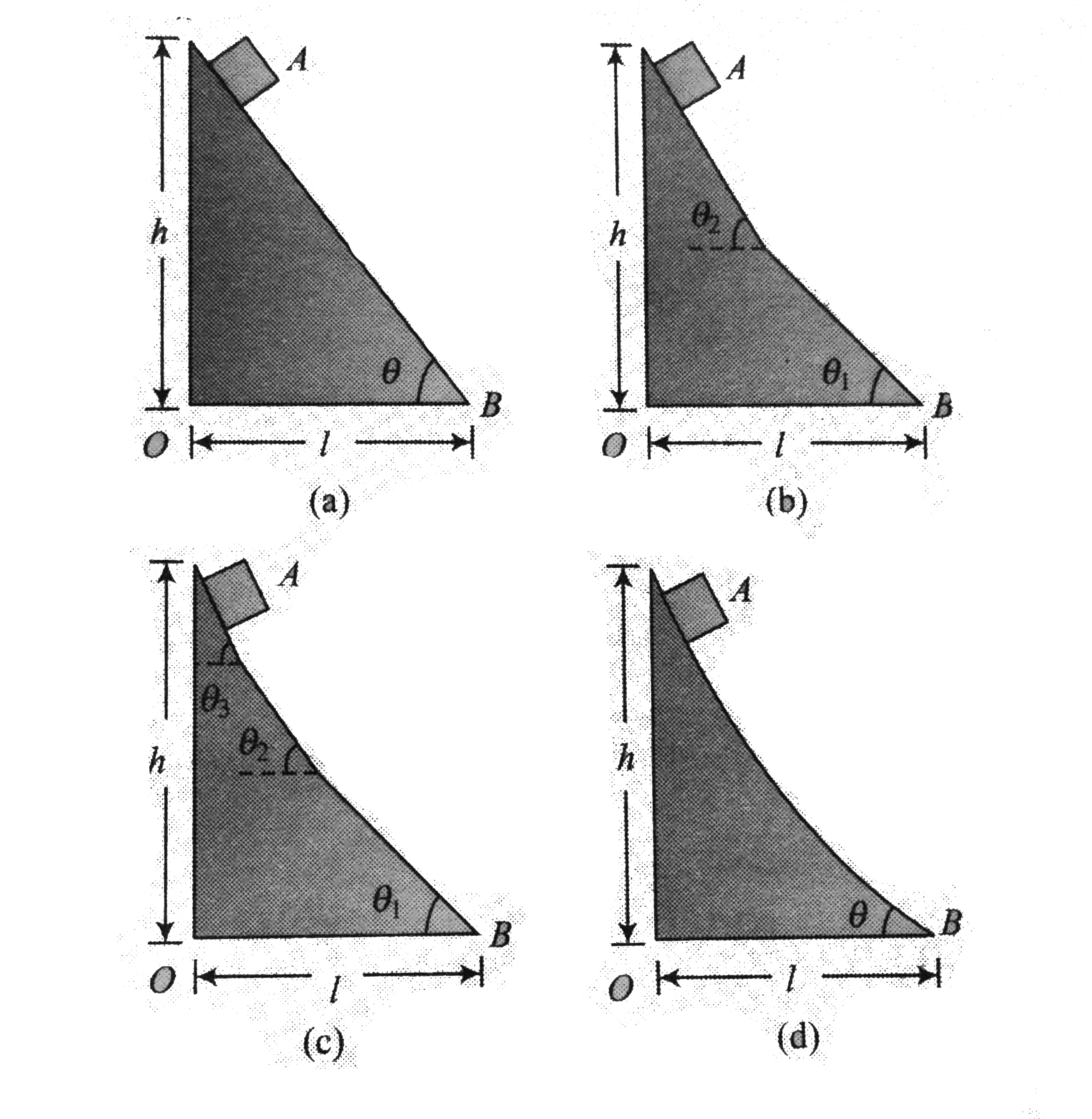
For figure (a)
Frictional force on the block =μkN=μkmgcosθ
If S be the length of the incline, work done by the frictional force on the block when the block slides down from A to B,
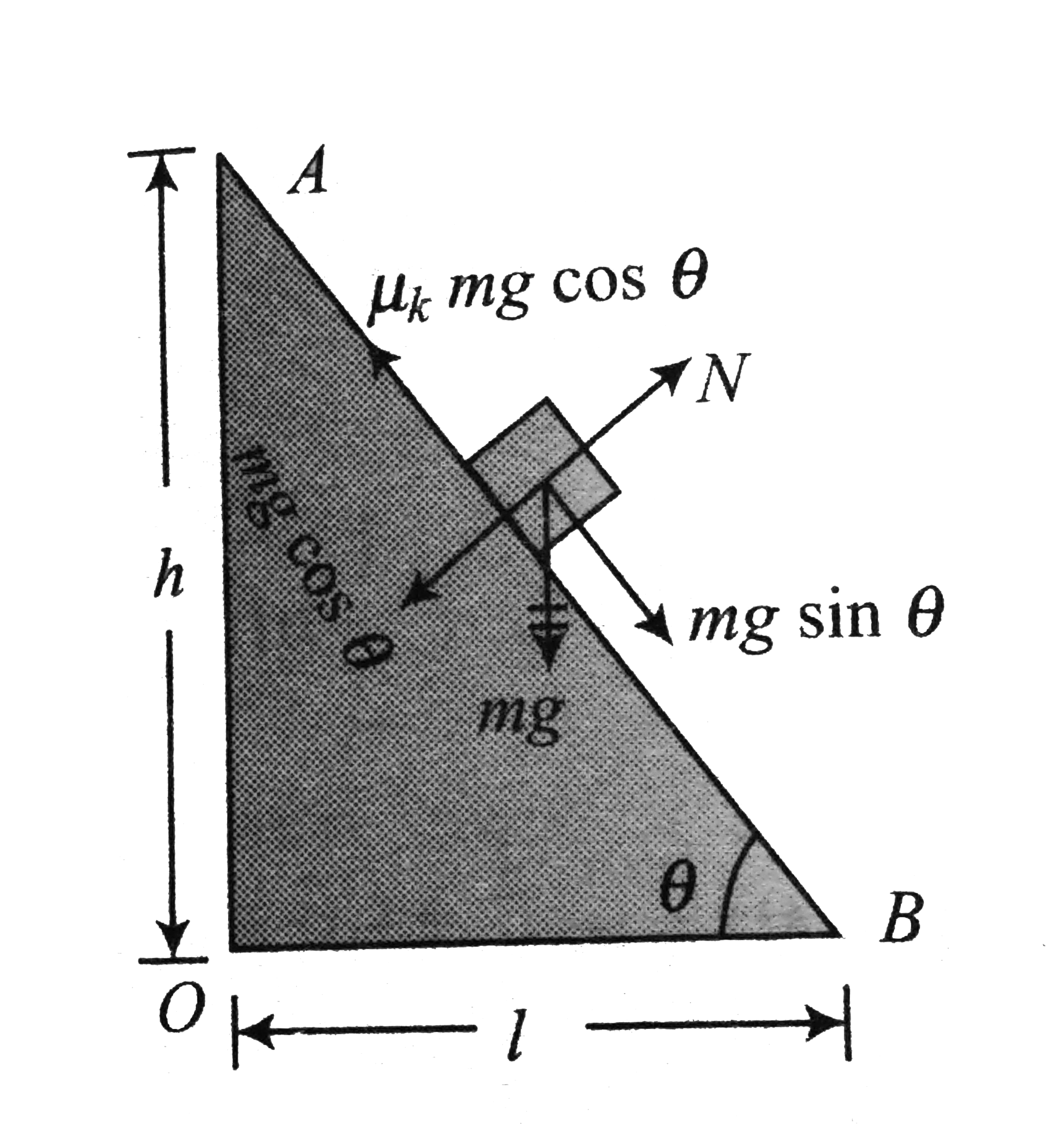
Wfl=(μkmgcosθ)(s)
{Force and displacement are antiparallel}
=−μkmg(scosθ)=−μkmg
Figure (b)
Frictional forces on the block at the plans with the inclination θ1 and θ2 are μkmgcosθ1 and μkcosθ2. If the lengths of the planes be S1 and S2, work done by fricitional force on the block,
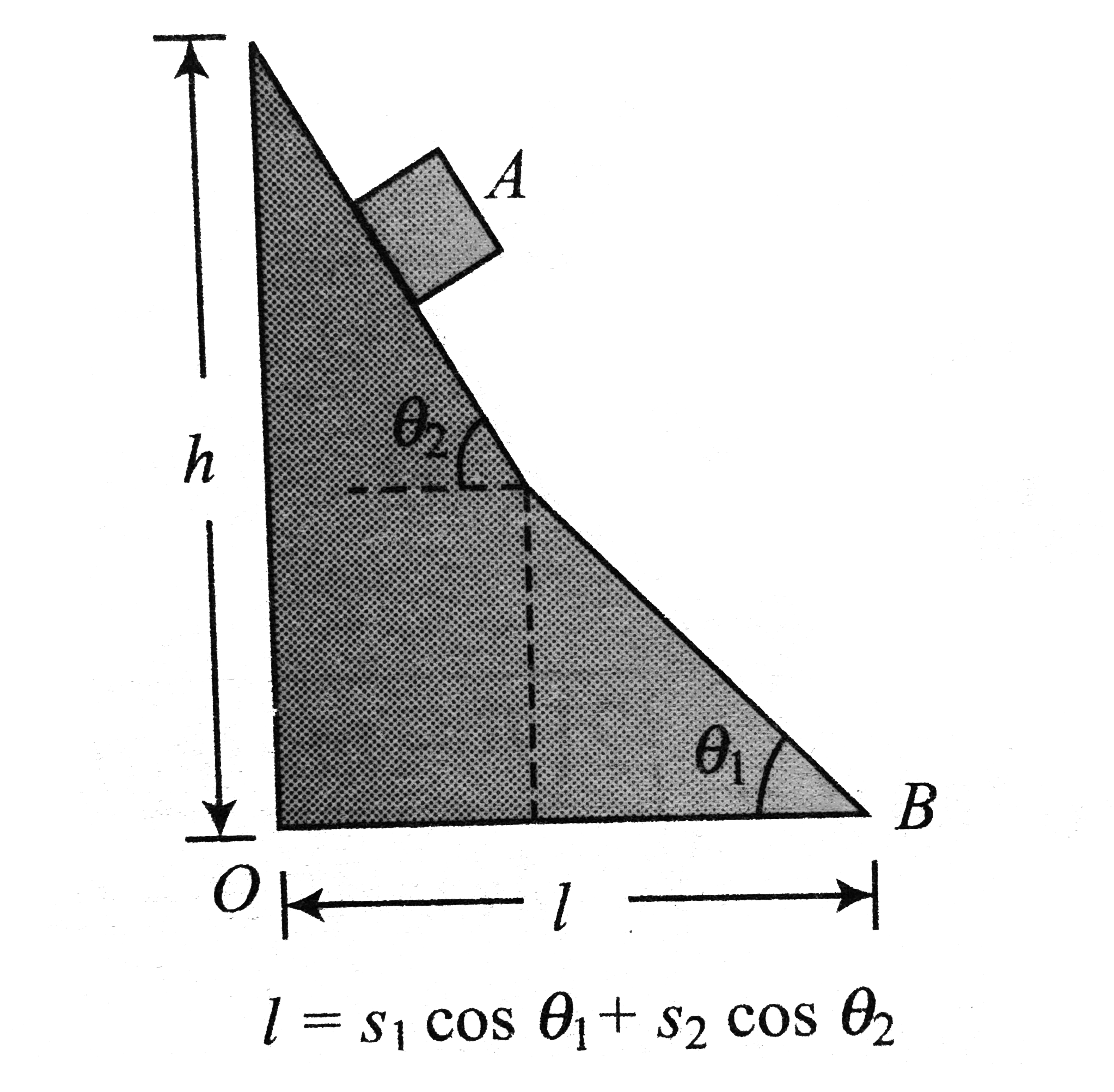
Wfk=−(μkmgcosθ1)s1−(μkmgcosθ2)s2
=−μkmg(s1cosθ1+s2cosθ2)=−μkmgl
For Figure (c)
Let the length of the inclines with inclination θ1, θ2, and θ3 be s1, s2, and s3, respectilvey. At these planes, the frictional force on the block are μkmgcosθ1, μkmgcosθ2, and μkmgcosθ3, respectively. Work done by the frictional force on the block,
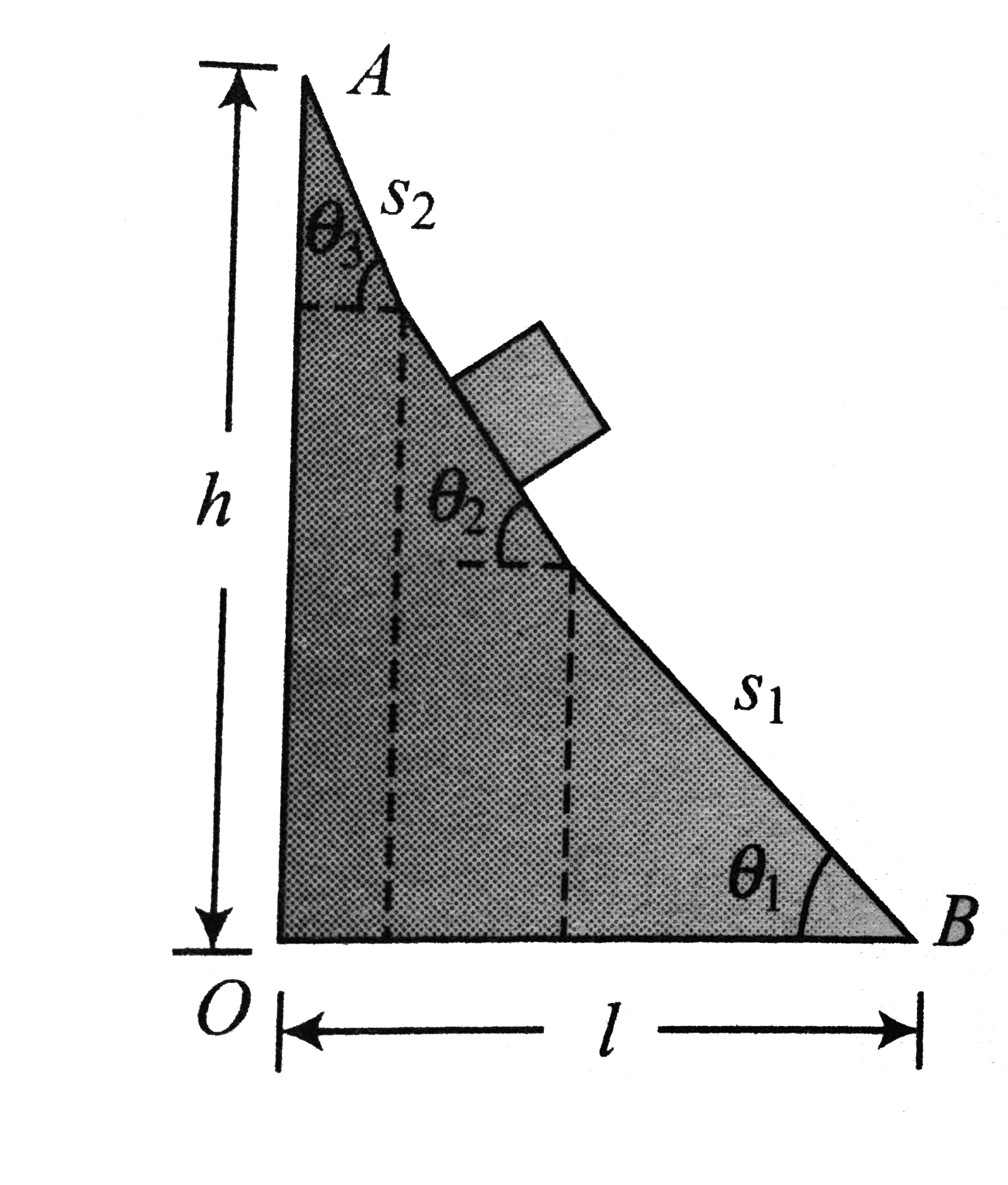
Wfk=−(μkmgcosθ1)s1−(μkmgcosθ2)s2−(μkmgcosθ3)s
=−μkmg(s1cosθ1+s2cosθ2+s3θ3)=−μkmgl.
For Figure (d)
Let the block be at point C where the tangent to the parabola makes an angle θ with the horizontal as shown in figure.
Frictional force on the block at
C=μkmgcosθ, as shown. Consider an infinitesimal displacement ds at C.
Work done by the frictional force over ds,

dW=μ1mgcosθds
Work done from A to B,
Wfk=∫BAdW∫BA−μkmgcosθds
=−∫BAμkmgdx=−μkmgl
Calculations show that the work done by the frictional force in each case are same.
Speed at B:
Using work-energy theorem.
Wmg=Wfk=K2−K1
⇒mgh−mkmgl=12mv2=0
(Block is at rest at A, K1=0)
⇒2g(h−mkl)=v2⇒v=√2g(h−μkl) 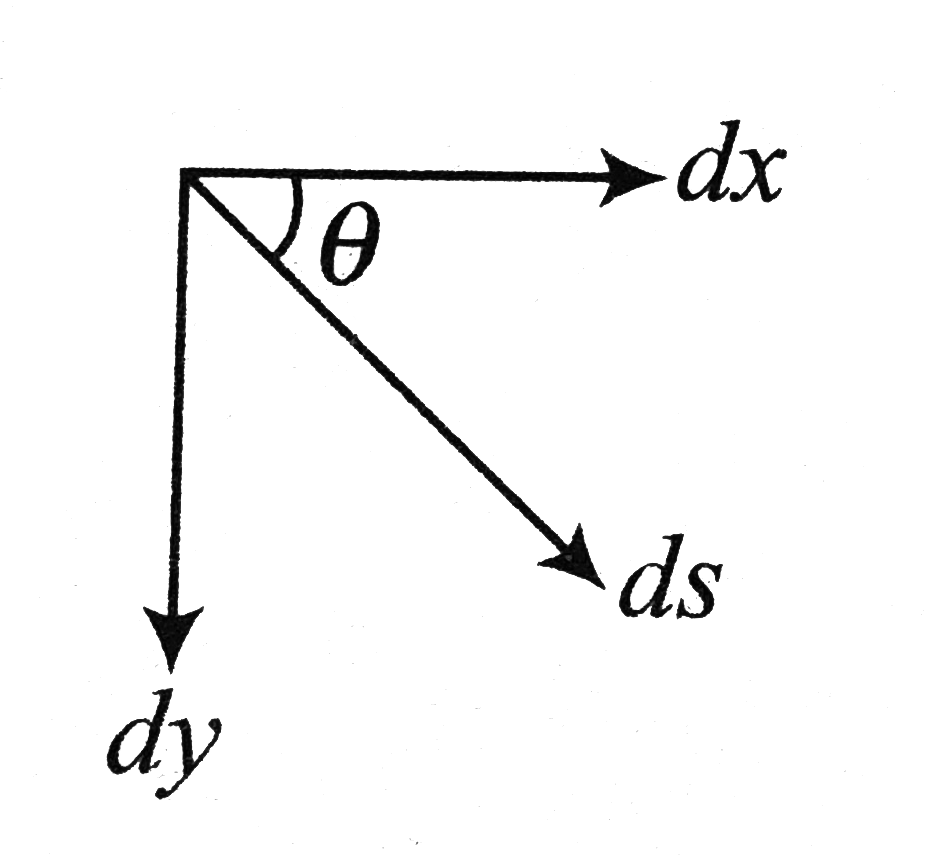
In each case, the block will reach B with same speed, which equals √2g(h−μkl).
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.2
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A block of mass m slides down on inclined wedge of same mass m as shown in figure . Friction is absent everywhere . Acceleration of centre of mass of the block and wedge is
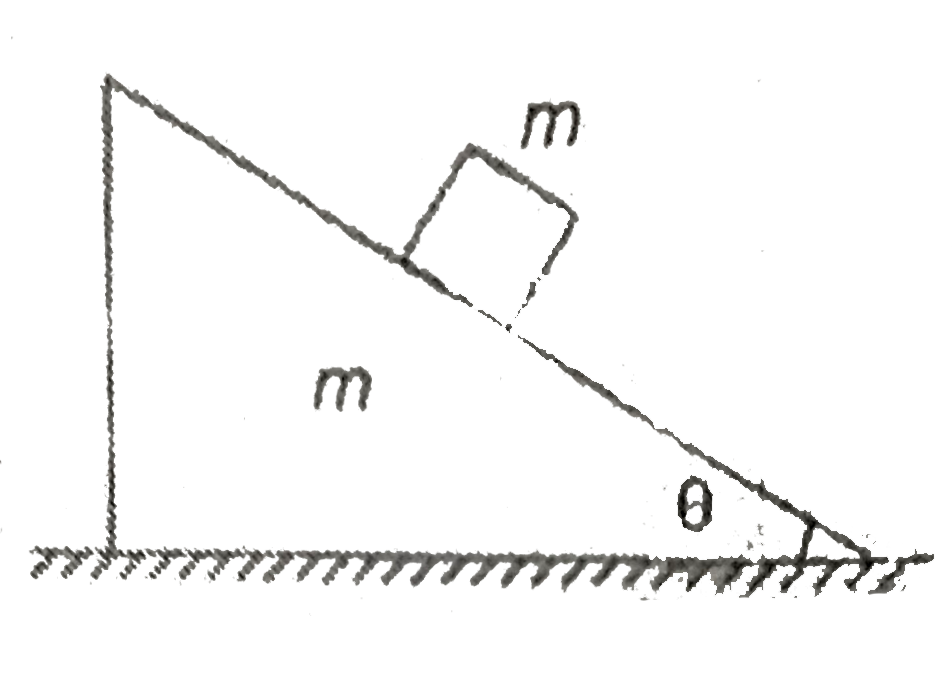
View SolutionA block of mass m slides down on inclined wedge of same mass m as shown in figure . Friction is absent everywhere . Acceleration of centre of mass of the block and wedge is

View SolutionA block of mass m is placed on the inclined sufrace of a wedge as shown in fig. Calculate the acceleration of the wedge and the block when the block is released. Assume all surfaces are frictionless.

View SolutionA block of mass 2kg is released from rest on a rough inclined ground as shown in figure. Find the work done on the block by a) gravity b) force of friction .when the block is displaced downward along the plane by 2m ?
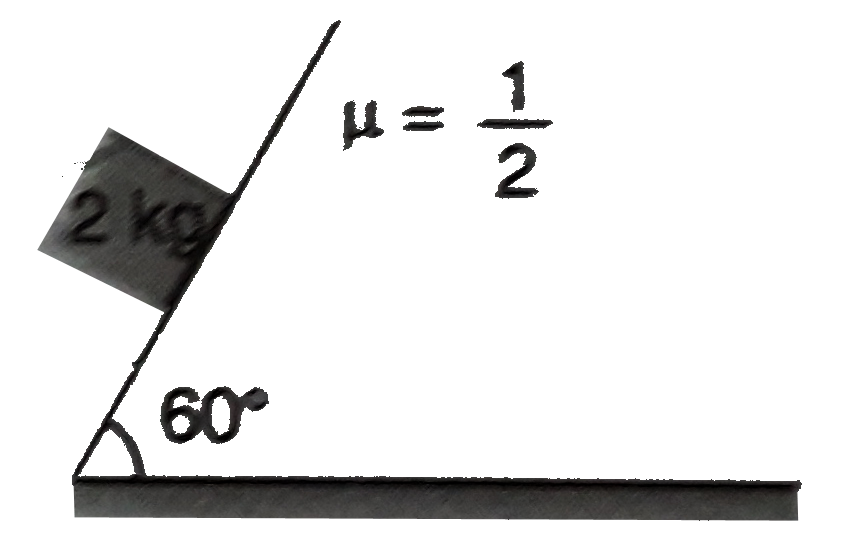 .
.
(Take g10m/s2).
View SolutionA small blockofmass 'm' is released from rest at the top of a rough inclined plane as shown The coefficient of friction between the block and inclined plane is 'μ' Using work-energy theorem, find the speed of block as it passes the lowest point.
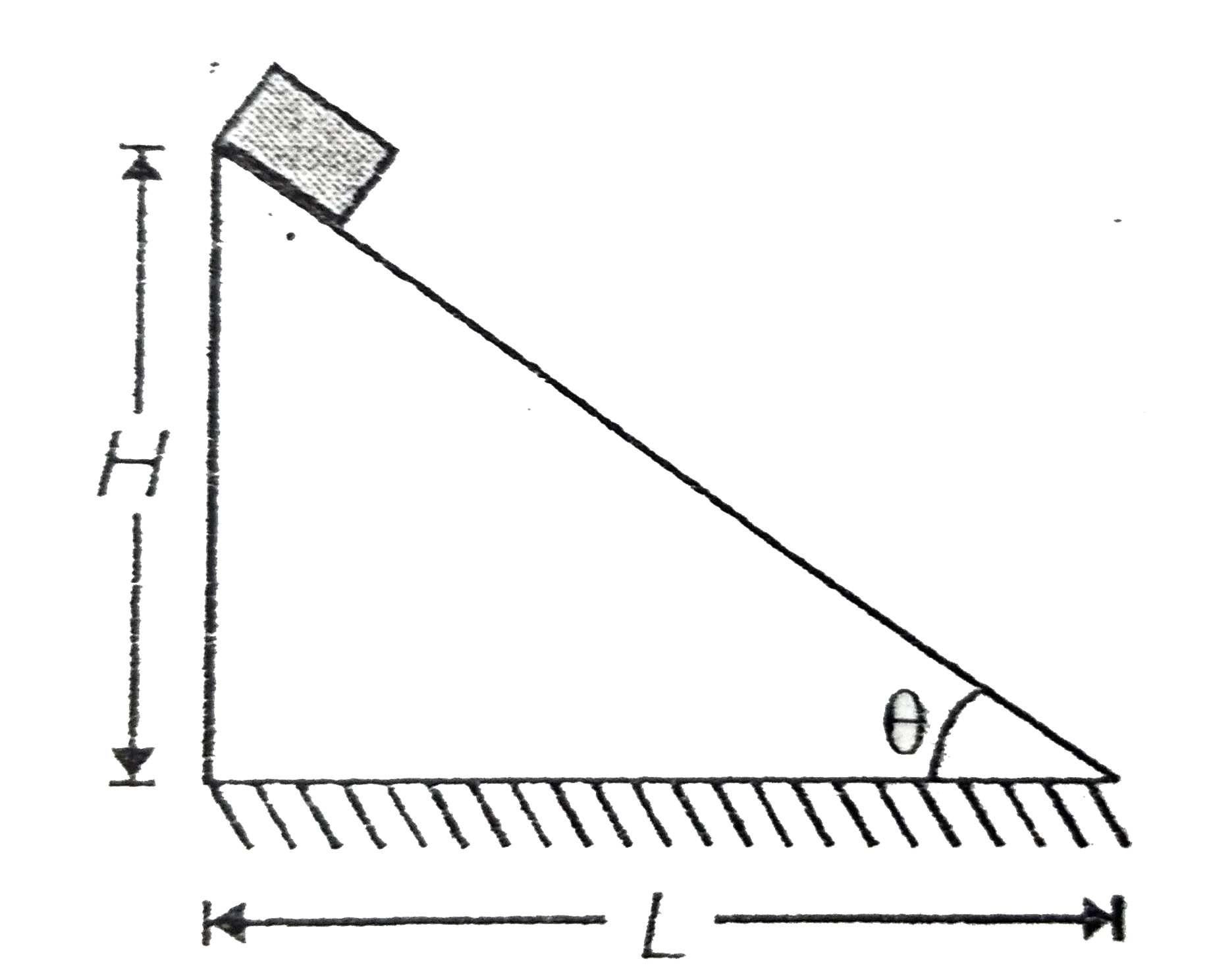
View Solution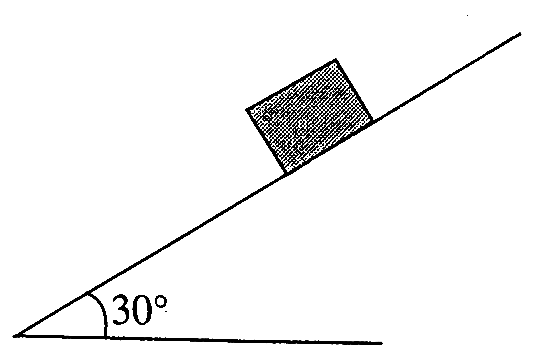
Figure shows a block kept on a rough inclined plane. The maximum external force down the incline for which the block remains at rest is 1 N while the maximum external force up the incline for which the block is at rest is 7 N. The coefficient of static friction μ is:
View SolutionA block of mass 250 g slides down an incline of inclination 370 with a uniform speed. Find the work done against the friction as the block slides through 1.0 m.
View SolutionA block of mass m is released on the top of a smooth inclined plane of length x and inclination θ as shown in figure. Horizontal surface is rough. If block comes to rest after moving a distabce d on the horizontal surface, then coefficient of friction between block and surface is :-
View SolutionA block of mass 4kg is released from the top of a rough inclines plane of height 10m. If velocity of the block is10m/s at the bottom of the inclined then work done by the friction force on the block will be
View SolutionA block of mass m slides along a frictionless surface as shown in the figure. If it is releases from rest from A what is its speed at B?
View Solution
