A man is standing on a rail road car travelling with a constant speed of v=10ms−1 (Fig . 5.34). He wishes to throw a ball through a stationary hoop 5m above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of 12.5ms−1 w.r.t. himself.
(a) What must be the vertical component of the initial velocity of the ball ?
(b) How many seconds after he releases the ball will it pass through the hoop ?
( c) At what horizontal distance in front of the loop must he release the ball ?
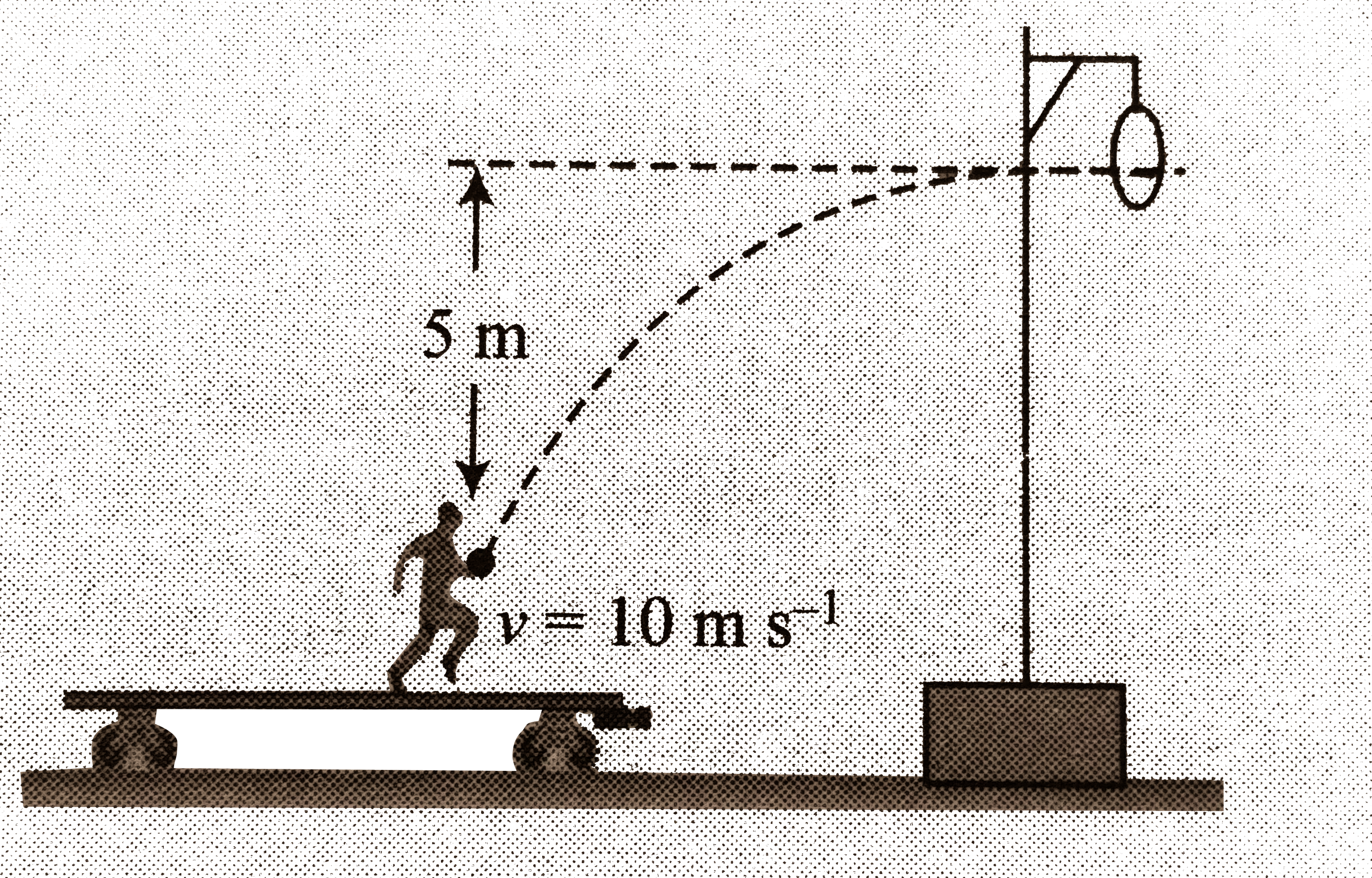 .
.
The important aspects to be noticed in this problem are :
The velocity of the projection of ball is relative to man in motion.
The ball clears the hoop when it is at the topmost point.
→vball,man=→vball−→vman
→vball=→vball,man+→vman
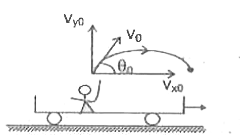
(a) Now we apply the above relation to x - as well as y - component of velocity. If the ball is projected with velocity v0and∠θ, then
x - component of →vball=(v0cosθ+10)ms−1
y - component of →vball=(v0sinθ)ms−1
(b) Since the vertical component of the ball's velocity is unaffected by the horizontal motion of car, we can use the formula for the time of flight.
(12.5sinθ)22g=5m
sin2θ=5×(2×10)12.5×12.5
sinθ=45andcosθ=35
v0sinθ=(12.5)×(45)=10ms−1
Time taken to reach the maximum height,
2v0sinθg=2×1010=2s
( c) The horizontal distance of loop from the point of projection =(12.5cosθ+10)×1=17.5m.
Topper's Solved these Questions
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Solved Examples
Explore 7 Videos
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Exercise 5.1
Explore 15 Videos
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Integer
Explore 9 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
Similar Questions
A boy is standing inside a train moving with a constant velocity of magnitude 10 m/s. He throws a ball vertically up with a speed 5 m/s relative to the train. Find the radius of curvature of the path of the L ball just at the time of projection.
View SolutionA player throws a ball upwards with an initial speed of 29.4ms−1. What are the velcity and acceleration of the hall at the highest point os its motion ?
View SolutionA person is standing on a truck moving with a constant velocity of 14.7 m/s o a hrozontal road. The man throws a ball in such a way that it returns to the truck after the truck has moved 58.8 m. Find the speed and the angle of projection. a. as seen from the truck b. as seen fromt the road.
View SolutionA car expends an energy of 25,000 J while moving at a constant speed of 5 ms−1. What is the mass of car ?
View SolutionThe ceiling of a tunnel is 5 m high. What is the maximum horizontal distance that a ball thrown with a speed of 20ms−1 can go without hitting the ceiling of the tunnel (g=10ms−2)
View SolutionA boy throws a ball in air in such a manner that when the ball is at its maximum height he throws another ball. If the balls are thrown with the time difference 1 second, the maximum height attained by each ball is
View SolutionA cricket fielder can throw the cricket ball with a speed v0. If the throws the ball while running with speed u at an angle θ to the horizontal,
View SolutionA person can throw a ball vertically upto maximum height of 20 mt. How far can he throw the ball.
View SolutionA boy standing on the top of a tower 36 m high has a throw a packet to his friend standing on the ground 48 m horizontally away. If he throws a packet directly aiming the friend with a speed of 10ms−1, how short will be packet fall ?
View SolutionA boy throws a ball at an angle θ with the vertical. If the vertical component of the initial velocity is 20ms−1 and the wind imparts a horizontal acceleration of 8ms−2 to the left, the angle at which the ball must be thrown so that the ball returns to the boy's hand is θ. What is the value of 10(tanθ)
View Solution