A boy standing on the top of a tower 36 m high has a throw a packet to his friend standing on the ground 48 m horizontally away. If he throws a packet directly aiming the friend with a speed of 10ms−1, how short will be packet fall ?
To solve the problem step-by-step, we will analyze the motion of the packet thrown by the boy from the top of the tower.
Step 1: Understand the Problem
A boy is standing on top of a tower that is 36 meters high. He throws a packet horizontally towards his friend who is 48 meters away. The packet is thrown with a speed of 10 m/s. We need to find out how short the packet will fall from the friend's position.
Step 2: Determine the Angle of Projection
The height of the tower (h) is 36 m, and the horizontal distance (d) to the friend is 48 m. We can find the angle of projection (θ) using the tangent function:
tan(θ)=heightdistance=3648=34
From this, we can find the sine and cosine of θ:
sin(θ)=35,cos(θ)=45
Step 3: Calculate the Horizontal and Vertical Components of Velocity
The initial speed of the packet is 10 m/s. We can find the horizontal (Vx) and vertical (Vy) components of the velocity:
Vx=V⋅cos(θ)=10⋅45=8m/s
Vy=V⋅sin(θ)=10⋅35=6m/s
Step 4: Calculate the Time of Flight
We will use the vertical motion to find the time taken for the packet to reach the ground. The vertical motion can be described by the equation:
s=ut+12at2
Where:
- s=36m (height of the tower)
- u=Vy=6m/s (initial vertical velocity)
- a=g=10m/s2 (acceleration due to gravity)
Substituting the values:
36=6t+12⋅10⋅t2
This simplifies to:
36=6t+5t2
Rearranging gives us:
5t2+6t−36=0
Step 5: Solve the Quadratic Equation
We can use the quadratic formula t=−b±√b2−4ac2a to solve for t:
- a=5
- b=6
- c=−36
Calculating the discriminant:
b2−4ac=62−4⋅5⋅(−36)=36+720=756
Now substituting into the quadratic formula:
t=−6±√75610
Calculating √756≈27.5:
t=−6+27.510≈2.15s
Step 6: Calculate the Horizontal Distance Covered
Now we can calculate the horizontal distance the packet travels during this time:
Horizontal distance=Vx⋅t=8⋅2.15≈17.2m
Step 7: Determine How Short the Packet Falls
The packet was aimed at a friend who is 48 m away. The distance it actually traveled horizontally is 17.2 m. Therefore, the distance it falls short is:
Shortfall=48−17.2=30.8m
Final Answer
The packet will fall 30.8 meters short of reaching the friend.
---
A boy is standing on top of a tower that is 36 meters high. He throws a packet horizontally towards his friend who is 48 meters away. The packet is thrown with a speed of 10 m/s. We need to find out how short the packet will fall from the friend's position.
The height of the tower (h) is 36 m, and the horizontal distance (d) to the friend is 48 m. We can find the angle of projection (θ) using the tangent function:
tan(θ)=heightdistance=3648=34
From this, we can find the sine and cosine of θ:
sin(θ)=35,cos(θ)=45
The initial speed of the packet is 10 m/s. We can find the horizontal (Vx) and vertical (Vy) components of the velocity:
Vx=V⋅cos(θ)=10⋅45=8m/s
Vy=V⋅sin(θ)=10⋅35=6m/s
We will use the vertical motion to find the time taken for the packet to reach the ground. The vertical motion can be described by the equation:
s=ut+12at2
Where:
- s=36m (height of the tower)
- u=Vy=6m/s (initial vertical velocity)
- a=g=10m/s2 (acceleration due to gravity)
36=6t+12⋅10⋅t2
This simplifies to:
36=6t+5t2
Rearranging gives us:
5t2+6t−36=0
We can use the quadratic formula t=−b±√b2−4ac2a to solve for t:
- a=5
- b=6
- c=−36
b2−4ac=62−4⋅5⋅(−36)=36+720=756
Now substituting into the quadratic formula:
t=−6±√75610
Calculating √756≈27.5:
t=−6+27.510≈2.15s
Now we can calculate the horizontal distance the packet travels during this time:
Horizontal distance=Vx⋅t=8⋅2.15≈17.2m
The packet was aimed at a friend who is 48 m away. The distance it actually traveled horizontally is 17.2 m. Therefore, the distance it falls short is:
Shortfall=48−17.2=30.8m
The packet will fall 30.8 meters short of reaching the friend.
Topper's Solved these Questions
- MOTIONBook:DC PANDEY ENGLISHChapter:MOTIONExercise:Check Point 4.1
Explore 15 Videos
- MOTIONBook:DC PANDEY ENGLISHChapter:MOTIONExercise:Check Point 4.2
Explore 20 Videos
- MEASUREMENT AND ERRORSBook:DC PANDEY ENGLISHChapter:MEASUREMENT AND ERRORSExercise:Subjective
Explore 19 Videos
- MOTION IN A PLANEBook:DC PANDEY ENGLISHChapter:MOTION IN A PLANEExercise:(C )Medical entrances gallery
Explore 32 Videos
Similar Questions
A person standing on the top of a cliff 171 ft high has to throw a packet to his friend standing on the ground 228 ft horizontally away. If he throws the packet directly aiming at the friend with a speed o 15.0 ft/s, how short will the packet fall?
View SolutionA boy standing on the top of a tower of height 54 ft. throws a packet with a speed of 20 ft/s directly aiming towards his friend standing on the ground at a distance of 72 ft from the foot of the tower. The packet falls short of the person on the ground by x×163 ft. The value of x is
View SolutionA boy standing at the top of a tower of 20m of height drops a stone. Assuming g=10ms−2, the velocity with which it hits the ground is :-
View SolutionA boy standing at the top of a tower of 20m. Height drops a stone. Assuming g=10ms−2 towards north. The average acceleration of the body is.
View SolutionA bullet is projected upwards from the top of a tower of height 90 m with the velocity 30ms−1 making an angle 30∘ with the horizontal. Find the time taken by it to reach the ground is (g=10ms−2)
View SolutionA hose pipe lying on the ground shoots a stream of water upward at an angle 60∘ to the horizontal at a speed of 20ms−1. The water strikes a wall 20m away at a height of (g=10ms2)
View SolutionA man throws a packet from a tower directly aiming at his friend who is standing at a certain distance from the base which is same as the height of the tower. If the packet is thrown with a speed of 4ms−1 and it hits the ground midway between the tower base & his friend than the height of the tower is (g=10ms−2)
View SolutionA boy playing on the roof of a 10 m high building throws a ball with a speed of 10 m/s at an angle 30∘ with the horizontal. How far from the throwing point will the ball be at the height of 10 m from the ground?
(g=10m/s2)
View SolutionA ball is thrown from the top of 36m high tower with velocity 5m/ss at an angle 37∘ above the horizontal as shown. Its horizontal distance on the ground is closest to [g=10m/s2
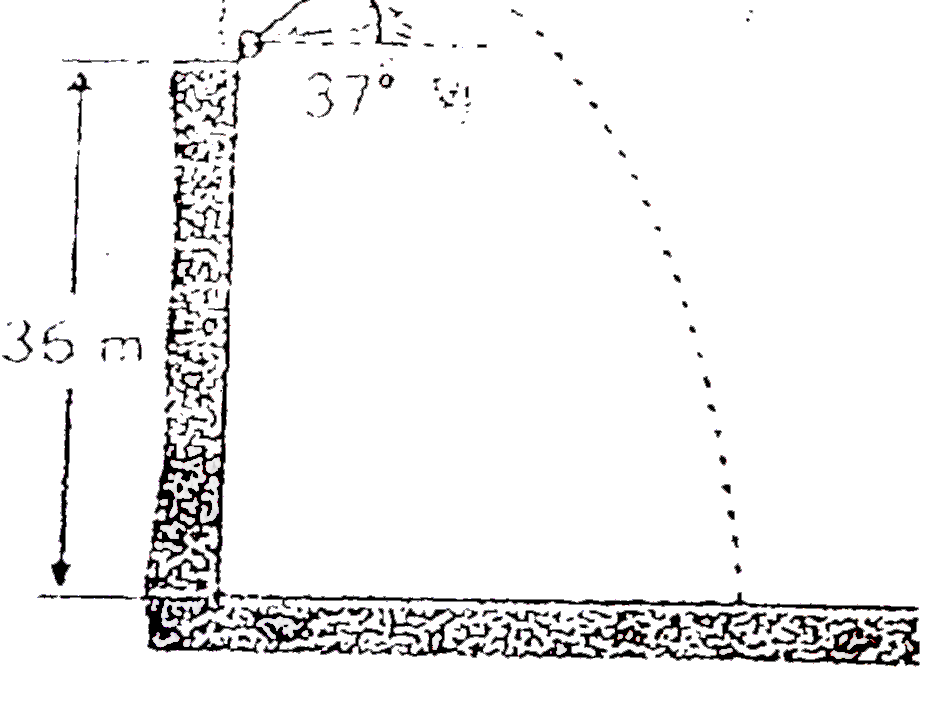 :
:
View SolutionA man is standing on a rail road car travelling with a constant speed of v=10ms−1 (Fig . 5.34). He wishes to throw a ball through a stationary hoop 5m above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of 12.5ms−1 w.r.t. himself.
(a) What must be the vertical component of the initial velocity of the ball ?
(b) How many seconds after he releases the ball will it pass through the hoop ?
( c) At what horizontal distance in front of the loop must he release the ball ?
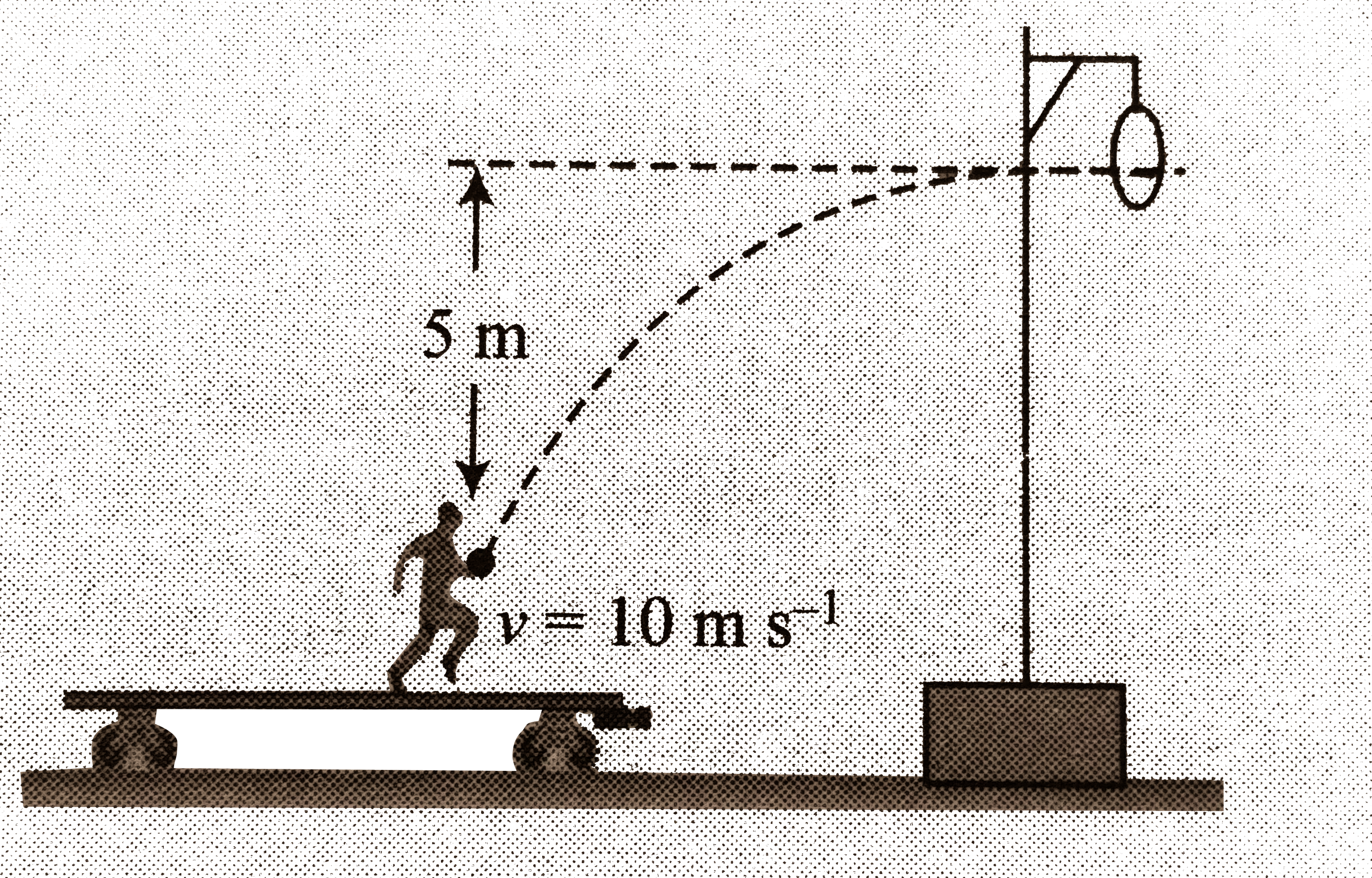 .
.
View Solution