A person is standing on a truck moving with a constant velocity of 14.7 m/s o a hrozontal road. The man throws a ball in such a way that it returns to the truck after the truck has moved 58.8 m. Find the speed and the angle of projection. a. as seen from the truck b. as seen fromt the road.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will break it down into two parts: finding the speed and angle of projection as seen from the truck and then as seen from the road.
Step 1: Calculate the time taken for the truck to travel 58.8 m
The truck moves with a constant velocity of vt=14.7m/s. The distance traveled by the truck is d=58.8m.
Using the formula for time:
t=dvt
Substituting the values:
t=58.8m14.7m/s=4s
Step 2: Determine the speed of projection as seen from the truck
Since the ball returns to the person’s hand after 4 seconds, it takes 2 seconds to reach the maximum height and another 2 seconds to come back down.
Using the equation of motion:
v=u+at
At the maximum height, the final velocity v=0. The acceleration due to gravity a=−g (taking downward as negative).
Thus, we have:
0=u−g⋅t
For the upward journey, t=2s:
0=u−g⋅2
Rearranging gives:
u=g⋅2
Substituting g≈9.8m/s2:
u=9.8⋅2=19.6m/s
Step 3: Determine the angle of projection as seen from the truck
Since the ball is thrown vertically upward, the angle of projection θ is:
θ=90∘
Step 4: Calculate the speed of projection as seen from the road
To find the speed of the ball as observed from the road, we need to consider the horizontal component of the velocity. The horizontal velocity of the truck is vt=14.7m/s and the vertical velocity of the ball is u=19.6m/s.
Using Pythagoras theorem to find the resultant velocity v:
v=√(vt)2+(u)2
Substituting the values:
v=√(14.7)2+(19.6)2
Calculating:
v=√216.09+384.16=√600.25≈24.5m/s
Step 5: Determine the angle of projection as seen from the road
The angle θ′ can be found using the tangent function:
tan(θ′)=uvt
Substituting the values:
tan(θ′)=19.614.7
Calculating:
θ′=tan−1(19.614.7)≈53.1∘
Final Results
- As seen from the truck:
- Speed of projection u=19.6m/s
- Angle of projection θ=90∘
- As seen from the road:
- Speed of projection v≈24.5m/s
- Angle of projection θ′≈53.1∘
t=dvt
Substituting the values:
t=58.8m14.7m/s=4s
v=u+at
At the maximum height, the final velocity v=0. The acceleration due to gravity a=−g (taking downward as negative).
0=u−g⋅t
For the upward journey, t=2s:
0=u−g⋅2
Rearranging gives:
u=g⋅2
Substituting g≈9.8m/s2:
u=9.8⋅2=19.6m/s
θ=90∘
v=√(vt)2+(u)2
Substituting the values:
v=√(14.7)2+(19.6)2
Calculating:
v=√216.09+384.16=√600.25≈24.5m/s
tan(θ′)=uvt
Substituting the values:
tan(θ′)=19.614.7
Calculating:
θ′=tan−1(19.614.7)≈53.1∘
- Speed of projection u=19.6m/s
- Angle of projection θ=90∘
- Speed of projection v≈24.5m/s
- Angle of projection θ′≈53.1∘
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective -2
9 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer
14 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
Similar Questions
A person is standing on an open car moving with a constant velocity of 30 m/s on a straight horizontal road.The men throws a ball in the vertically upward direction and it return to the person after the car has moved 240 m. The speed and angel of projection
In the previous problem if the car moving with a constant acceleration of 2 m/s2 , the ball will fall behind the person at a distance
A. 32m
B. 64m
C. 96m
D. 16m
View SolutionA car is moving horizontally along a straight line with a unifrom velocity of 25ms−1. A projectile is to be fired from this car in such a way that it will return to it after it has moved 100m. The speed of the projection must be.
View SolutionA boy is standing inside a train moving with a constant velocity of magnitude 10 m/s. He throws a ball vertically up with a speed 5 m/s relative to the train. Find the radius of curvature of the path of the L ball just at the time of projection.
View SolutionA cart is moving horizontally along a straight line with constant speed 30 m/s. A projectile is to be fired from the moving cart in such a way that it will return to the cart after the cart has moved 120m. At what speed (relative to the cart, in m/s) must the projectile be fired ? (Take g=10m/s2)
View SolutionA cart is moving horizontally along a straight line with constant speed 30ms−1. A particle is to be fired vertically upwards from the moving cart in such a way that it returns to the cart at the same point from where it was projected after the cart has moved 80m. At what speed (relative to the cart) must the projectile be fired? (Take g=10ms−2)
View SolutionA cart is moving horizontally along a straight line with constant speed 30ms−1. A particle is to be fired vertically upwards from the moving cart in such a way that it returns to the cart at the same point from where it was projected after the cart has moved 80m. At what speed (relative to the cart) must the projectile be fired? (Take g=10ms−2)
View SolutionAn automobile and a truck start from rest at the same instant, with the automobile initially at some distance behind the truck. The truck has a constant acceleration of 2.2m/s2 and the automobile has an acceleration of 3.5m/s2. The automobile overtakes the truck when it (truck) has moved 60 m.
(a) How much time does it take the automobile to overtake the truck ?
(b) How far was the automobile behind the truck initially ?
(c) What is the speed of each during overtaking ?
View SolutionA man is standing on a rail road car travelling with a constant speed of v=10ms−1 (Fig . 5.34). He wishes to throw a ball through a stationary hoop 5m above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of 12.5ms−1 w.r.t. himself.
(a) What must be the vertical component of the initial velocity of the ball ?
(b) How many seconds after he releases the ball will it pass through the hoop ?
( c) At what horizontal distance in front of the loop must he release the ball ?
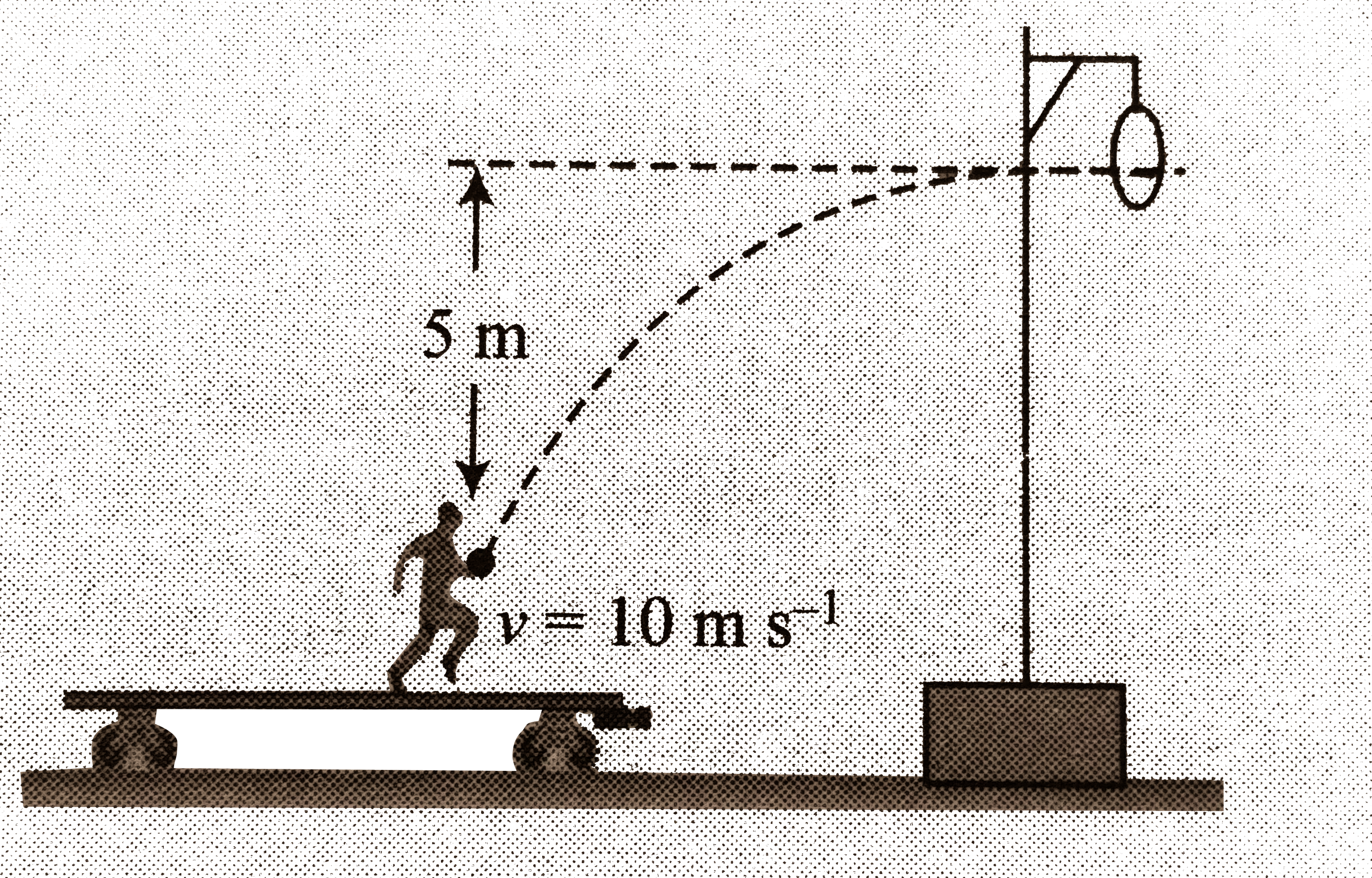 .
.
View SolutionA truck is moving with a constant velocity of 54kmh−1. In which direction (angle with the direction of motion of truck) should a stone be projected up with a velocity of 20ms−1, from the floor of the truck of the truck, so as to appear at right angles to the truck, for a person standing on earth ?
View SolutionA truck is moving with a constant velocity of 54kmh−1. In which direction (angle with the direction of motion of truck) should a stone be projected up with a velocity of 20ms−1, from the floor of the truck of the truck, so as to appear at right angles to the truck, for a person standing on earth ?
View Solution
