Question

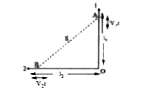
Two particles, 1 and 2, move with constant velocities ${\displaystyle v_1}$ and ${\displaystyle v_2}$ along two mutually perpendicular straight lines toward the intersection point O. At the moment ${\displaystyle t=0}$ the particles were located at the distances ${\displaystyle l_1}$ and ${\displaystyle l_2}$ from the point O. How soon will the distance between the particles become the smallest? What is it equal to?

Explanation

To solve the problem step by step, we need to analyze the motion of the two particles and find the time at which the distance between them is minimized, as well as the value of that minimum distance.

Step 1: Understand the setup
We have two particles moving towards a common point O along two perpendicular lines. Particle 1 starts at a distance \( l_1 \) from O and moves with velocity \( v_1 \). Particle 2 starts at a distance \( l_2 \) from O and moves with velocity \( v_2 \).

Step 2: Define the positions of the particles
At time \( t \):
- The position of Particle 1 from point O is \( l_1 - v_1 t \).
- The position of Particle 2 from point O is \( l_2 - v_2 t \).

Step 3: Use the Pythagorean theorem to find the distance
The distance \( x \) between the two particles at time \( t \) can be expressed using the Pythagorean theorem:
\( x(t) = \sqrt{(l_1 - v_1 t)^2 + (l_2 - v_2 t)^2} \)

Step 4: Differentiate to find the minimum distance
To find the time \( t \) when the distance \( x(t) \) is minimized, we need to differentiate \( x(t) \) with respect to \( t \) and set the derivative equal to zero:
\( \frac{dx}{dt} = \frac{1}{2} \cdot \frac{1}{\sqrt{(l_1 - v_1 t)^2 + (l_2 - v_2 t)^2}} \cdot 2 \left( (l_1 - v_1 t)(-v_1) + (l_2 - v_2 t)(-v_2) \right) \)
Setting this equal to zero gives:
\( (l_1 - v_1 t)v_1 + (l_2 - v_2 t)v_2 = 0 \)

Step 5: Solve for \( t \)
Rearranging the equation, we get:
\( l_1 v_1 - v_1^2 t + l_2 v_2 - v_2^2 t = 0 \)
Combining terms:
\( l_1 v_1 + l_2 v_2 = (v_1^2 + v_2^2) t \)
Thus, we can solve for \( t \):
\( t = \frac{l_1 v_1 + l_2 v_2}{v_1^2 + v_2^2} \)

Step 6: Find the minimum distance
Now, we substitute this value of \( t \) back into the equation for \( x(t) \) to find the minimum distance:
\( x_{\text{min}} = \sqrt{(l_1 - v_1 t)^2 + (l_2 - v_2 t)^2} \)
Substituting \( t \):
\( x_{\text{min}} = \sqrt{\left(l_1 - v_1 \left(\frac{l_1 v_1 + l_2 v_2}{v_1^2 + v_2^2}\right)\right)^2 + \left(l_2 - v_2 \left(\frac{l_1 v_1 + l_2 v_2}{v_1^2 + v_2^2}\right)\right)^2} \)
After simplification, we find:
\( x_{\text{min}} = \frac{l_1 v_2 - l_2 v_1}{\sqrt{v_1^2 + v_2^2}} \)

Final Answers
1. The time at which the distance between the particles is minimized:
\( t = \frac{l_1 v_1 + l_2 v_2}{v_1^2 + v_2^2} \)
2. The minimum distance between the particles:
\( x_{\text{min}} = \frac{l_1 v_2 - l_2 v_1}{\sqrt{v_1^2 + v_2^2}} \)