It has been observed that velocity of ripple waves produced in water (ρ) , and surface tension (T) . Prove that V2∝T/λρ.
The correct Answer is:v2∝Tλρ
To prove that the velocity of ripple waves produced in water V2 is proportional to Tλρ, we will follow these steps:
Step 1: Identify the dimensions of the variables involved
1. Wavelength (λ): This is a measure of length, so its dimension is:
(λ)=L
2. Density (ρ): Density is mass per unit volume. Therefore, its dimension is:
(ρ)=ML3
3. Surface Tension (T): Surface tension is defined as force per unit length. The dimension of force is (F)=MLT−2. Thus, the dimension of surface tension is:
(T)=FL=MLT−2L=MLT2
4. Velocity (V): The dimension of velocity is:
(V)=LT−1
Step 2: Set up the relationship
Assume that the velocity V can be expressed as a function of λ, ρ, and T:
V=KλaρbTc
where K is a dimensionless constant and a, b, and c are the powers to be determined.
Step 3: Write the dimensions of both sides
The dimensions of the left-hand side (velocity) are:
(LT−1)
The dimensions of the right-hand side can be expressed as:
(λa)=La,(ρb)=(ML3)b=MbL−3b,(Tc)=(MLT2)c=McL−cT−2c
Combining these, we have:
(RHS)=LaMbL−3bMcL−cT−2c=Mb+cLa−3b−cT−2c
Step 4: Equate dimensions
Now, we equate the dimensions from both sides:
1. For mass:
b+c=0(1)
2. For length:
a−3b−c=1(2)
3. For time:
−2c=−1(3)
Step 5: Solve the equations
From equation (3):
c=12
Substituting c into equation (1):
b+12=0⟹b=−12
Now substituting b and c into equation (2):
a−3(−12)−12=1⟹a+32−12=1⟹a+1=1⟹a=0
Step 6: Substitute back into the equation
Now we have:
- a=0
- b=−12
- c=12
Thus, we can write:
V=Kλ0ρ−12T12=K√T√ρ
Step 7: Square both sides
Squaring both sides gives:
V2=K2Tρ
Step 8: Relate to wavelength
To express this in terms of λ, we note that:
V2∝Tλρ
Conclusion
Thus, we have shown that:
V2∝Tλρ
1. Wavelength (λ): This is a measure of length, so its dimension is:
(λ)=L
(ρ)=ML3
(T)=FL=MLT−2L=MLT2
(V)=LT−1
Assume that the velocity V can be expressed as a function of λ, ρ, and T:
V=KλaρbTc
where K is a dimensionless constant and a, b, and c are the powers to be determined.
The dimensions of the left-hand side (velocity) are:
(LT−1)
(λa)=La,(ρb)=(ML3)b=MbL−3b,(Tc)=(MLT2)c=McL−cT−2c
(RHS)=LaMbL−3bMcL−cT−2c=Mb+cLa−3b−cT−2c
Now, we equate the dimensions from both sides:
1. For mass:
b+c=0(1)
a−3b−c=1(2)
−2c=−1(3)
From equation (3):
c=12
b+12=0⟹b=−12
a−3(−12)−12=1⟹a+32−12=1⟹a+1=1⟹a=0
Now we have:
- a=0
- b=−12
- c=12
V=Kλ0ρ−12T12=K√T√ρ
Squaring both sides gives:
V2=K2Tρ
To express this in terms of λ, we note that:
V2∝Tλρ
Thus, we have shown that:
V2∝Tλρ
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Single Correct
93 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Multiple Correct
2 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.3
17 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Video
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Video
Similar Questions
Frequency is the function of density (ρ) , length (a) and surface tension (T) . Then its value is
View SolutionThe speed (v) of ripples on the surface of waterdepends on surface tension (σ), density (ρ) and wavelength (λ). The square of speed (v) is proportional to
View SolutionWater is filled up to a height h in a beaker of radius R as shown in the figure. The density of water is ρ, the surface tension of water is T and the atmospheric presure is p0. Consider a vertical section ABCDof the water on one side of this section by water on the other side of this section has magnitude
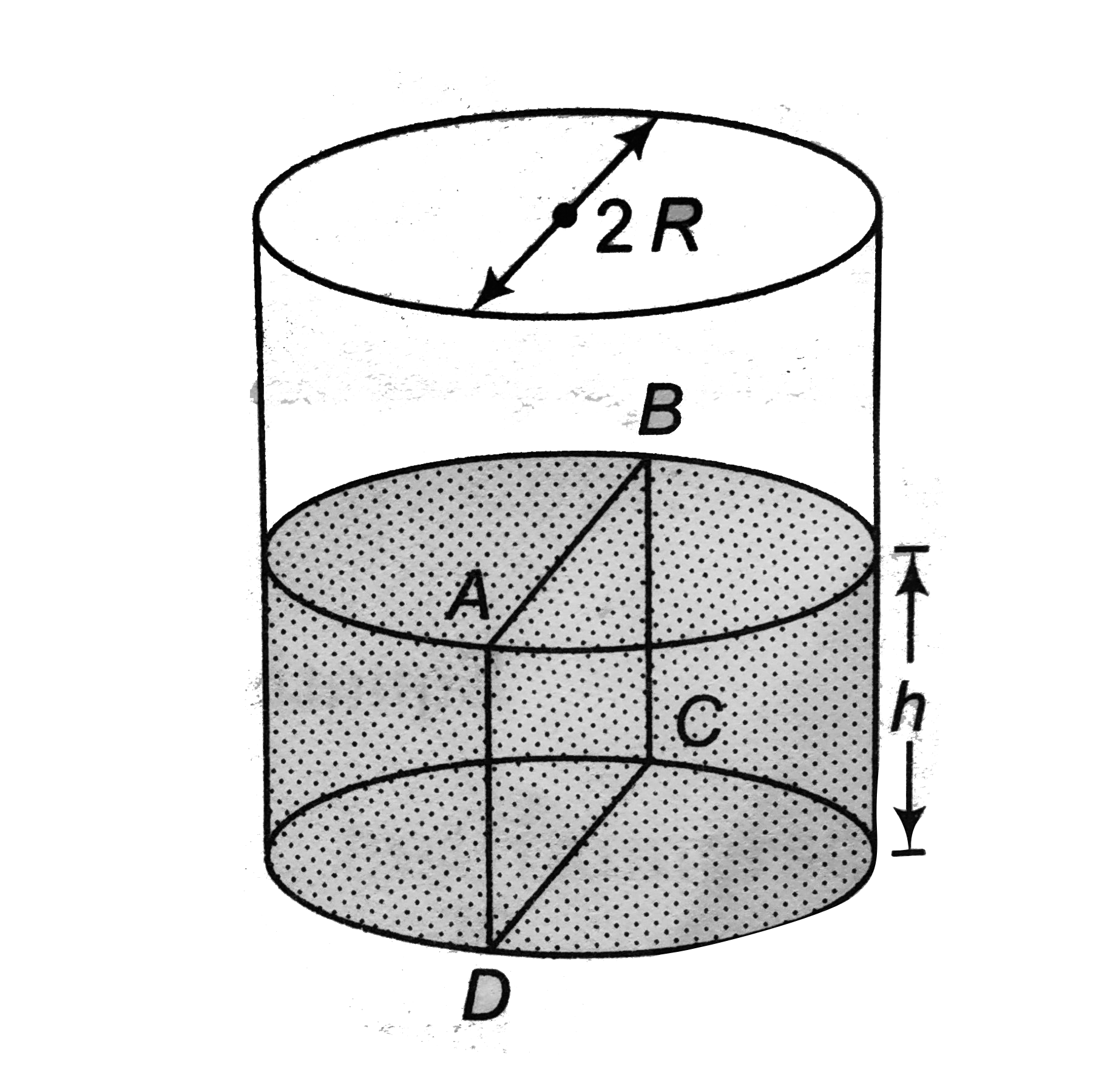
(a) ∣∣2p0Rh+πR2ρgh−2RT∣∣
(b)∣∣2p0Rh+Rρgh2=2RT∣∣
(c ) ∣∣p0(π)R2+Rρgh2−2RT∣∣
(d) ∣∣p0πR2+Rρgh2+2RT∣∣.
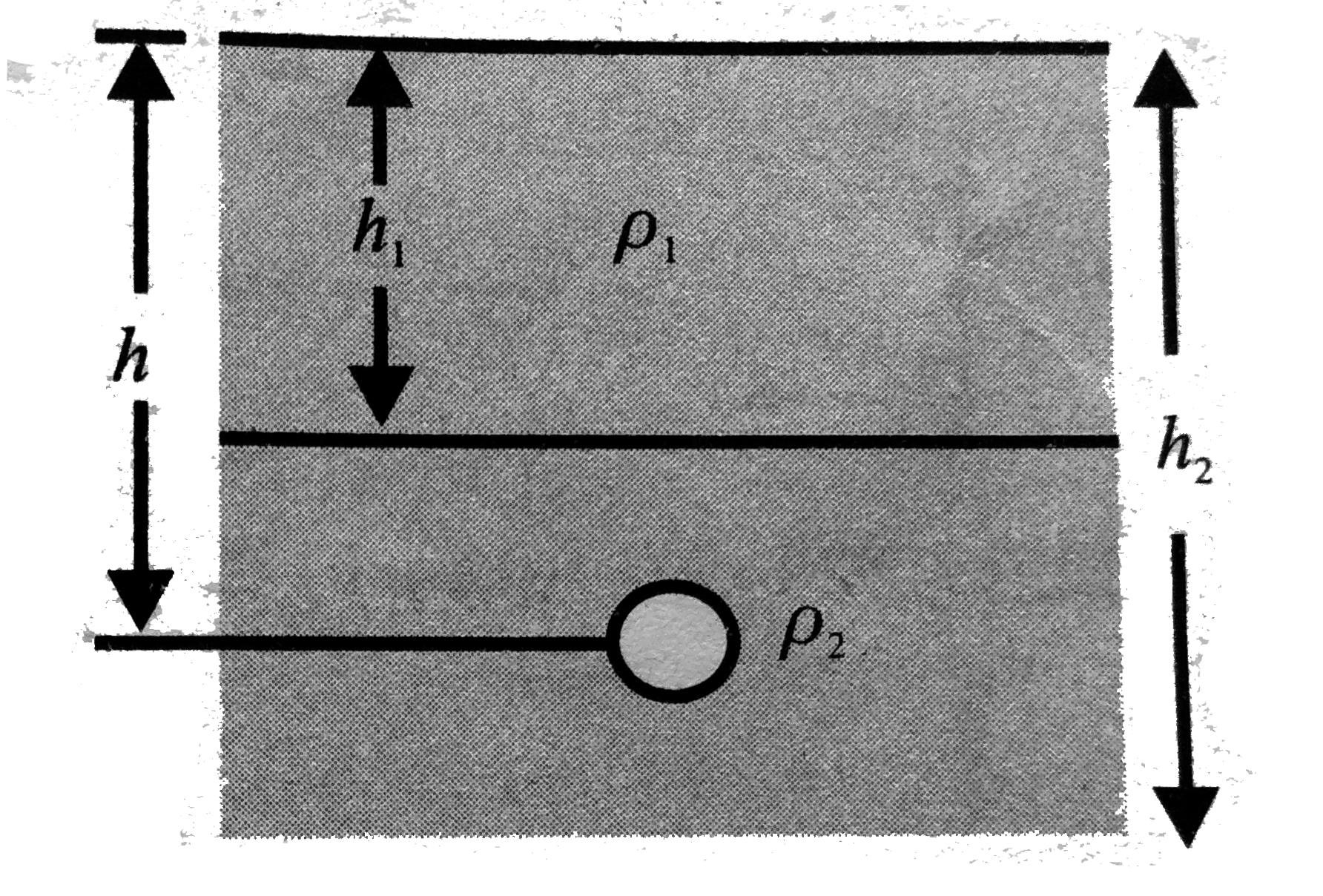
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
View SolutionExperiments reveal that the velocity v of water waves may depend on their wavelength λ, density of water ρ, and acceleration due to gravity g. Establish a possible relation between vandλ,g,ρ.
View SolutionTime period of an oscillating drop of radius r, density ρ and surface tension T is t=k√ρr5T. Check the correctness of the relation
View SolutionThe weight of a body at earth's surface is W. At a depth half way to the centre of earth it will weight
View SolutionA small air bubble of radius 'r' is at a depth 'h' below the water surface (density of water=ρ). Surface tension of water is T, atmospheric pressure is p0. Find pressure inside the air bubble for the condition r<<h
View SolutionA spherical liquid drop is placed on a horizontal plane . A small distrubance cause the volume of the drop to oscillate . The time period oscillation (T) of the liquid drop depends on radius (r) of the drop , density (ρ) and surface tension tension (S) of the liquid. Which amount the following will be be a possible expression for T (where k is a dimensionless constant)?
View SolutionThe dependency of speed of water surface waves (capillary waves) on the density of water (ρ) their wavelength (λ) and surface tension (γ) is -
View Solution
