A capacitor of capacitance C=2.0±0.1μF is charged to a voltage V=20±0.2V. What will be the charge Q on the capacitor ? Use Q=CV.
The correct Answer is:40±2.4×10−6 coulomb
To solve the problem, we will follow these steps:
Step 1: Calculate the charge Q using the formula Q=CV.
Given:
- Capacitance C=2.0μF=2.0×10−6F
- Voltage V=20V
Using the formula:
Q=C×V
Substituting the values:
Q=(2.0×10−6F)×(20V)=40×10−6C=40μC
Step 2: Calculate the uncertainty in charge ΔQ.
To find the uncertainty in the charge, we use the formula for the propagation of uncertainties:
ΔQQ=ΔCC+ΔVV
Where:
- ΔC=0.1μF=0.1×10−6F
- ΔV=0.2V
Now substituting the values:
ΔCC=0.1×10−62.0×10−6=0.12.0=0.05
ΔVV=0.220=0.01
Now, adding these two uncertainties:
ΔQQ=0.05+0.01=0.06
Step 3: Calculate ΔQ.
Now, we can find ΔQ:
ΔQ=Q×ΔQQ=40μC×0.06=2.4μC
Step 4: Write the final result for the charge Q with uncertainty.
Thus, the charge on the capacitor is:
Q=40μC±2.4μC
Final Answer:
The charge on the capacitor is 40μC±2.4μC.
---
- Capacitance C=2.0μF=2.0×10−6F
- Voltage V=20V
Q=C×V
Substituting the values:
Q=(2.0×10−6F)×(20V)=40×10−6C=40μC
ΔQQ=ΔCC+ΔVV
- ΔC=0.1μF=0.1×10−6F
- ΔV=0.2V
ΔCC=0.1×10−62.0×10−6=0.12.0=0.05
ΔVV=0.220=0.01
ΔQQ=0.05+0.01=0.06
ΔQ=Q×ΔQQ=40μC×0.06=2.4μC
Q=40μC±2.4μC
The charge on the capacitor is 40μC±2.4μC.
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Single Correct
93 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Multiple Correct
2 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.3
17 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A capacitor of 0.75μF is charged to a voltage of 16 V. What is the magnitude of the charge on each plate of the capacitor ?
View SolutionA capaitor of capacitance C1=1.0μF withstands teh maximum voltage V1=6.0kV while a capacitor of capacitance Cs=2.0μF, the maximum voltage Vs=4.0kV. What voltage will the system of these two capacitors withsatand if they are connected in sereis ?
View SolutionA capacitor of capacitance 2.0μF is charged to a potential difference of 12 V. It is then connected to an uncharged capacitor of capacitance 4.0μF. Find (a) the charge flow through connecting wire upto study strate on each of the two capacitors after the connection in μC (b) The total electrostatic energy stored in both capacitors in μJ (c) the heat produced during the charge transfer from one capacitor to the other in μJ.
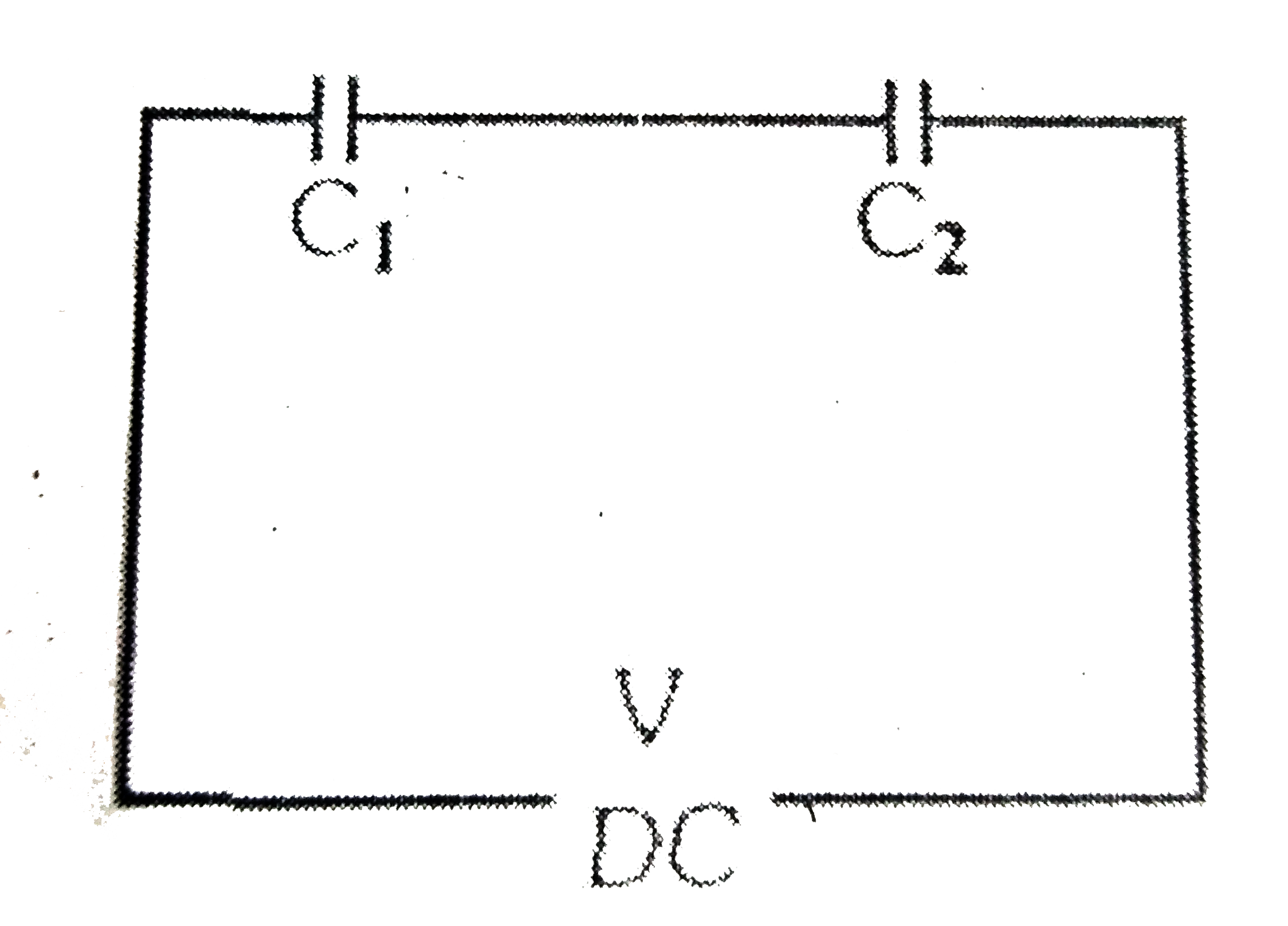
View SolutionA Capacitor of capacitance C1=1μF can withstand a maximum voltage V1=6.0 KV while another capacitor of capacitance C2=2.0μF withstands the maximum voltage V2=4.0 KV . What maximum will the system of these two capacitance withstand when connected in series as shown below .
View SolutionA capacitor of capacitance 10(μ)F is connected to a battery of emf 2V.It is found that it takes 50 ms for the charge on the capacitor to bacome 12.6(μ)C.find the resistance of the circuit.
View SolutionA capacitor of capacitance C1=1.0μF charged upto a voltage V=110V is connected in parallel to the terminals of a circuit consisting of two uncharged capacitors connected in series and possessing the capacitance C2=2.0μF and C3=3.0μF. What charge will flow through the connecting wires?
View SolutionAn ideal capacitor of capacitance 0.2μF is charged to a potential difference of 10V. The charging battery is than disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5mH. The current at a time when the potential difference across the capacitor is 5V is :
View SolutionA capacitor of capacitance C is charged to a potential difference V0. The charged battery is disconnected and the capacitor is connected to a capacitor of unknown capacitance Cx. The potential difference across the combination is V. The value of Cx should be
View SolutionA capacitor of capacitance C0 is charged to potential V0. Now it is connected to another uncharged capacitor of capacitance C02. Calculate the heat loss in this process.
View SolutionA capacitor of capacitance 10μF is charged to a potential 50V with a battery. The battery is now disconnected and an additional charge 200μC is given to the positive plate of the capacitor. The potential difference across the capacitor will be.
View Solution
