The resistance R=VI, where V=(100±5.0)V and I=(10±0.2)A. Find the percentage error in R.
The correct Answer is:7%
To find the percentage error in the resistance R given by the formula R=VI, where V=(100±5.0)V and I=(10±0.2)A, we will follow these steps:
Step 1: Identify the values and uncertainties
- Voltage V=100V with an uncertainty ΔV=5.0V
- Current I=10A with an uncertainty ΔI=0.2A
Step 2: Calculate the relative uncertainties
The relative uncertainty in V is given by:
ΔVV=5.0100=0.05
The relative uncertainty in I is given by:
ΔII=0.210=0.02
Step 3: Use the formula for the relative uncertainty in R
The formula for the relative uncertainty in R when R=VI is:
ΔRR=ΔVV+ΔII
Step 4: Substitute the values
Substituting the relative uncertainties we calculated:
ΔRR=0.05+0.02=0.07
Step 5: Convert to percentage error
To find the percentage error in R, we multiply the relative uncertainty by 100:
Percentage Error=ΔRR×100=0.07×100=7%
Final Answer
The percentage error in the resistance R is 7%.
---
- Voltage V=100V with an uncertainty ΔV=5.0V
- Current I=10A with an uncertainty ΔI=0.2A
The relative uncertainty in V is given by:
ΔVV=5.0100=0.05
ΔII=0.210=0.02
The formula for the relative uncertainty in R when R=VI is:
ΔRR=ΔVV+ΔII
Substituting the relative uncertainties we calculated:
ΔRR=0.05+0.02=0.07
To find the percentage error in R, we multiply the relative uncertainty by 100:
Percentage Error=ΔRR×100=0.07×100=7%
The percentage error in the resistance R is 7%.
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Single Correct
Explore 93 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Multiple Correct
Explore 2 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Exercise 1.3
Explore 17 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:INTEGER_TYPE
Explore 1 Video
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
The resistance R=V/i, where V=100±5VandI=10±0.2A. What is the total error in R?
View SolutionThe resistant R=V/I where V=100±0.5V and I=10±0.2A. The percentage error in V is 5% and in I is 2%. Find the total percentage error is R.
View SolutionIf voltage V=(100±5) V and current I=(10±0.2) A, the percentage error in resistance R is
View SolutionWhen the voltage and current in a conductor are measured as (100±4)Vand(5±0.2) A, then the percentage of error in the calculation of resistance is
View SolutionThe voltage and current in a conductor are measured as (50±2)Vand(5±0.2)A The percentage error in the calculation of resistance is
View SolutionThe resistance of metal is given by V=IR, The voltage in the resistance is V=(8±0.5)V and current in the resistance is I=(2±0.2)A, the value fo resistance with its percentage error is
View SolutionThe value of resistance of an unknown resistor is calculated using the formula R=V/I where V and I are the readings of the voltmeter and the ammeter, respectivley. Consider the circuits below. The internal resistances of the voltmeter and the ammeter (RVandRG,respectively) are finite and nonzero.
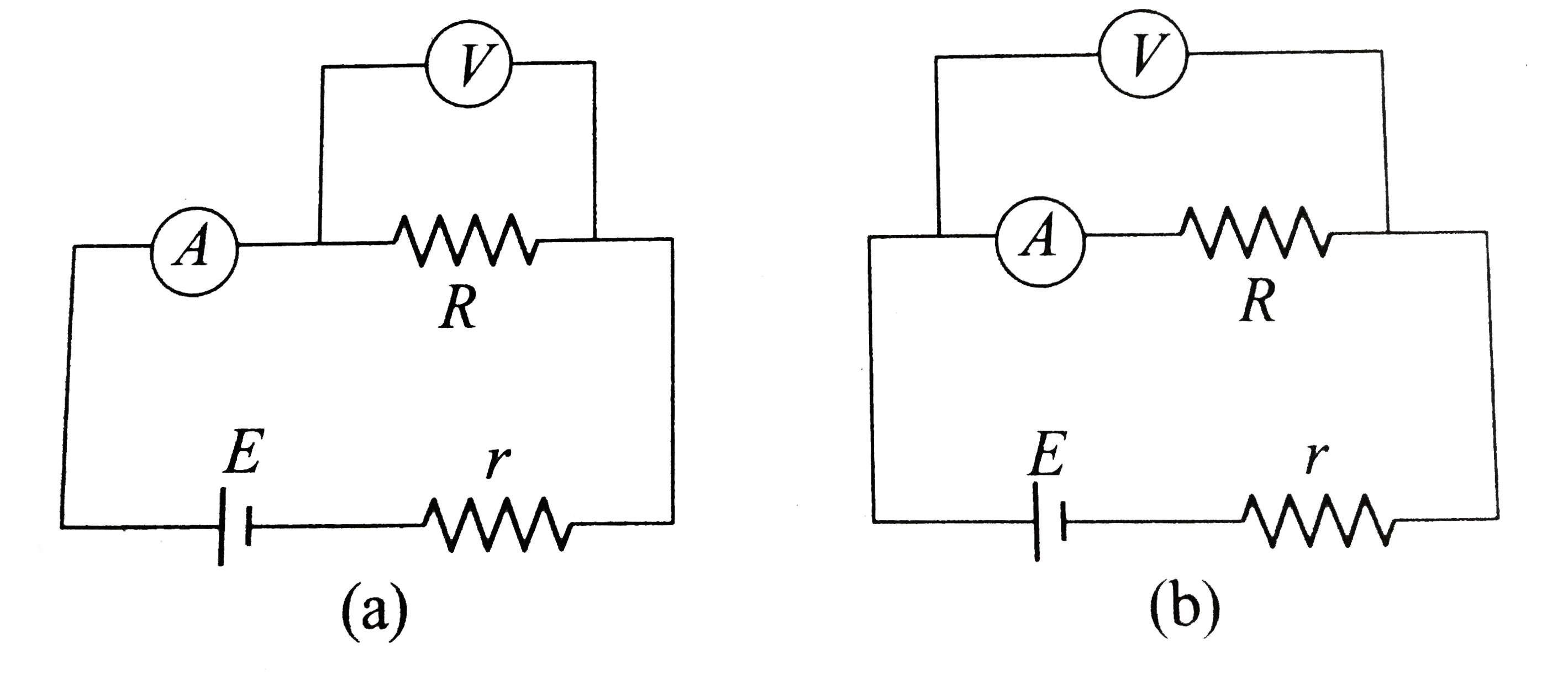 ,
,
Let RAandRB be the calculated values in the two cases A and B , respectively.
If the resistance of voltmeter is RV=1kΩ and the percentage error in the measurement of R (the value of R is nearly 10Ω)is
View SolutionThe resistance of a metal is given by R=V/I , where V is potential difference and I is current . In a circuit , the potential difference across resistance is V=(8±0.5)V and current in resistance , I=(4±0.2)A. What is the value of resistance with its percentage error?
View SolutionIn Ohm's law experiment , the potential drop acros a resistance was as V=5.0V and the current was measured as I=2.00A. Find the maximum permissible error in resistance.
View SolutionIn the circuit shown in Fig. the emf of the sources is equal to ξ=5.0V and the resistances are equal to R1=4.0Ω and R2=6.0Ω. The internal resistance of the source equals R=1.10Ω. Find the currents flowing through the resistances R1 and R2.
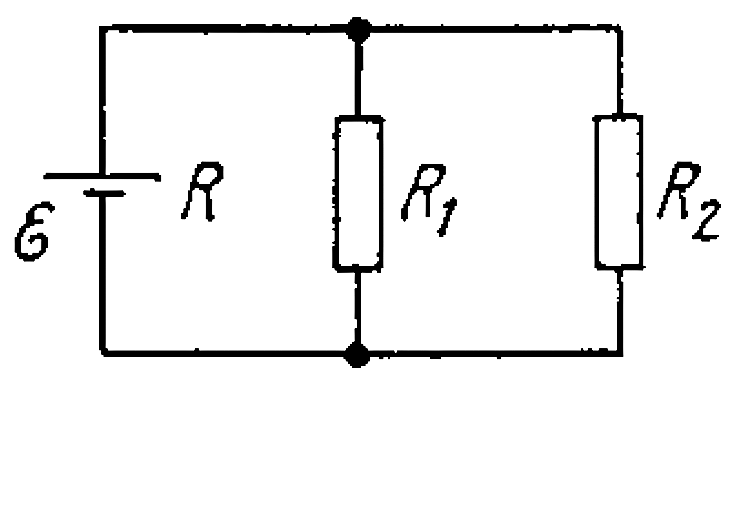
View Solution