A small air bubble of radius 'r' is at a depth 'h' below the water surface (density of water=ρ). Surface tension of water is T, atmospheric pressure is p0. Find pressure inside the air bubble for the condition r<<h
The correct Answer is:B
To find the pressure inside the air bubble at a depth 'h' below the water surface, we will follow these steps:
1. Understand the Pressure at Depth:
The pressure at a depth 'h' in a fluid is given by the hydrostatic pressure formula:
Ph=P0+ρgh
where:
- Ph is the pressure at depth 'h',
- P0 is the atmospheric pressure at the water surface,
- ρ is the density of water,
- g is the acceleration due to gravity,
- h is the depth below the water surface.
2. Consider the Effect of Surface Tension:
The air bubble is subjected to surface tension, which affects the pressure inside the bubble. For a spherical bubble, the pressure difference between the inside and outside of the bubble due to surface tension is given by:
ΔP=2Tr
where:
- T is the surface tension of water,
- r is the radius of the bubble.
3. Relate Inside Pressure to Outside Pressure:
The pressure inside the bubble P can be expressed as:
P=Ph+ΔP
Substituting the expressions for Ph and ΔP:
P=(P0+ρgh)+2Tr
4. Final Expression for Pressure Inside the Bubble:
Combining all the terms, we have:
P=P0+ρgh+2Tr
Final Answer:
The pressure inside the air bubble at a depth 'h' is given by:
P=P0+ρgh+2Tr
The pressure at a depth 'h' in a fluid is given by the hydrostatic pressure formula:
Ph=P0+ρgh
where:
- Ph is the pressure at depth 'h',
- P0 is the atmospheric pressure at the water surface,
- ρ is the density of water,
- g is the acceleration due to gravity,
- h is the depth below the water surface.
The air bubble is subjected to surface tension, which affects the pressure inside the bubble. For a spherical bubble, the pressure difference between the inside and outside of the bubble due to surface tension is given by:
ΔP=2Tr
where:
- T is the surface tension of water,
- r is the radius of the bubble.
The pressure inside the bubble P can be expressed as:
P=Ph+ΔP
Substituting the expressions for Ph and ΔP:
P=(P0+ρgh)+2Tr
Combining all the terms, we have:
P=P0+ρgh+2Tr
The pressure inside the air bubble at a depth 'h' is given by:
P=P0+ρgh+2Tr
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.8
4 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Assertion And Reason
10 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.6
4 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
An air bubble of radius r in water is at a depth h below the water surface at some instant. If P is atmospheric pressure, d and T are density and surface tension of water respectively . the pressure inside the bubble will be :
View SolutionWater is filled up to a height h in a beaker of radius R as shown in the figure. The density of water is ρ, the surface tension of water is T and the atmospheric presure is p0. Consider a vertical section ABCDof the water on one side of this section by water on the other side of this section has magnitude
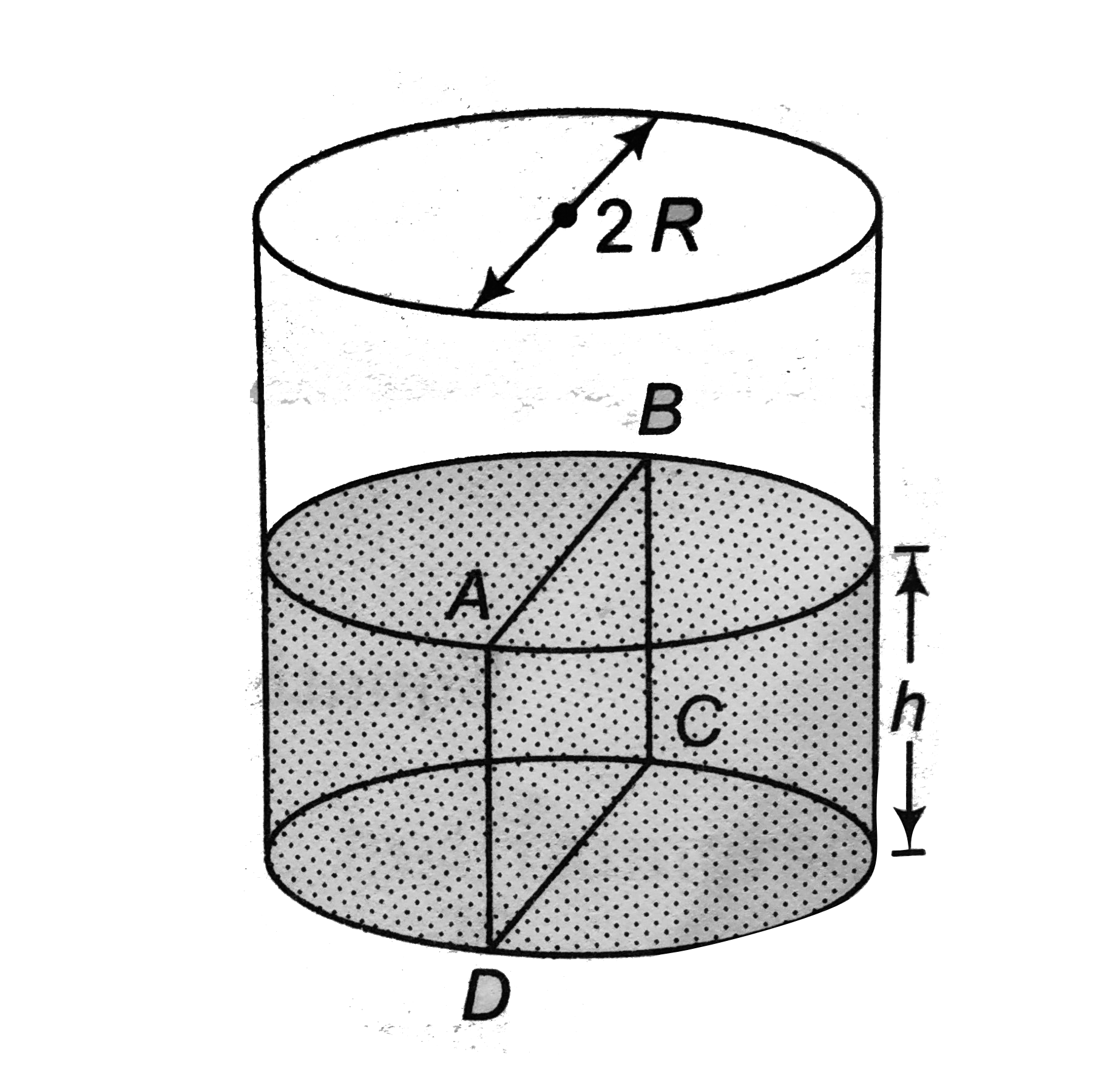
(a) ∣∣2p0Rh+πR2ρgh−2RT∣∣
(b)∣∣2p0Rh+Rρgh2=2RT∣∣
(c ) ∣∣p0(π)R2+Rρgh2−2RT∣∣
(d) ∣∣p0πR2+Rρgh2+2RT∣∣.
View Solution
Knowledge Check
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth
An air bubble is lying just below the surface of water. The surface tension of water is 70×10−3Nm−1 and atmospheric pressure is 1.013×105Nm−2. If the radius of bubble is 1mm, then the pressure inside the bubble will be-
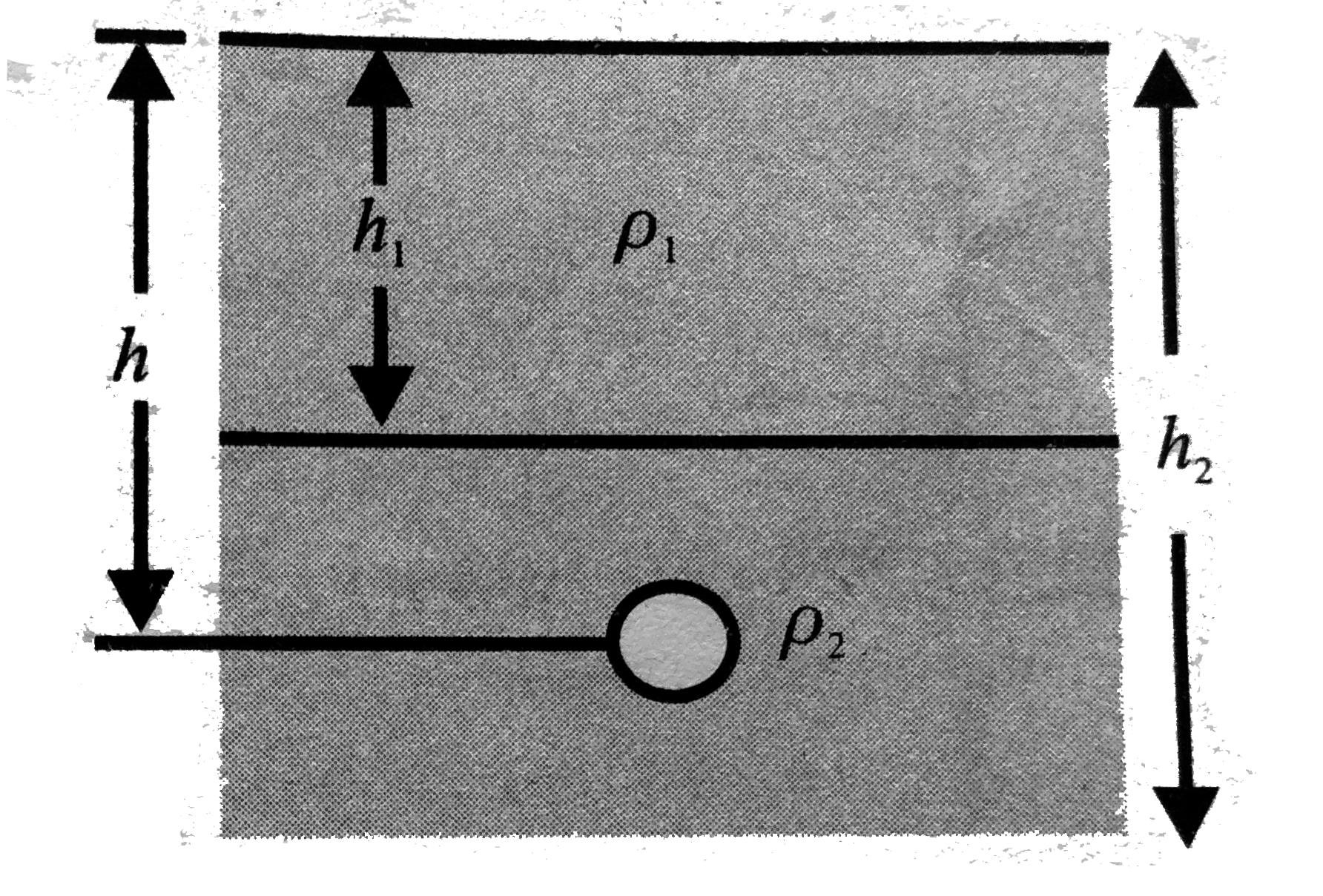
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
View SolutionAn air bubble of radius 1mm is formed inside water at a depth 10m below free surface (where air pressure is 105Nm2). The pressure inside the bubble is – (Surface tension of water = 7x×10–2N/m)
View SolutionCalculate the pressue inside air bubble of diameter 0.2 mm situated just below the surface of water. Surface tension of water is 72×10−3Nm−1.
View SolutionThe pressure inside an air bubble of radius 2 cm formed 20 cm below an open water surface is (Given surface tension of water = 70×10−3Nm−1)
View SolutionThe absolute pressure at a depth h below the surface of a liquid of density ρ is [Given Pa = atmospheric pressure, g = acceleration due to gravity]
View SolutionWhen an air bubble rise from the bottom of a deep lake to a point just below the water surface, the pressure of air inside the bubble
View Solution
