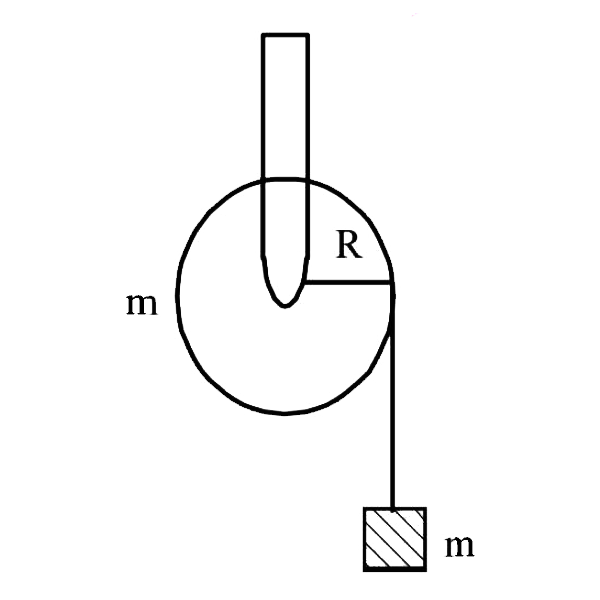
A bucket of mass 8 kg is supported by a light rope wound around a solid wooden cylinder of mass 12 kg and radius 20 cm free to rotate about its axis. A man holding the free end of the rope, with the bucket and the cylinder at rest initially, lets go the bucket freely downwards in a well 50 m deep. Neglecting friction, obtain the speed of the bucket and the angular speed of the cylihder just before the bucket enters water.
To solve the problem, we will use the principle of conservation of energy. The initial potential energy of the bucket will be converted into kinetic energy of the bucket and rotational kinetic energy of the cylinder as the bucket falls.
1. Identify the given values:
- Mass of the bucket, mb=8kg
- Mass of the cylinder, mc=12kg
- Radius of the cylinder, r=0.2m
- Height of the well, h=50m
- Acceleration due to gravity, g=9.81m/s2
2. Calculate the initial potential energy (PE_initial) of the bucket:
PEinitial=mb⋅g⋅h=8⋅9.81⋅50
PEinitial=3924J
3. Set up the energy conservation equation:
The initial potential energy will be equal to the sum of the kinetic energy of the bucket and the rotational kinetic energy of the cylinder just before the bucket enters the water.
PEinitial=KEbucket+KEcylinder
3924=12mbv2+12Iω2
4. Calculate the moment of inertia (I) of the cylinder:
The moment of inertia for a solid cylinder is given by:
I=12mcr2=12⋅12⋅(0.2)2
I=12⋅12⋅0.04=0.24kg m2
5. Relate the angular velocity (ω) to the linear velocity (v):
The linear velocity of the bucket and the angular velocity of the cylinder are related by:
v=rω⇒ω=vr
6. Substitute ω into the energy equation:
Substitute ω=vr into the kinetic energy of the cylinder:
KEcylinder=12I(vr)2=12⋅0.24⋅(v0.2)2
KEcylinder=0.242⋅0.04v2=3v2
7. Substitute back into the energy conservation equation:
3924=12⋅8v2+3v2
3924=4v2+3v2=7v2
v2=39247≈560.57
v≈√560.57≈23.7m/s
8. Calculate the angular speed (ω) of the cylinder:
ω=vr=23.70.2≈118.5rad/s
Final Answers:
- Speed of the bucket just before entering the water: v≈23.7m/s
- Angular speed of the cylinder just before the bucket enters the water: ω≈118.5rad/s
- Mass of the bucket, mb=8kg
- Mass of the cylinder, mc=12kg
- Radius of the cylinder, r=0.2m
- Height of the well, h=50m
- Acceleration due to gravity, g=9.81m/s2
PEinitial=mb⋅g⋅h=8⋅9.81⋅50
PEinitial=3924J
The initial potential energy will be equal to the sum of the kinetic energy of the bucket and the rotational kinetic energy of the cylinder just before the bucket enters the water.
PEinitial=KEbucket+KEcylinder
3924=12mbv2+12Iω2
The moment of inertia for a solid cylinder is given by:
I=12mcr2=12⋅12⋅(0.2)2
I=12⋅12⋅0.04=0.24kg m2
The linear velocity of the bucket and the angular velocity of the cylinder are related by:
v=rω⇒ω=vr
Substitute ω=vr into the kinetic energy of the cylinder:
KEcylinder=12I(vr)2=12⋅0.24⋅(v0.2)2
KEcylinder=0.242⋅0.04v2=3v2
3924=12⋅8v2+3v2
3924=4v2+3v2=7v2
v2=39247≈560.57
v≈√560.57≈23.7m/s
ω=vr=23.70.2≈118.5rad/s
- Speed of the bucket just before entering the water: v≈23.7m/s
- Angular speed of the cylinder just before the bucket enters the water: ω≈118.5rad/s
Topper's Solved these Questions
Similar Questions
A solid cylinder of mass 2 kg and radius 4 cm rotating about its axis at the rate of 3 rpm. The torque required to stop after 2π revolutions is :
View SolutionA rope is wound around a hollow cylinder of mass 3kg and radius 40cm. What is the angular acceleration of the cylinder if the rope is pulled with a force of 30N ?
View Solution
Knowledge Check
- Question 1 - Select One
A rope is wound round a hollow cylinder of mass 3kgandradius40cm. If the rope is pulled with a force of 30N, what is the angualr acceleration of the cylinder ?
A15rads−2B20rads−2C25rads−2D30rads−2 - Question 2 - Select One
A solid cylinder of mass M and radius R rotates about its axis with angular speed ω. Its rotational kinetic energy is
A12MR2ω2BMR2ω2C14MR2ω2D18MR2ω2 - Question 3 - Select One
An solid cylinder of mass 20kg and radius 20cm rotates about its axis with a angular speed 100rads−1. The angular momentum of the cylinder about its axis is: a) 40 J s b) 400 J s c) 20 J s d) 200 J s
A40 J sB400 J sC20 J sD200 J s
A rope is wound around a hollow cylinder of mass 3kg and radius 40cm. What is the angular acceleration of the cylinder if the rope is pulled with a force of 30N ?
View SolutionA mass M is supported by a massless string wound round a uniform cylinder ofmass M and radius R. On releasing the mass from rest, it will fall with acceleration?
 View Solution
View SolutionA soild cylinder of mass 2 kg and radius 0.2 m is rotating about its owm axis without friction with an angular velocity of 3 rad s−1 . Angular momentum of the cylinder is
View SolutionA mass 'm' is supported by a massless string wound around a uniform hollow cylinder of mass m and radius R. If the string does not slip on the cylinder, with what acceleration will the mass fall or release?
 View Solution
View SolutionAn angular impulse of 20 Nms is applied to a hollow cylinder of mass 2 kg and radius 20 cm. The change in its angular speed is :
View Solution
