A race car negotiates a curve of radius 50.0 m on a flat road. What is its angular displacement when it covers a linear distance of 78.5 m?
To solve the problem of finding the angular displacement of a race car negotiating a curve, we can follow these steps:
Step 1: Identify the given values.
- Radius of the curve (R) = 50.0 m
- Linear distance covered (L) = 78.5 m
Step 2: Use the relationship between linear distance and angular displacement.
The relationship between linear distance (L), radius (R), and angular displacement (θ) in radians is given by the formula:
L=R⋅θ
From this, we can express angular displacement as:
θ=LR
Step 3: Substitute the known values into the formula.
Substituting the values we have:
θ=78.5m50.0m
Step 4: Calculate the angular displacement in radians.
Calculating the above expression:
θ=78.550=1.57radians
Step 5: Convert the angular displacement from radians to degrees.
To convert radians to degrees, we use the conversion factor:
Degrees=θ×180π
Substituting the value of θ:
Degrees=1.57×180π
Step 6: Calculate the angular displacement in degrees.
Calculating this gives:
Degrees≈90∘
Final Answer:
The angular displacement of the race car when it covers a linear distance of 78.5 m is approximately:
- 1.57 radians or 90 degrees.
---
- Radius of the curve (R) = 50.0 m
- Linear distance covered (L) = 78.5 m
The relationship between linear distance (L), radius (R), and angular displacement (θ) in radians is given by the formula:
L=R⋅θ
From this, we can express angular displacement as:
θ=LR
Substituting the values we have:
θ=78.5m50.0m
Calculating the above expression:
θ=78.550=1.57radians
To convert radians to degrees, we use the conversion factor:
Degrees=θ×180π
Substituting the value of θ:
Degrees=1.57×180π
Calculating this gives:
Degrees≈90∘
The angular displacement of the race car when it covers a linear distance of 78.5 m is approximately:
- 1.57 radians or 90 degrees.
Topper's Solved these Questions
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 1 (CONCEPTUAL SHORT ANSWERS QUESTIONS WITH ANSWERS )
Explore 11 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 1 (LONG ANSWER QUESTIONS)
Explore 8 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
Explore 50 Videos
Similar Questions
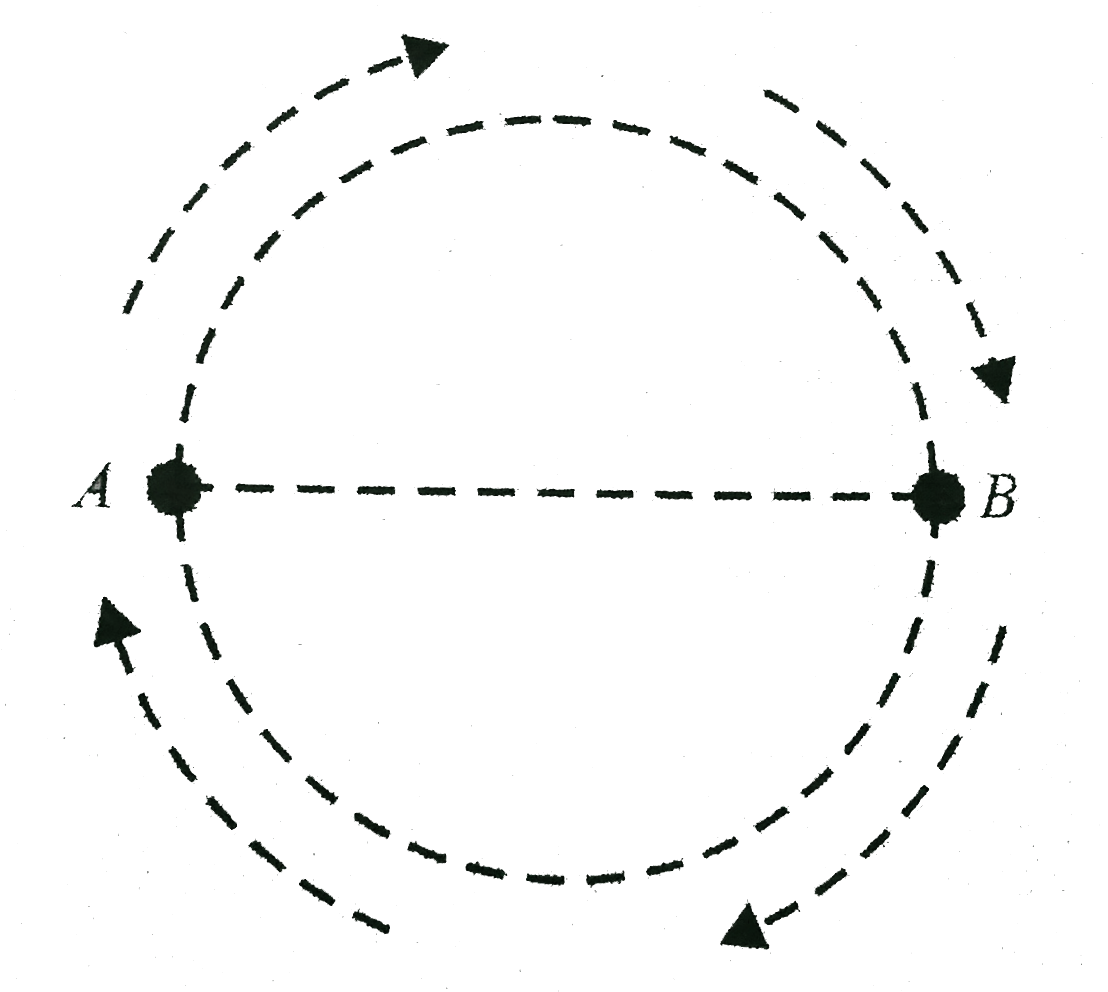
A particle is moving in a circle of radius R.
a. What is its displacement when it covers (i) half the circle, (ii) full circle?
b. What is its distance when it comers (i) half the the circle and (ii) full circle ?.
 .
.
View SolutionA train has to negotiate a curve of radius 2000 m. By how much should the outer rail be raised with respect to inner rail for a speed of 72kmh−1. The distance between the rails is 1 m.
View SolutionA car of mass 1000kg negotiates a banked curve of radius 90m on a frictionless road. If the banking angle is 45∘ the speed of the car is:
View SolutionA car of mass 1000kg negotiates a banked curve of radius 90m on a fictionless road. If the banking angle is 45∘ the speed of the car is:
View SolutionThe distance between the inner and outer wheels of a car is 1.75 m and its centre of gravity is 0-50 m above the ground. What is the maximum speed with which the car can negotiate a curve of radius of 40 m without overturning ?
View SolutionA uniform sphere of radius 0.25 m and mass 2 kg rotates about its diameter. What is the angular momentum when the angular speed is 5 rad/s ?
View SolutionA train has to negotiate a curve of 40 m radius. What is the super elevation of the outer rail required for a speed of 54kmh−1. The distance between the rails is one metre ?
View SolutionA pick up van negotiates an unbanked curve of radius 40 m with a crate of eggs in the middle of its flat bed. If the coefficient of static friction between the crate and the truck, is 0.55 what is the maximum speed the van can take, so that the crate does not slide ?
View SolutionCalculate the safe speed with which a train can negotiate a curve of 50 m radius, where the superelevation of the outer rail above the inner rail is 0.6 m. Given that the distance between the rails is 1 m
View SolutionCalculate the magnitude of linear acceleration of a particle moving in a circle of radius 0.5 m at the instant when its angular velocity is 2.5 rad s–1 and its angular acceleration is 6rads−2.
View Solution