A source of light is located at double focal length from a convergent lens. The focal length of the lens is f=30cm. At what distance from the lens should a flat mirror be placed, so that the rays reflected from the mirror are parallel after passing through the lens for the second time?
To solve the problem, we need to determine the distance from the convergent lens where a flat mirror should be placed so that the rays reflected from the mirror are parallel after passing through the lens for the second time.
1. Identify the Focal Length:
The focal length of the lens is given as f=30cm.
2. Determine the Object Distance:
The source of light is located at double the focal length from the lens, which is 2f=2×30cm=60cm.
3. Locate the Image Formed by the Lens:
When the object is at 2f, the image formed by the lens will also be at 2f on the opposite side of the lens. Thus, the image distance v=60cm.
4. Understanding the Requirement for Parallel Rays:
For the rays reflected from the mirror to be parallel after passing through the lens for the second time, the image formed by the mirror must be at the focal point of the lens. Therefore, we need to place the mirror such that the image formed by the mirror acts as an object at the focal point of the lens.
5. Positioning the Mirror:
Let the distance from the lens to the mirror be d. The distance from the mirror to the image formed by the lens (which is at 60cm) will then be 60−d.
6. Setting Up the Equation:
For the rays to be parallel after passing through the lens for the second time, the distance from the mirror to the image formed by the lens must equal the focal length of the lens:
60−d=f
Substituting f=30cm:
60−d=30
7. Solving for d:
Rearranging the equation gives:
d=60−30=30cm
8. Final Distance of the Mirror:
Therefore, the flat mirror should be placed at a distance of 30cm from the lens.
Conclusion:
The flat mirror should be placed at a distance of 30 cm from the convergent lens.
The focal length of the lens is given as f=30cm.
The source of light is located at double the focal length from the lens, which is 2f=2×30cm=60cm.
When the object is at 2f, the image formed by the lens will also be at 2f on the opposite side of the lens. Thus, the image distance v=60cm.
For the rays reflected from the mirror to be parallel after passing through the lens for the second time, the image formed by the mirror must be at the focal point of the lens. Therefore, we need to place the mirror such that the image formed by the mirror acts as an object at the focal point of the lens.
Let the distance from the lens to the mirror be d. The distance from the mirror to the image formed by the lens (which is at 60cm) will then be 60−d.
For the rays to be parallel after passing through the lens for the second time, the distance from the mirror to the image formed by the lens must equal the focal length of the lens:
60−d=f
Substituting f=30cm:
60−d=30
Rearranging the equation gives:
d=60−30=30cm
Therefore, the flat mirror should be placed at a distance of 30cm from the lens.
The flat mirror should be placed at a distance of 30 cm from the convergent lens.
Topper's Solved these Questions
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Exercise 31.1
Explore 3 Videos
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Exercise 31.2
Explore 2 Videos
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Example Type 11
Explore 3 Videos
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
- SEMICONDUCTORSBook:DC PANDEY ENGLISHChapter:SEMICONDUCTORSExercise:Subjective
Explore 12 Videos
Similar Questions
A point source of light is placed at a distance of 2f from a converging lens of focal length f. The intensity onteh other side of the lens is maximum at a distance
View SolutionA parallel beam of rays is incident on a convergent lens with a focal length of 40cm. Where should a divergent lens with a focal length of 15cm be placed for the beam of rays to remain parallel after passing through the two lenses.
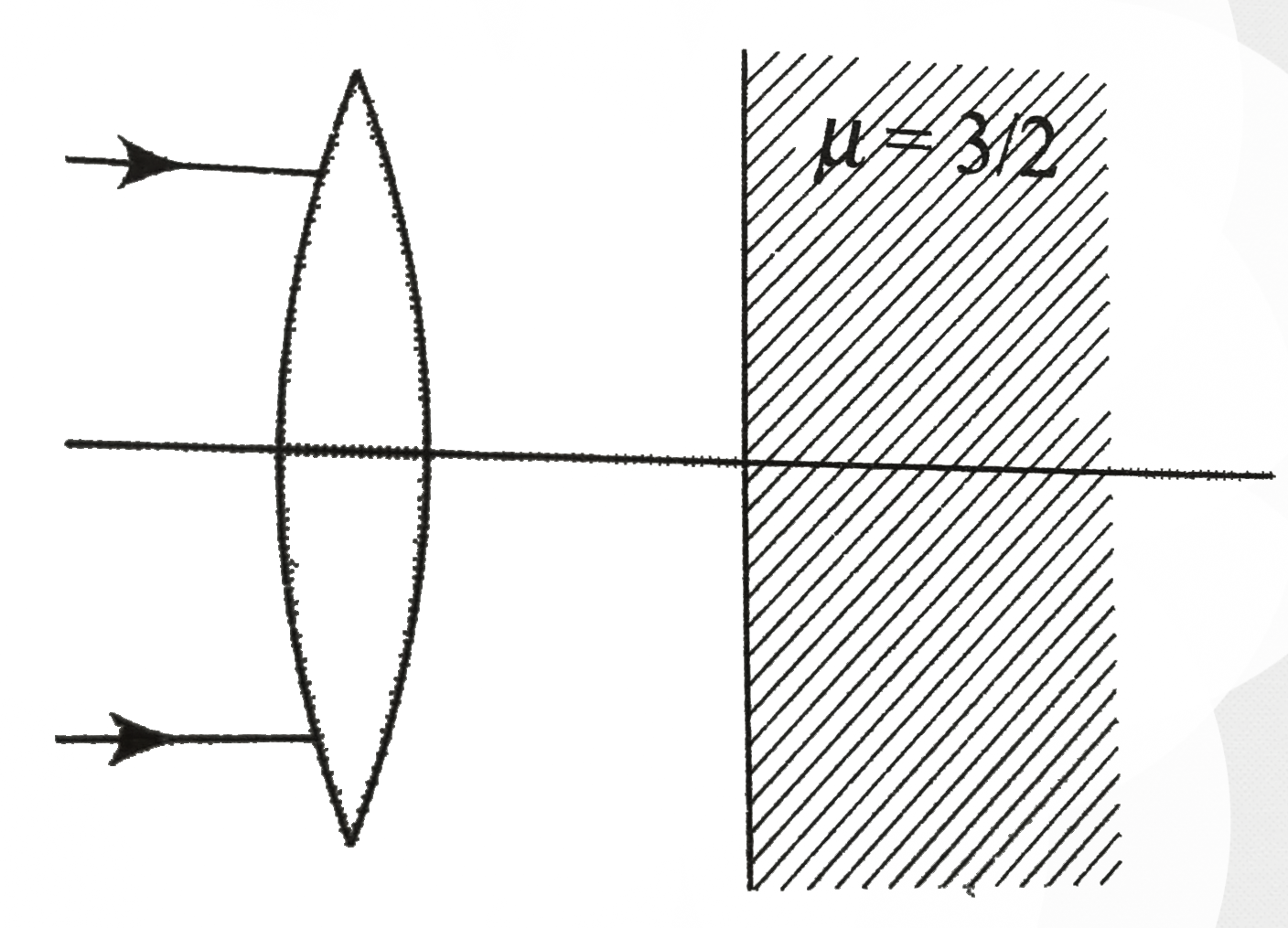
View SolutionThe focal length of a thin convex-lens is 30cm. At a distance of 10 cm from the lens there is a plane refracting surface fo refractive inidex 3/2 Where will parallel rays incident on lens converge?
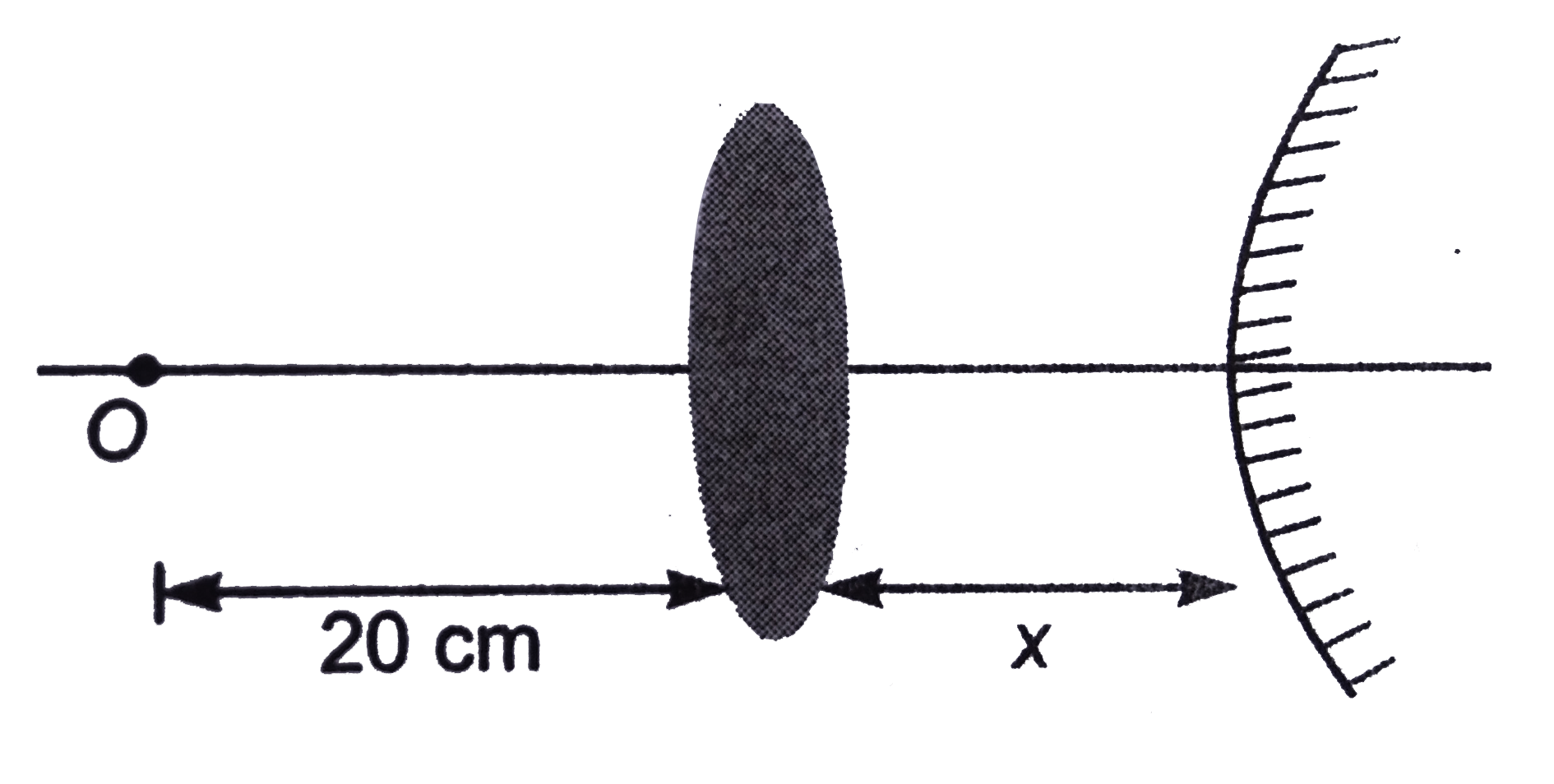
View SolutionA point object O is placed at a distance of 20 cm from a convex lens of focal length 10 cm as shown in figure. At what distance x from the lens should a concave mirror of focal length 60 cm, placed so that final image coincides with the object ?

View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
View SolutionA concave lens has focal length of 15 cm. At what distance should an object from the lens be placed so that it forms an image at 10 cm from the lens ? Also, find the magnification of the lens.
View SolutionFocal length of a thin convex lens is 30 cm. At distance of 10 cm from the lens there is a plan refracting surface of refractive index 3/2. Where w the parallel rays incident on lens converge ?
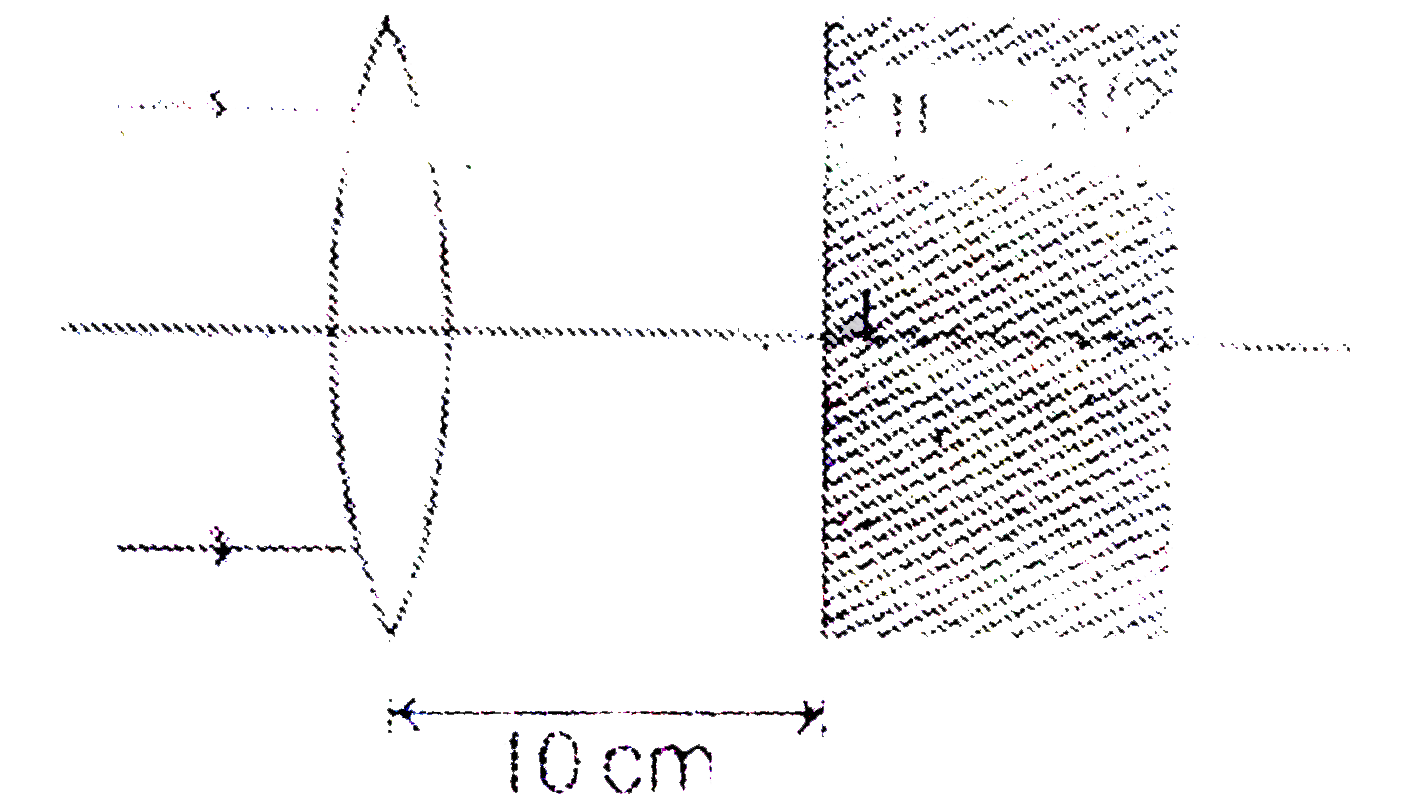
View SolutionFind distanace of image from a convex lens of focal length
20cm if object is placed at a distance of 30cm from the lens. Also find its
magnification.
View SolutionA point object O is placed at a distance of 20cm from a convex lens of focal length 10cm as shown in the figure. At what distance x from the lens should a convex mirror of focal length 60cm, be placed so that final image coincide with the object?
View SolutionA point source of light is kept at a distance of 15cm from a converging lens,on its optical axis.The focal length of the lens is 10cm and its diameter is 3cm ,A screen is placed on the other side of the lens ,perpendicular to the axis of lens,at a distance 20cm from it.Then find the area of the illuminated part of the screen?
View Solution