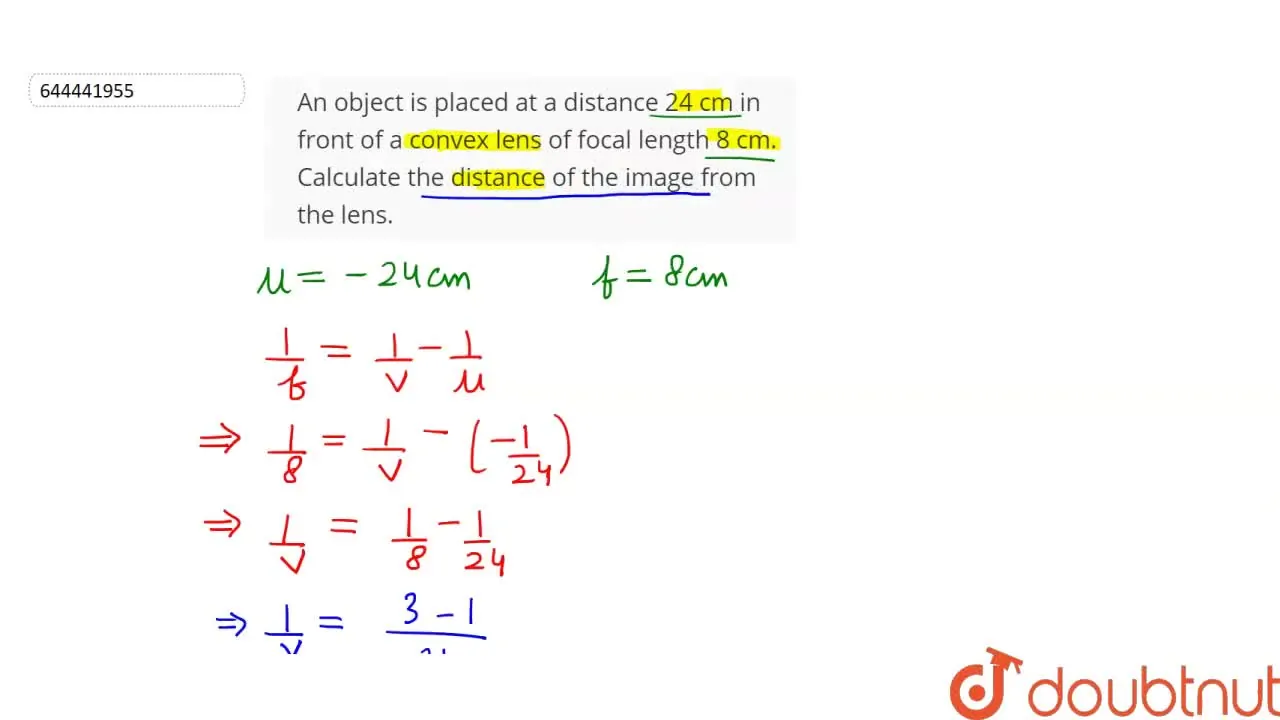
An object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
Given u=24cm (- ve ) f= 8 cm ( +ve )
Let v be the distance of image from optical centre. From the relation 1v−1u=1f
we have :1v=124=18,orv=12cm
∴ The distance of image from the lens = 12 cm.
Related Playlists
Similar Questions
An object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
(i) What is the nature of the image so formed ?
(ii) Calculate the distance of the image from the lens.
(iii) Calculate the magnification of the image.View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the imageView SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the imageView SolutionAn object is placed at a distance of f2 from a convex lens of focal length f. The image will be
View SolutionA point object is placed on the axis in front of a convex lens of focal length 30 cm. The distance of the object from the lens is 45 cm. If the lens is moved away from object by 1 mm and also the object is moved apart from the line joining the object and optical centre by 1 mm the displacement of the image has magnitude-
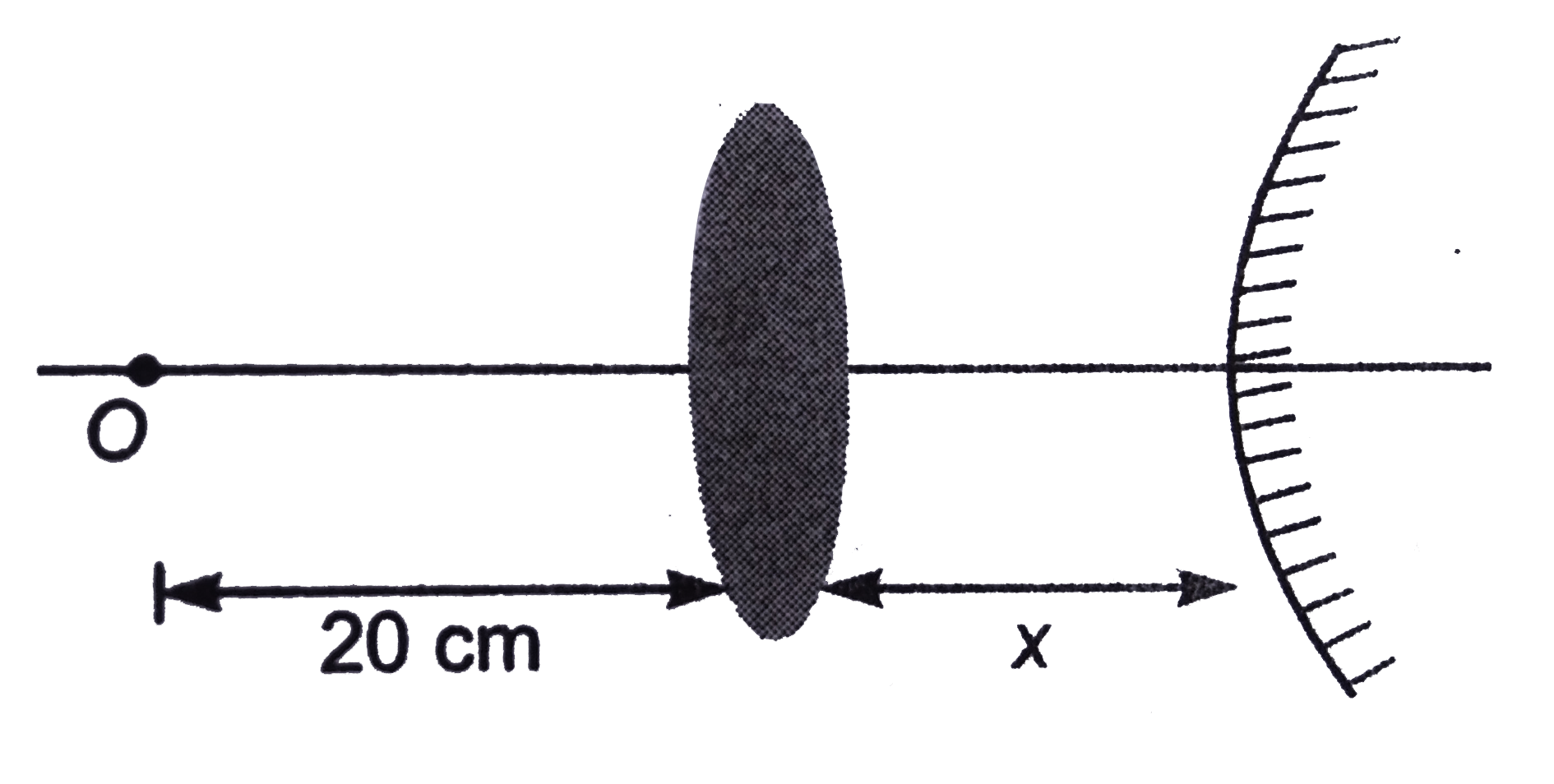
View SolutionA point object O is placed at a distance of 20 cm from a convex lens of focal length 10 cm as shown in figure. At what distance x from the lens should a concave mirror of focal length 60 cm, placed so that final image coincides with the object ?
 View Solution
View SolutionA point object O is placed at a distance of 20cm from a convex lens of focal length 10cm as shown in the figure. At what distance x from the lens should a convex mirror of focal length 60cm, be placed so that final image coincide with the object?
 View Solution
View SolutionAn object is kept at a distance of 15 cm from a convex lens of focal length 10 cm. Calculate the image distance and state the characteristics of the image formed.
View SolutionAn object is placed at a distance of 48 cm in front of a concave mirror of focal length 24 cm.
a. Find the position of image.
b. What will be the nature of image?View SolutionA point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View Solution