An object is kept at a distance of 15 cm from a convex lens of focal length 10 cm. Calculate the image distance and state the characteristics of the image formed.
To solve the problem, we will use the lens formula and the given data to find the image distance and the characteristics of the image formed by the convex lens.
1. Identify the Given Data:
- Object distance (u) = -15 cm (negative as per sign convention for real objects)
- Focal length (f) = +10 cm (positive for convex lens)
2. Use the Lens Formula:
The lens formula is given by:
1v−1u=1f
Where:
- v = image distance
- u = object distance
- f = focal length
3. Substitute the Values into the Lens Formula:
1v−1−15=110
This simplifies to:
1v+115=110
4. Rearrange the Equation:
To isolate 1v:
1v=110−115
5. Find a Common Denominator:
The least common multiple (LCM) of 10 and 15 is 30. Thus, we rewrite the fractions:
110=330and115=230
Therefore:
1v=330−230=130
6. Calculate the Image Distance (v):
Taking the reciprocal gives:
v=30 cm
7. Determine the Characteristics of the Image:
To find the characteristics of the image, we calculate the magnification (m):
m=vu=30−15=−2
- The negative sign indicates that the image is inverted.
- The magnitude of magnification (2) indicates that the image is enlarged.
Characteristics of the Image:
- Type: Real
- Orientation: Inverted
- Size: Enlarged (twice the size of the object)
Summary:
- The image distance is v=30 cm.
- The characteristics of the image are that it is real, inverted, and enlarged.
- Object distance (u) = -15 cm (negative as per sign convention for real objects)
- Focal length (f) = +10 cm (positive for convex lens)
The lens formula is given by:
1v−1u=1f
Where:
- v = image distance
- u = object distance
- f = focal length
1v−1−15=110
This simplifies to:
1v+115=110
To isolate 1v:
1v=110−115
The least common multiple (LCM) of 10 and 15 is 30. Thus, we rewrite the fractions:
110=330and115=230
Therefore:
1v=330−230=130
Taking the reciprocal gives:
v=30 cm
To find the characteristics of the image, we calculate the magnification (m):
m=vu=30−15=−2
- The negative sign indicates that the image is inverted.
- The magnitude of magnification (2) indicates that the image is enlarged.
- Type: Real
- Orientation: Inverted
- Size: Enlarged (twice the size of the object)
- The image distance is v=30 cm.
- The characteristics of the image are that it is real, inverted, and enlarged.
Topper's Solved these Questions
Similar Questions
An object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the image
View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the image
View SolutionAn object is placed at a distance 20 cm from the pole of a convex mirror of focal length 20 cm. The image is produced at
View SolutionAn extended object is placed at a distance of 5.0 cm from a convex lens of focal length 8.0 cm. (a) Draw the ray diagram (to the scale) to locate the image and from this, measure the distance of the image from the lens, (b) Find the position of the image from the lens formula and see how close the drawing is to the correct result.
View SolutionA point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View SolutionA point object O is placed at a distance of 20cm from a convex lens of focal length 10cm as shown in the figure. At what distance x from the lens should a convex mirror of focal length 60cm, be placed so that final image coincide with the object?
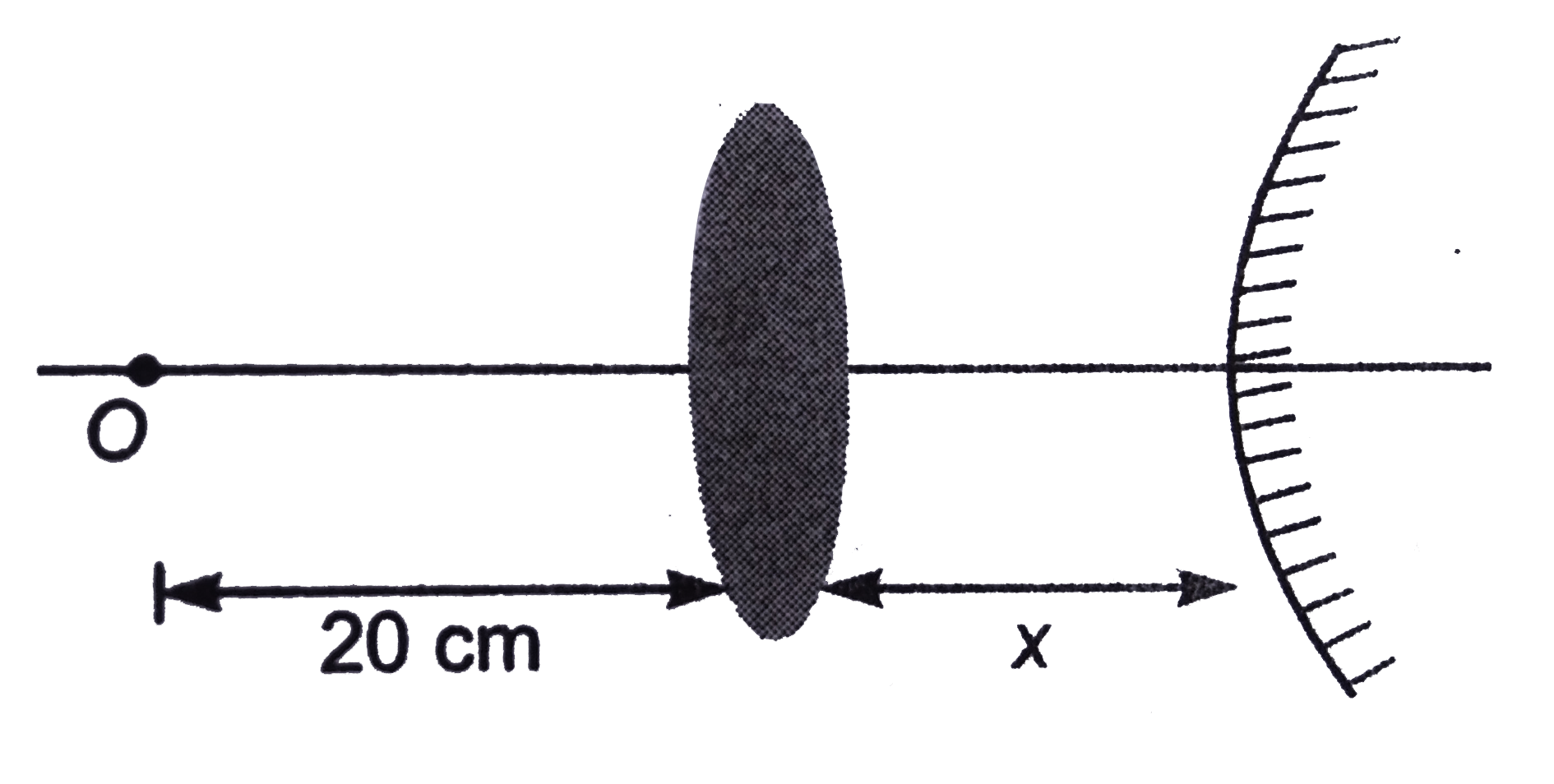
View SolutionA point object is placed at distance of 30 cm in front of a convex mirror of Focal length 30 cm. The image will form at
View SolutionA luminous object is placed at a distance of 30 cm. from the convex lens of focal length 20 cm. On the other side of the lens, at what distance from the lens, a convex mirror of radius of curvature 10 cm. be placed in order to have an upright image of the object coincident with it ?
View SolutionA point object O is placed at a distance of 15 cm from a convex lens L of focal length 10 cm as shown in figure below. On the other side of the lens, a convex mirror M is placed such that its distance from the lens is equal to the focal length of the lens. The final image formed by this combination is observed to coincide with the object 0. Find the focal length of the convex mirror.
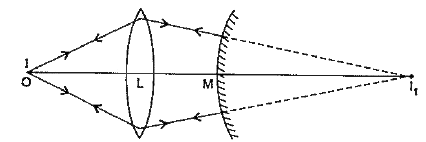
View Solution
