There is a horizontal film of soap solution. On it a thread is placed in the form of a loop. The film is pierced inside the loop and the thread becomes a circular loop of radius R. If the surface tension of the loop be T, then what will be the tension in the thread?
πR2T
π2R2T
2πRT
2RT
The correct Answer is:D
To solve the problem of finding the tension in the thread when a soap film is pierced, we can follow these steps:
1. Understand the Setup:
- We have a horizontal soap film with a thread forming a loop. When the film is pierced, the thread takes the shape of a circular loop with radius R.
- The surface tension of the soap film is denoted as T.
2. Identify Forces Acting on the Thread:
- The surface tension acts along the surface of the soap film, pulling the thread inward. Since the soap film has two surfaces (front and back), the effective surface tension acting on the thread will be 2T.
3. Consider the Geometry of the Circular Loop:
- Let’s consider a small segment of the circular loop. If we take two points on the loop that subtend an angle 2θ at the center, we can analyze the forces acting on this segment.
4. Resolve the Tension:
- The tension T′ in the thread will have components acting vertically due to the surface tension. For the two segments of the thread at angle θ:
- The vertical component of the tension from each side is T′sin(θ).
- Therefore, the total upward force from both sides is 2T′sin(θ).
5. Calculate the Arc Length:
- The arc length s subtended by the angle 2θ at radius R is given by:
s=R⋅2θ
- Since there are two surfaces of the soap film, the effective length contributing to the surface tension is 2s.
6. Set Up the Force Balance:
- The total upward force due to surface tension must balance the downward force due to the tension in the thread:
2T′sin(θ)=2T⋅s
- Substituting s=R⋅2θ:
2T′sin(θ)=2T⋅(R⋅2θ)
7. Simplify the Equation:
- Canceling out the common factors of 2:
T′sin(θ)=2T⋅R⋅θ
8. Approximate for Small Angles:
- For small angles, sin(θ)≈θ. Thus, we can replace sin(θ) with θ:
T′θ=2T⋅R⋅θ
9. Solve for Tension in the Thread:
- Dividing both sides by θ (assuming θ≠0):
T′=2T⋅R
Final Answer:
The tension in the thread is given by:
T′=2TR
- We have a horizontal soap film with a thread forming a loop. When the film is pierced, the thread takes the shape of a circular loop with radius R.
- The surface tension of the soap film is denoted as T.
- The surface tension acts along the surface of the soap film, pulling the thread inward. Since the soap film has two surfaces (front and back), the effective surface tension acting on the thread will be 2T.
- Let’s consider a small segment of the circular loop. If we take two points on the loop that subtend an angle 2θ at the center, we can analyze the forces acting on this segment.
- The tension T′ in the thread will have components acting vertically due to the surface tension. For the two segments of the thread at angle θ:
- The vertical component of the tension from each side is T′sin(θ).
- Therefore, the total upward force from both sides is 2T′sin(θ).
- The arc length s subtended by the angle 2θ at radius R is given by:
s=R⋅2θ
- Since there are two surfaces of the soap film, the effective length contributing to the surface tension is 2s.
- The total upward force due to surface tension must balance the downward force due to the tension in the thread:
2T′sin(θ)=2T⋅s
- Substituting s=R⋅2θ:
2T′sin(θ)=2T⋅(R⋅2θ)
- Canceling out the common factors of 2:
T′sin(θ)=2T⋅R⋅θ
- For small angles, sin(θ)≈θ. Thus, we can replace sin(θ) with θ:
T′θ=2T⋅R⋅θ
- Dividing both sides by θ (assuming θ≠0):
T′=2T⋅R
The tension in the thread is given by:
T′=2TR
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 More Than One Correct
10 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Comprehension Based
7 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
What will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionA massless inextensible string in the form of a loop is placed on a horizontal film of soap solution of surface tension T. If film is pierced inside the loop and it convert into a circular loop of diameter d, then the tension produced in string is
View SolutionA wire forming a loop is dipped into soap solution and taken out so that a film of soap solution is formed. A loop of 6.28 cm long thread is gently put on the film and the film is pricked with a needle inside the loop. The thread loop takes the shape of a circle. Find the tension in the thread. Surface tension of soap solution =0.030Nm−1.
View SolutionA wire forming a loop is dipped into soap solution and taken out so that a film of soap solution is formed. A loop of 6.28 cm long thread is gently put on the film and the film is pricked with a needle inside the loop. The thread loop takes the shape of a circle. Find the tension in the thread. Surface tension of soap solution =0.030Nm−1.
View SolutionThe excess pressure inside a soap bubble of radius R is (S is the surface tension)
View SolutionOn meter length of wires carriers a constant current. The wire is bent to from a circular loop. The magnetic field at the centre of this loop is B. The same is now bent to form a circular loop of smaller radius to have four turns in the loop. The magnetic field at the centre of this loop B. The same is now bent to form a circular loop of smaller radius of have four turns in the loop. The magnetic field at the centre of this new loop is
View SolutionA current I flows along a round circular loop of radius R. Find the line integration of magnetic field along the axis of the loop from center to ∞
View SolutionA film of soap solution is formed on a loop frame loop of 6.28cm long thread is gently put on the film and the film is broken with a needle inside the loop. The tread loop takes the shape of a circle. Find the tension in the thread. Surface tension of soap solution =0.030n/m.
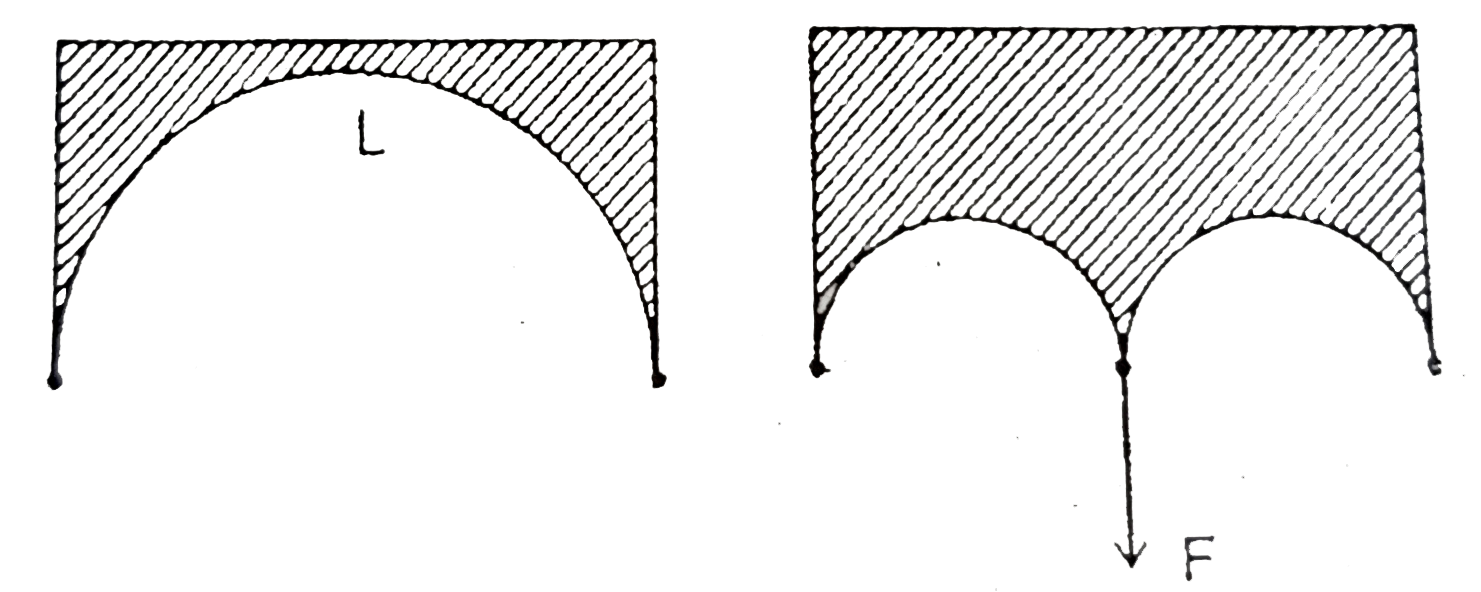
View SolutionA thin and light thread of sufficient length L is attached to a wire frame. The frame (along with the thread) is dipped into soap solution. When the frame is taken out of the solution, the thread takes the form of a semicircle with a soap film extending between the frame and the thread as shown in the figure (a). Now the thread is deformed into two semicircles by applying a force of F at the middle of the thread, as a result the film gets strectched as shown in the figure. (b). Calculate the surface tension of the soap solution.
View SolutionA square loop of sides L is placed at centre of a circular loop of radius r (r << L) such that they are coplanar, then mutual inductance between both loop is
View Solution
