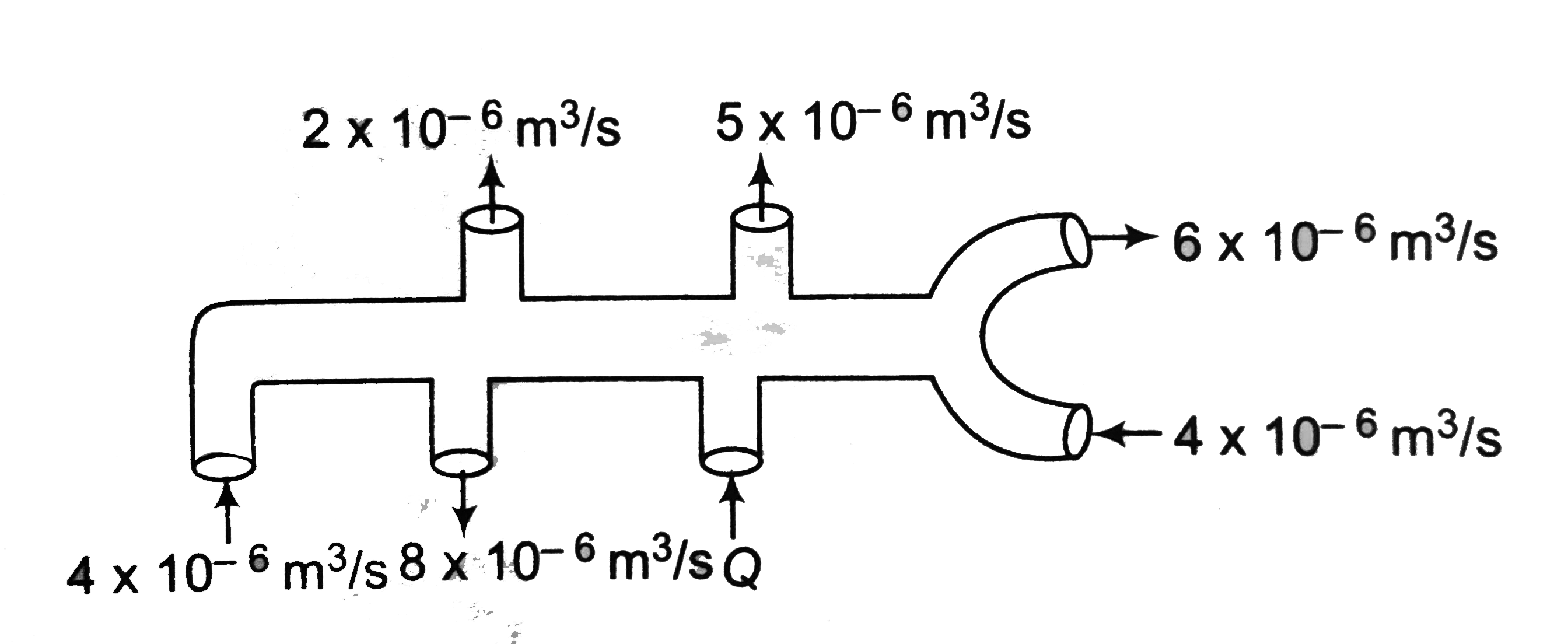
The pipe shows the volume flow rate of an ideal liquid at certain time and its direction. What is the value of Q in m3/s? (Assume steady state and equal area of cross section at each opening)
10×10−6
11×10−6
13×10−6
18×10−6
The correct Answer is:C
(σQ)inflow=(σQ)outflow
∴(4×10−6)+Q+(4×10−6)
=(8×10−6)+(2×10−6)+(5×10−6)
+(6×10−4)
∴Q=13×10−6m3/s.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Subjective
36 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion and Reason
1 Video
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
Two very large open tanks A&F both contain the same liquid. A horizontal pipe BCD, having a small constriction at C, leads out of the bottom of tank A, and a vertical pipe E containing air opens into the constriction at C and dips into the liquid in tank F. Assume stremline flow and no viscosity. If the cross section area at C is one-half that at D, and if D is at distance h1 below the level of the liquid in A, to what height h2 will liquid rise in pipe E? Express your answer in terms of h1.
[Neglect changes in atmosphere pressure with elevation. In the containers there is atmosphere above the water surface and D is also open to atmosphere.]

View Solution(a) What is meant by the principle of continuity ? Show that velocity of a liquid is inversely proportional to the area of cross-section of a pipe or tube.
View Solution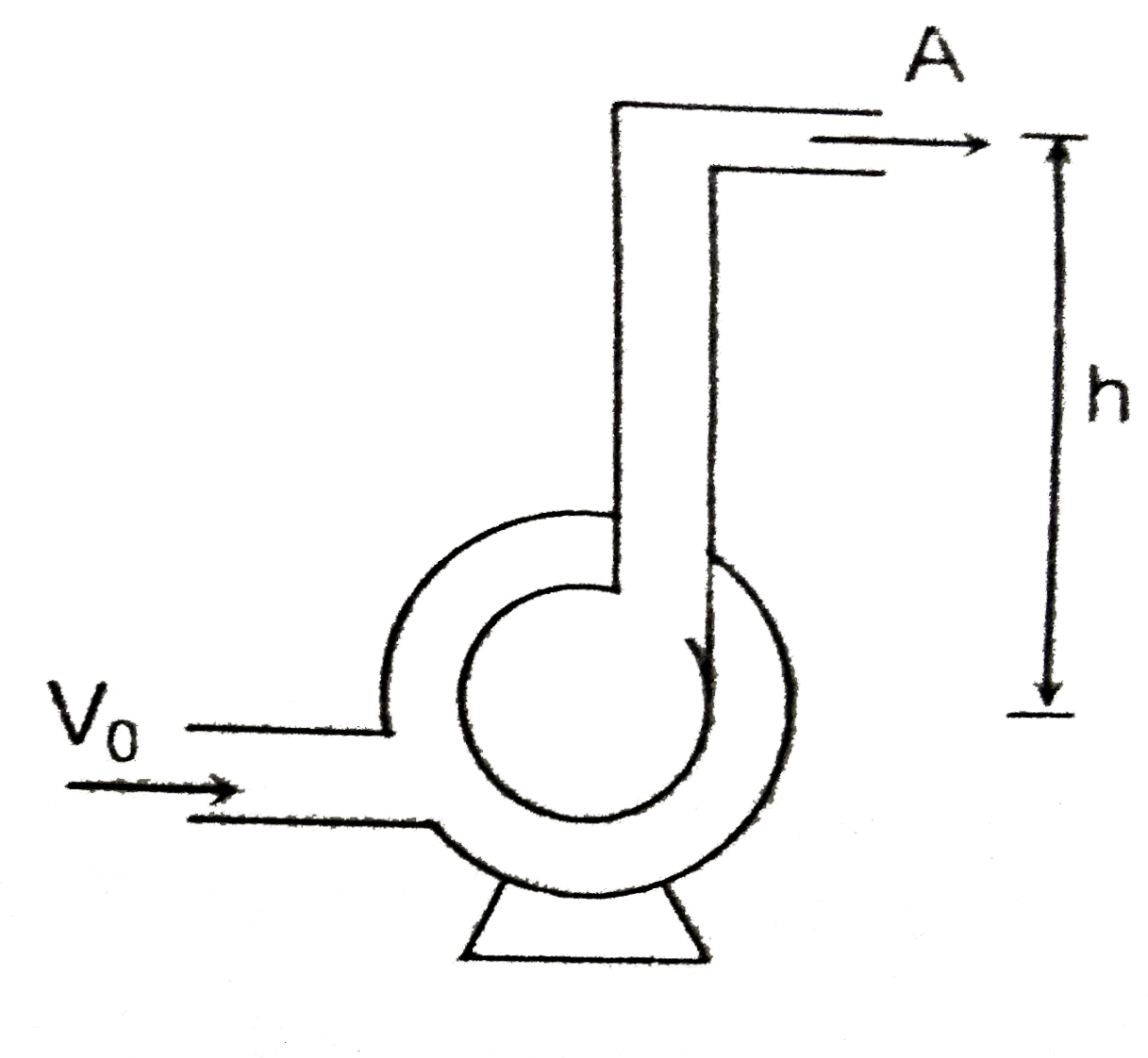
In a certain pump whose rated power is P. ideal fluid of denstiy ρ enters with speed V0 which lifts water upto height h through a pipe of uniform cross section area without discontinuity. Volumetric flow rate of the liquid is Q. Speed of fluid when it comes out of the pipe at point A is (Assume that pump works at 100 % efficiency and flow is streamline)
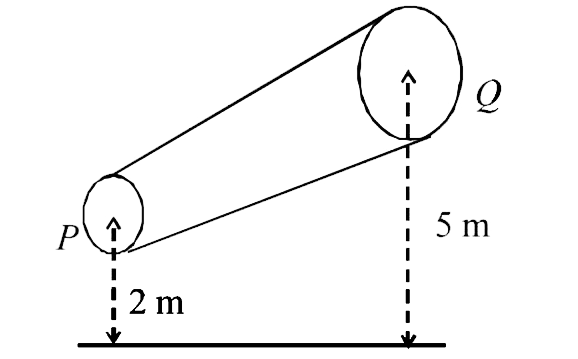
View SolutionA non-viscous liquid of constant density 1000kg/m3 flows in a streamline motion along a tube of variable cross section. The tube is kept inclined in the vertical plane as shown in Figure. The area of cross section of the tube two point P and Q at heights of 2 metres and 5 metres are respectively 4×10−3m2 and 8×10−3m2. The velocity of the liquid at point P is 1m/s. Find the work done per unit volume by the pressure and the gravity forces as the fluid flows from point P to Q.
View SolutionVolume of a cube is increasing at the rate of 9cm3/s if the length of its side is equal to 10 cm than at what rate its surface area is increasing?
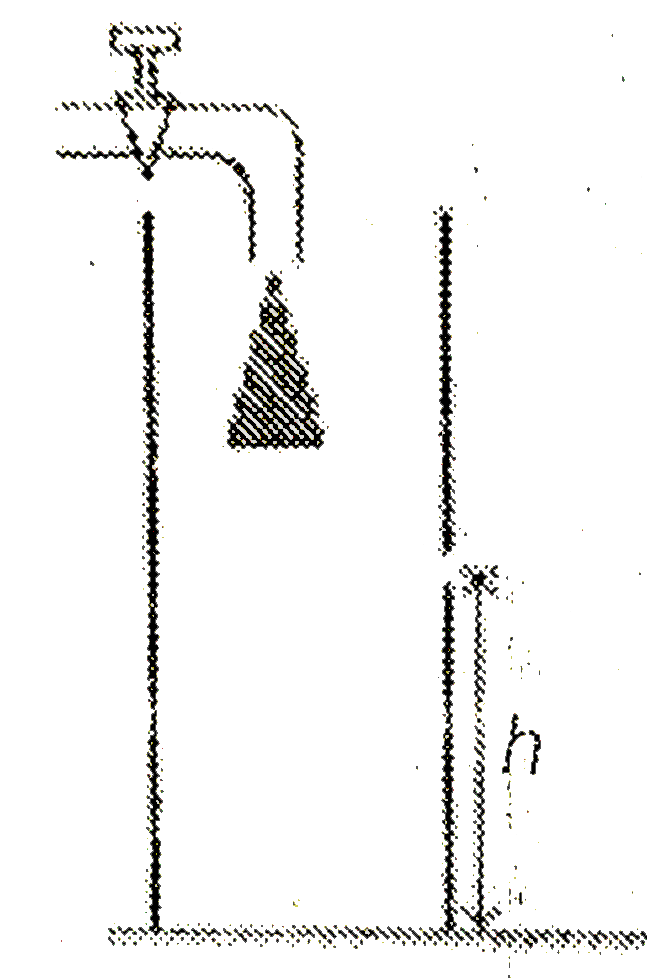
View SolutionThe diagram shows a tall cylindrical container kept on a horizontal surface. From a tap, water come with constant rate qm3/s. Initially the container was empty. An orifice of area of cross section A is made at some height h in the side wall of the container. If the horizontal range of the water jet in steady is found to be 2h, then the relation between h and q is
View SolutionFigure shows a liquid flowing through a tube at the rate of 0.1m3/s. The tube is branched into two semicircular tubes of cross-sectional area A/3 and 2A/3. The velocity of liquid at Q is (the cross section of the main tube is A=10−2m2 and vp=20m/s)
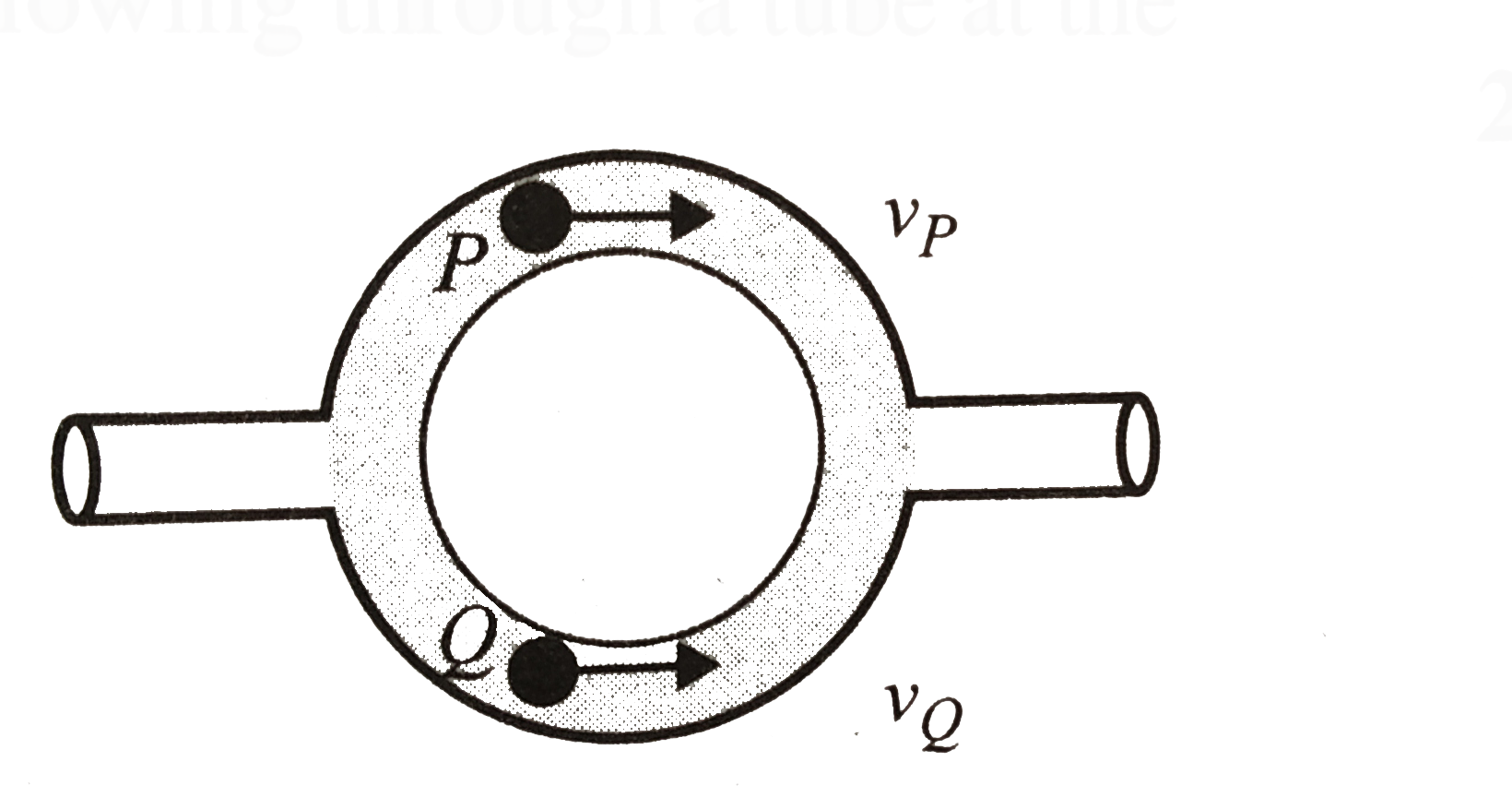
View SolutionA horizontal pipe of varying cross-sectional area carries water flowing at a constant rate. The pressure at sections (1) and (2) are 50cm and 20cm of Hg. If the velocity of section (1) is 5m/s, what is the velocity of section (2). Also determine the ratio of areas at sections (1) and (2) (as shown in Fig.
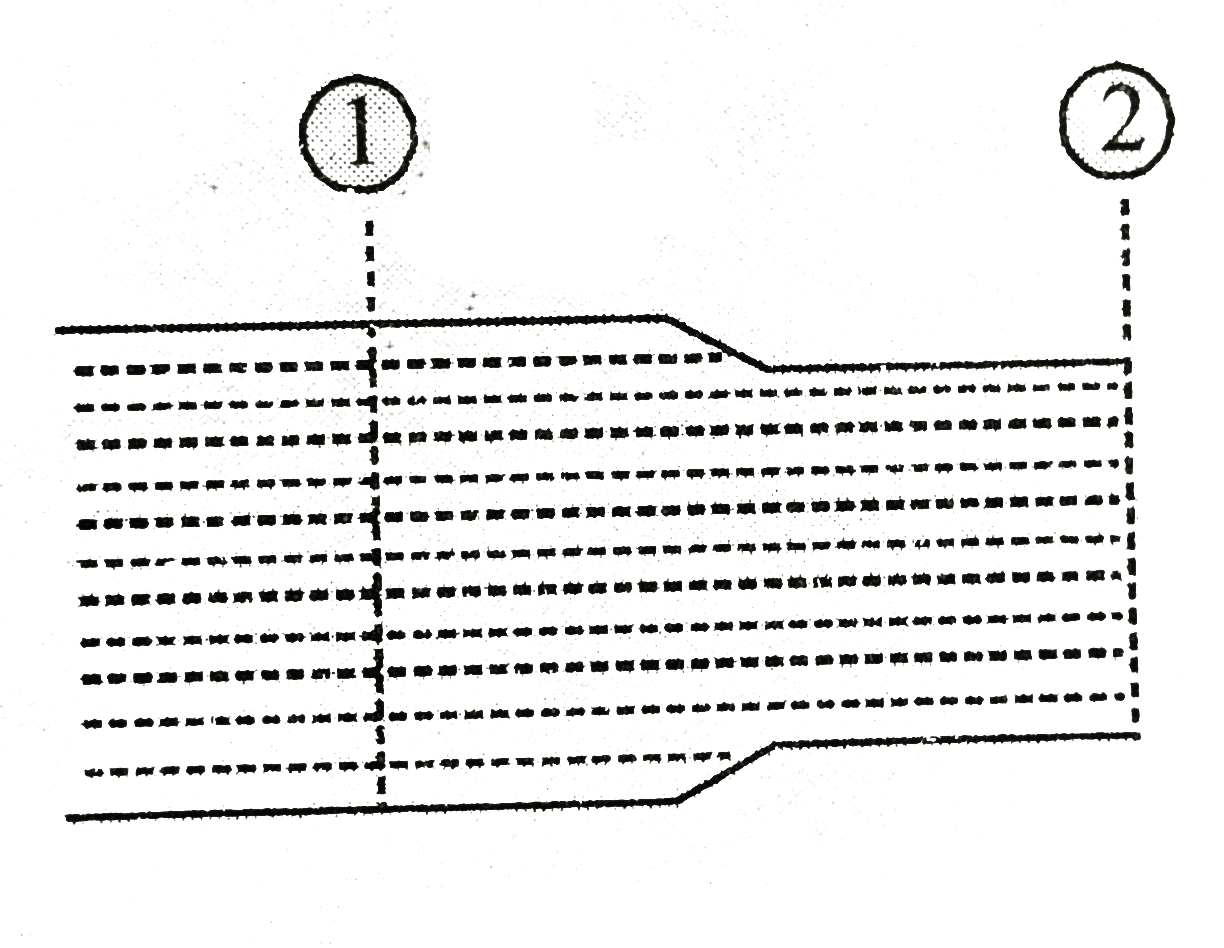
View SolutionA hose-pipe of cross-section area 2cm2 delivers 1500 litres of water in 5 minutes. What is the speed of water in m/s through the pipe?
View SolutionA "flow calorimeter" is used to measure the specific heat of a liquid. Heat is added at a known rate to a stream of the liquid as it passes through the calorimeter at known rate, then a measurement of the resulting temperature difference between the in flow and the out flow points of the liquid stream enables us to compute the specific heat of the liquid. A liquid of density 0.85 g/cm3 flows through a calorimeter at the rate of 8.0 cm3/s. heat is added by means of a 250 watt electric heating coil, and a temperature difference of 15∘C is established in steady state conditions between the in flow and out flow points.
Q. Rate of heat absorption per unit specific heat capacity is
View Solution