Find the moment of inertia of a uniform square plate of mass M and edge a about one of its diagonals.
To find the moment of inertia of a uniform square plate of mass M and edge a about one of its diagonals, we can follow these steps:
Step 1: Understand the Geometry
We have a uniform square plate with mass M and edge length a. The square plate has two diagonals, and we need to find the moment of inertia about one of these diagonals.
Step 2: Moment of Inertia about an Axis Perpendicular to the Plate
The moment of inertia of a square plate about an axis perpendicular to the plate and passing through its center is given by the formula:
Iperpendicular=16Ma2
Step 3: Apply the Perpendicular Axis Theorem
The perpendicular axis theorem states that for a planar body, the moment of inertia about an axis perpendicular to the plane (let's call it Iz) is equal to the sum of the moments of inertia about two perpendicular axes in the plane of the body (let's call them Ix and Iy):
Iz=Ix+Iy
For a square plate, since it is symmetric, we have Ix=Iy. Therefore, we can write:
Iz=2Ix
Step 4: Substitute the Known Values
From the earlier step, we know:
Iz=16Ma2
Substituting this into the equation gives:
16Ma2=2Ix
Step 5: Solve for Ix
Now, we can solve for Ix:
Ix=12(16Ma2)=112Ma2
Step 6: Moment of Inertia about the Diagonal
Since Ix is the moment of inertia about an axis parallel to one of the sides of the square, and we want the moment of inertia about one of the diagonals, we can use the relationship:
Idiagonal=Ix+Iy
However, since Ix=Iy and both are equal to 112Ma2, we find:
Idiagonal=112Ma2+112Ma2=16Ma2
Final Result
Thus, the moment of inertia of the uniform square plate about one of its diagonals is:
Idiagonal=16Ma2
We have a uniform square plate with mass M and edge length a. The square plate has two diagonals, and we need to find the moment of inertia about one of these diagonals.
The moment of inertia of a square plate about an axis perpendicular to the plate and passing through its center is given by the formula:
Iperpendicular=16Ma2
The perpendicular axis theorem states that for a planar body, the moment of inertia about an axis perpendicular to the plane (let's call it Iz) is equal to the sum of the moments of inertia about two perpendicular axes in the plane of the body (let's call them Ix and Iy):
Iz=Ix+Iy
For a square plate, since it is symmetric, we have Ix=Iy. Therefore, we can write:
Iz=2Ix
From the earlier step, we know:
Iz=16Ma2
Substituting this into the equation gives:
16Ma2=2Ix
Now, we can solve for Ix:
Ix=12(16Ma2)=112Ma2
Since Ix is the moment of inertia about an axis parallel to one of the sides of the square, and we want the moment of inertia about one of the diagonals, we can use the relationship:
Idiagonal=Ix+Iy
However, since Ix=Iy and both are equal to 112Ma2, we find:
Idiagonal=112Ma2+112Ma2=16Ma2
Thus, the moment of inertia of the uniform square plate about one of its diagonals is:
Idiagonal=16Ma2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Single Correct
26 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Multiple Correct
36 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Objective
30 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
Knowledge Check
Find the momentof inertia of a uniform square plate of mass m and edge a about one of its diagonals.
AMl26BMl212CMl23DMl24
Find the moment of inertia of a uniform ring of mass M and radius R about a diameter.
View SolutionFind the moment of inertia of a uniform rectangular plate of mass M and edges of length 'I' and 'b' about its axis passing through the centre and perpendicular to it.
View SolutionThe moment of inertia of a cube of mass m and side a about one of its edges is equal to
View SolutionFind the moment of inertia of a uniform sphere of mass m and radius R about a tangent if the spheres (1) solid (ii) hollow?
View SolutionThe moment of inertia of a uniform solid regular tetrahedron of mass m and edge a, about its symmetry axis (i.e and axis passing through on evertex and the centre of the opposite face) is:
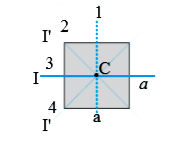
View SolutionIn the figure shown find the moment of inertia of square plate having mass m and sides a. About an axis 2 passing through point C (centre of mass) and in the plane of plate?
View SolutionMoment of inertia of a circular wire of mass M and radius R about its diameter is
View Solution