A copper cylindrical tube has inner radius a and outer radius b. The resistivity is p. The resistance of . the cylinder between the two ends is
ρlb2−a2
ρl2π(b−a)
ρlπ(b2−a2)
π(b2−a2)ρl
The correct Answer is:C
To find the resistance of a copper cylindrical tube with inner radius a, outer radius b, and resistivity ρ, we can follow these steps:
1. Understand the Geometry:
The cylindrical tube has two radii: an inner radius a and an outer radius b. The length of the cylinder is denoted as L.
2. Resistance Formula:
The resistance R of a conductor is given by the formula:
R=ρLA
where ρ is the resistivity, L is the length of the conductor, and A is the cross-sectional area.
3. Determine the Cross-Sectional Area:
Since we have a hollow cylinder, the cross-sectional area A is the area of the outer circle minus the area of the inner circle:
A=πb2−πa2
This can be factored as:
A=π(b2−a2)
4. Substituting the Area into the Resistance Formula:
Now, substitute the expression for the area A back into the resistance formula:
R=ρLπ(b2−a2)
5. Final Expression for Resistance:
Therefore, the resistance of the cylindrical tube between the two ends is:
R=ρLπ(b2−a2)
The cylindrical tube has two radii: an inner radius a and an outer radius b. The length of the cylinder is denoted as L.
The resistance R of a conductor is given by the formula:
R=ρLA
where ρ is the resistivity, L is the length of the conductor, and A is the cross-sectional area.
Since we have a hollow cylinder, the cross-sectional area A is the area of the outer circle minus the area of the inner circle:
A=πb2−πa2
This can be factored as:
A=π(b2−a2)
Now, substitute the expression for the area A back into the resistance formula:
R=ρLπ(b2−a2)
Therefore, the resistance of the cylindrical tube between the two ends is:
R=ρLπ(b2−a2)
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:NCERT FINGERTPS ENGLISHChapter:CURRENT ELECTRICITYExercise:CELLS, EMF, INTERNAL RESISTANCE
Explore 6 Videos
- CURRENT ELECTRICITYBook:NCERT FINGERTPS ENGLISHChapter:CURRENT ELECTRICITYExercise:KIRCHOFF S LAW
Explore 14 Videos
- CURRENT ELECTRICITYBook:NCERT FINGERTPS ENGLISHChapter:CURRENT ELECTRICITYExercise:ELECTRICAL ENERGY, POWER
Explore 8 Videos
- COMMUNITCATION SYSTEMSBook:NCERT FINGERTPS ENGLISHChapter:COMMUNITCATION SYSTEMSExercise:Assertion And Reason
Explore 30 Videos
- DUAL NATURE OF RADIATION AND MATTER Book:NCERT FINGERTPS ENGLISHChapter:DUAL NATURE OF RADIATION AND MATTER Exercise:Assertion And Reason
Explore 15 Videos
Similar Questions
A cylindrical tube of length l has inner radius a while outer radius b. What is the resistance of the tube between (a) its ends (b) inner and outer surfaces ? (The resistivitiy of its material is p)
View SolutionA cylinderical tube of length L has inner radius a and outer radius b. What is the resistance of the tube between its inner and outer surface ? Given that the resistivity of its material is ρ.
View SolutionSphere of inner radius a and outer radius b is made of p uniform resistivity find resistance between inner and outer surface
View SolutionSphere of inner radius a and outer radius b is made of p uniform resistivity find resistance between inner and outer surface
View SolutionA coaxial cable is made up of two conductors. The inner conductor is solid is of radius R1 and the outer coductor is hollow of inner radius R2 and outer radius R3 . The space between the conductors are carrying currents of equal magnitudes and in oppsite direactions. then the variation of magnetic field with distance form the axis is best plotted as :

View SolutionA cylinder of radius R is surrounded by a cylindrical shell of inner radius R and oputer radius 2R. The thermal conductivity of the material of the inner cylinder is k1 and that of the outer cylinder is K2 ,Assumming no loss of heat , the effective thermal conductivity of the system for heat flowing along the length of the cylinder is :
View SolutionA current i is uniformly distributed over the cross section of a long hollow cylinderical wire of inner radius R1 and outer radius R2. Magnetic field B varies with distance r form the axis of the cylinder is
View Solution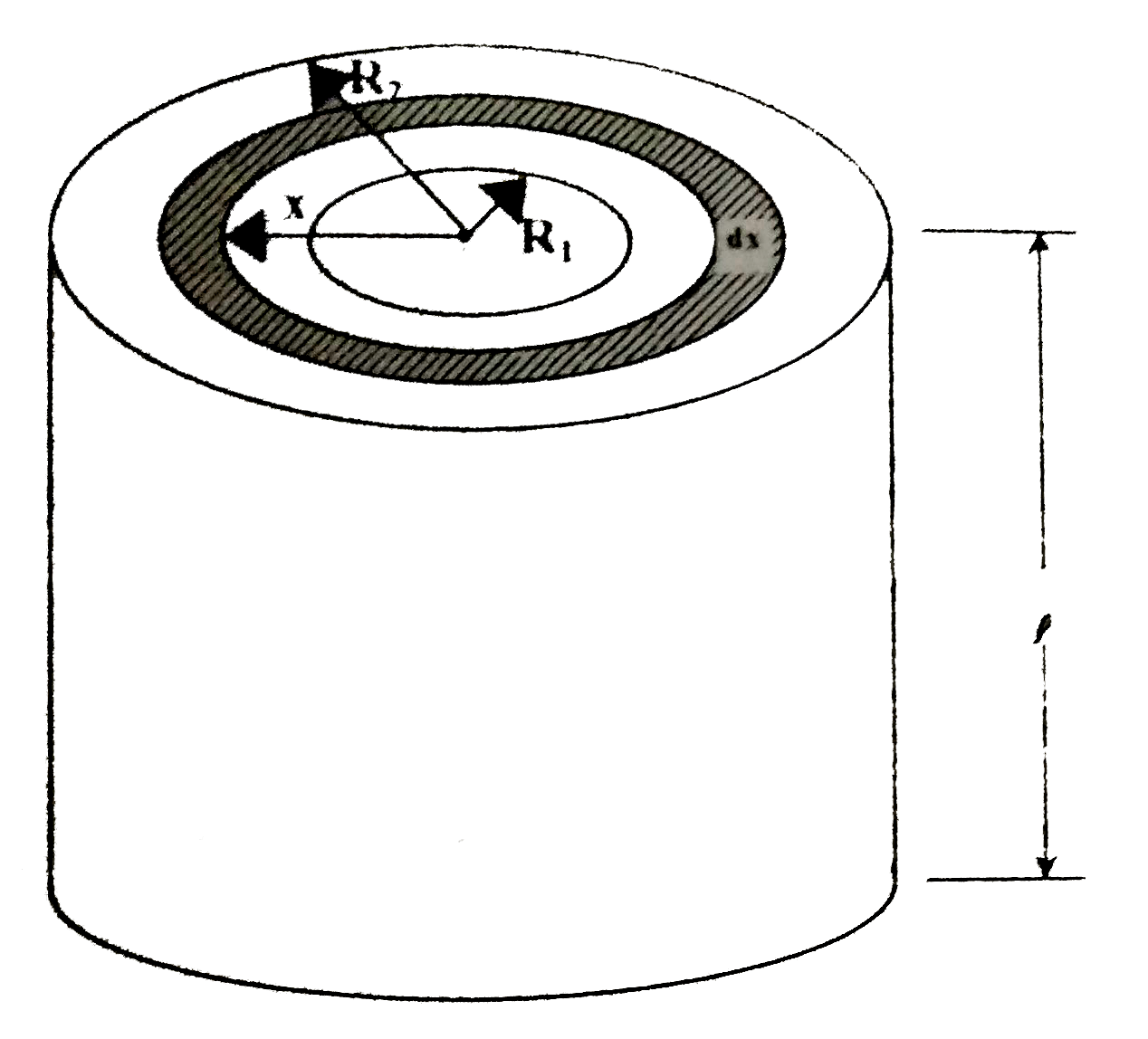
A cylindrical conductor of length l and inner radius R1 and outer radius R2 has specific resistance ρ. A cell of emf ε is connected across the two lateral faces of the conductor. Find the current drown from the cell.
View SolutionA circular running track has inner radius 126 m and outer radius 140 m . Find the area of the track .
View SolutionLet the moment of inertia of a hollow cylinder of length 30 cm (inner radius 10 cm and outer radius 20 cm ), about its axis be I. the radius of a thin cylinder of the same about its axis is also I is :
View Solution