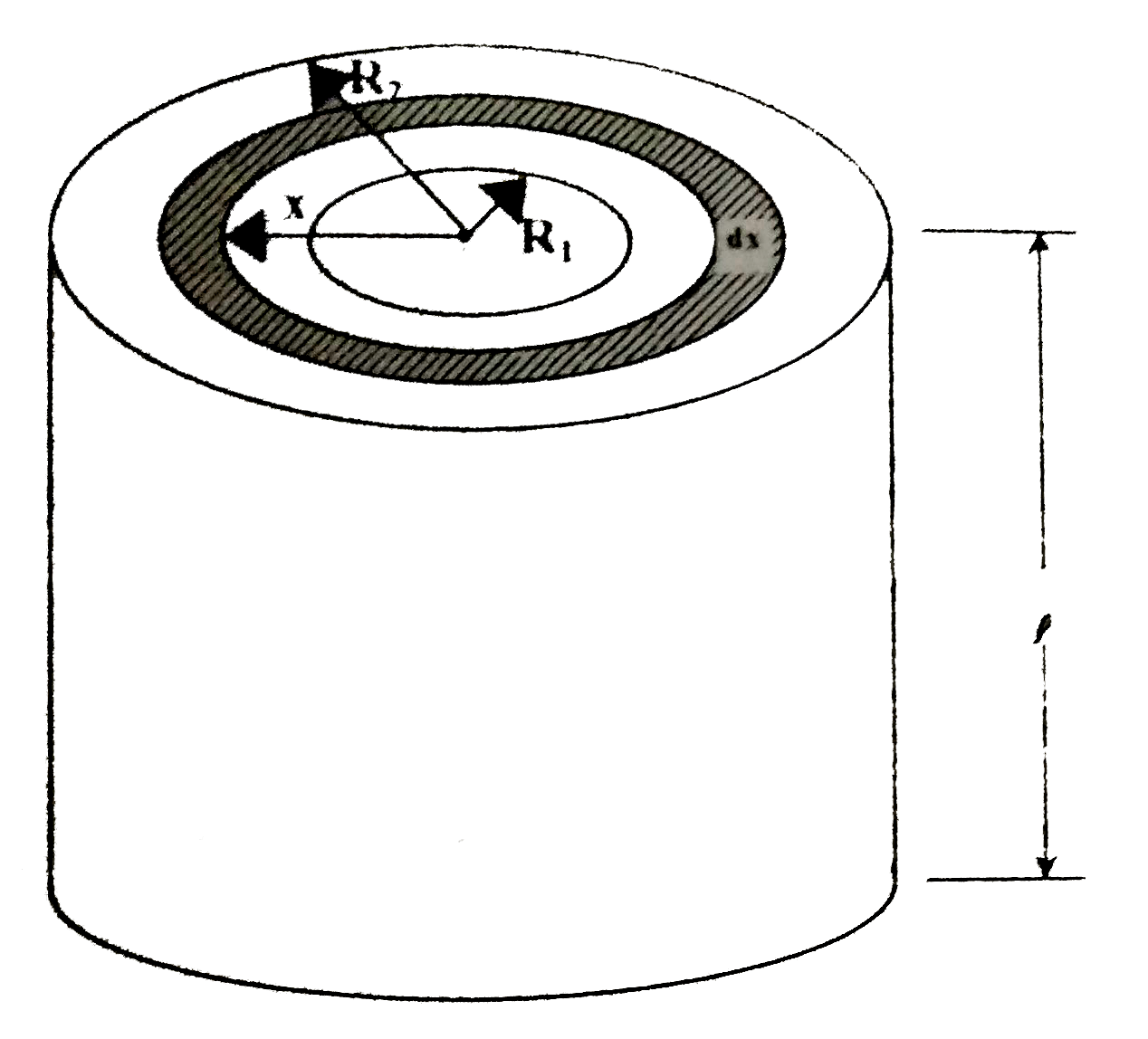
A cylindrical conductor of length l and inner radius $R_{1}$ and outer radius $R_{2}$ has specific resistance $ρ$ . A cell of emf $ε$ is connected across the two lateral faces of the conductor. Find the current drown from the cell.

$\frac{2 π l ε}{ρ In \frac{R_{2}}{R_{1}}}$

$\frac{2 π l ε}{ρ (R_{2}^{2} - R_{1}^{2})}$

$\frac{2 l ε}{ρ π (R_{2}^{2} - R_{1}^{2})}$

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Consider the differential element of the cylinder as shown in the figure.
$∴ R = R_{2} \int R_{1} ρ \frac{d x}{2 π x l} (∵ R = ρ \frac{l}{a})$
$\Rightarrow R = \frac{ρ}{2 π l} In (R_{2} / R_{1})$
$I = \frac{ε}{R}, \Rightarrow I = \frac{2 π / ε}{ρ In (\frac{R_{2}}{R_{1}})}$

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Similar Questions

A cylinderical tube of length L has inner radius a and outer radius b. What is the resistance of the tube between its inner and outer surface ? Given that the resistivity of its material is $ρ$ .
View Solution
A cylindrical conductor of length $l$ and uniform area of cross-section $A$ has resistance $R$ . Another conductor of length $2 l$ and resistance $R$ of the same material has area of cross-section :
View Solution
A cylindrical tube of length l has inner radius a while outer radius b. What is the resistance of the tube between (a) its ends (b) inner and outer surfaces ? (The resistivitiy of its material is p)
View Solution
The hollow cylinder of length l and inner and outer radius $R_{1}$ and $R_{2}$ respectively. Find resistance of cylinder if current flows radially outward in the cylinder. Resistivity of material of cylinder id $ρ$ .
View Solution
A metallic spherical shell has an inner radius $R_{1}$ and outer radius $R_{2}$ . A charge is placed at the centre of the spherical cavity. The surface charge density on the inner surface is

View Solution
A cell of emf $ε$ and internal resistance r is connected across a load resistance R
(i) Find the maximum power delivered at the load ?
.
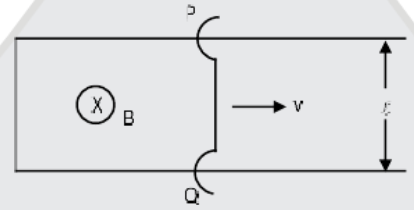
View Solution
A rod having length l and resistance $R$ is moving with velocity v on a $π$ shape conductor. Find the current in the rod.
View Solution
A conductor of length $l$ has a non-uniform cross-section. The radius of cross-section varies linearly from $a$ to $b$ . The resistivity of the material is $ρ$ . Find the resistance of the conductc across its ends.

View Solution
A metal spherical shell has an inner radius $R_{1}$ and outer radius $R_{2}$ . A charge Q is placed at the center of the spherical cavity. What will be surface charge density on (i) the inner surface, and (ii) the outer surface ?
View Solution
A coaxial cable made up of two conductors. The inner conductor is solid and is of radius $R_{1}$ and the outer conductor is hollow of inner radius $R_{2}$ and outer radius $R_{3}$ . The space between the conductors is filled with air. The inner and outer conductors are carrying currents of equal magnitudes and in opposite directions. Then the variation of magnetic field with distance from the axis is best plotted as
View Solution

A cylindrical conductor of length l and inner radius R1 and outer radius R2 has specific resistance ρ. A cell of emf ε is connected across the two lateral faces of the conductor. Find the current drown from the cell.

The correct Answer is:A

Consider the differential element of the cylinder as shown in the figure. ∴R=R2∫R1ρdx2πxl (∵R=ρla) ⇒R=ρ2πlIn(R2/R1) I=εR,⇒I=2π/ερIn(R2R1)

Similar Questions

Recommended Questions

A cylindrical conductor of length l and inner radius $R_{1}$ and outer radius $R_{2}$ has specific resistance $ρ$ . A cell of emf $ε$ is connected across the two lateral faces of the conductor. Find the current drown from the cell.

Consider the differential element of the cylinder as shown in the figure.
$∴ R = R_{2} \int R_{1} ρ \frac{d x}{2 π x l} (∵ R = ρ \frac{l}{a})$
$\Rightarrow R = \frac{ρ}{2 π l} In (R_{2} / R_{1})$
$I = \frac{ε}{R}, \Rightarrow I = \frac{2 π / ε}{ρ In (\frac{R_{2}}{R_{1}})}$