Find the capacitance of an isolated ball-shaped conductor of radius R1 surrounded by an adjacent concentric layer of dielectric with permittivity ε and outside radius R2.
Let us mentally inmpart a charge q on the conductor, then
varhπ+−φ−=∫R2R1q4πε0εr2dr+∫∞R2q4πε0r2dr
=q4πε0ε[1R1−1R2]+q4πε01R2
=q4πε0ε[(ε−1)R2+1R1]
Hence the sought capacitance,
C=qφ+−φ−=q4πε0εq[(ε−1)R2+1R1]=4πε0εR1(ε−1)R1R2+1

Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Current
72 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics
69 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Conductors And Dielectrics In An Electric Field
47 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
Knowledge Check
The capacitance of an isolated conducting sphere of radius R is proportional to
A4πε0rB2πε0rCπε0rD8πε0rThe capacitance of an isolated conducting sphere of radius R is proportional to
AR−1BR2CR−2DRThe capacitance of earth of radius R treating as spherical conductor is
A4π∈0RB4πRC∈0RD4π∈0R
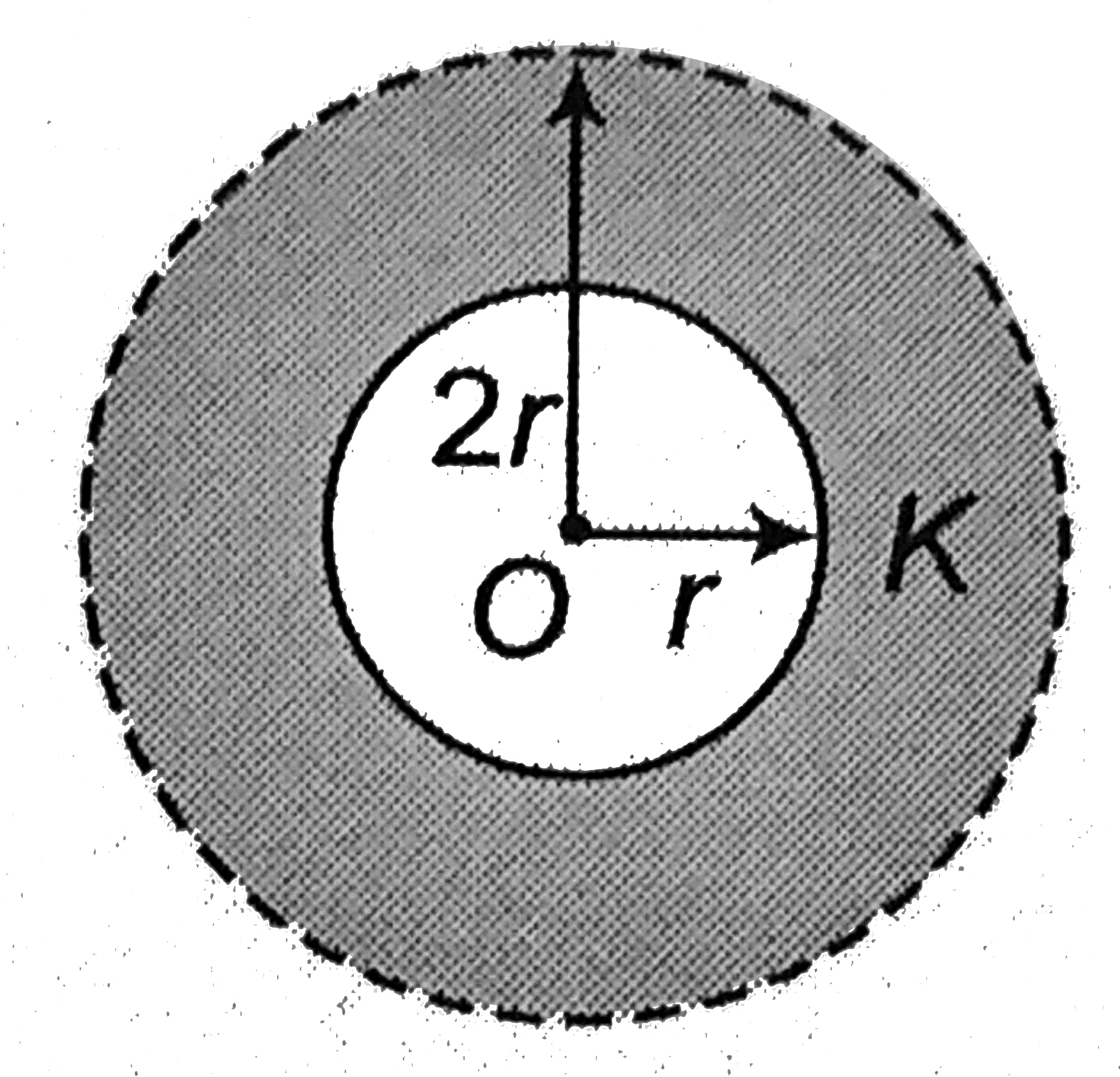
Find the capacitance of an isolated spherical conductor of radius a surrounded by a concentric layer of dielectric of outer radius b and relative permittivity. ε
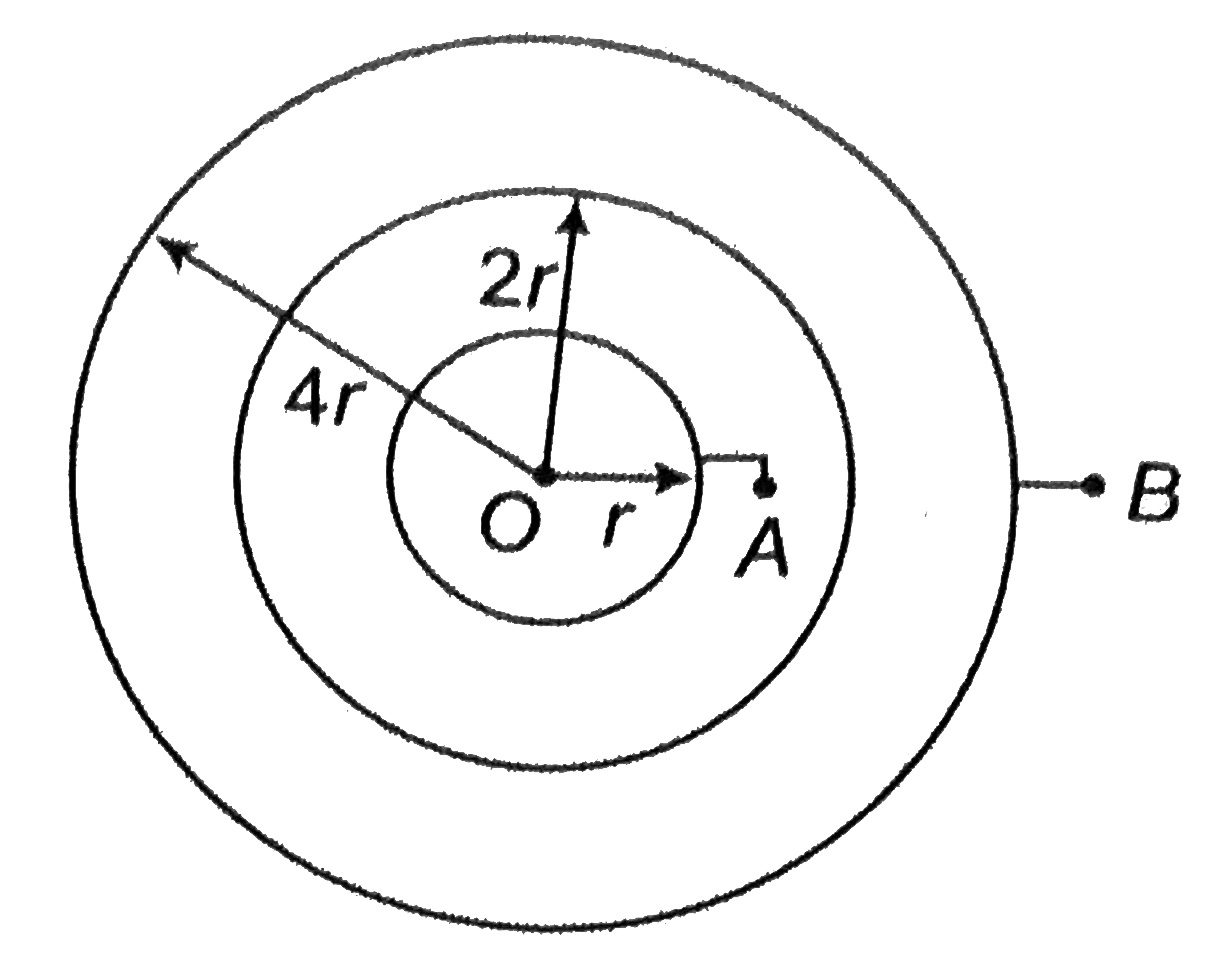
View SolutionFind the equivalent cappacitance between A and B.
(a) Three conducting concentic shells of radii r,2r and
(b) An isoltated ball-shaped conductor of radius r surrounded by an adjuacent layer of dielectric (K) and outer radius 2r.
Two conducting concentic spherical shells having radii r1 and r2(r2>r1), calculate capacity of the system if
(i) The inner shell is given a charge and outer is connected to the earth.
(ii) The outer shell is given a charge and inner is connected to the earth.
(d) Find capacitance of the system
(i) Two shells of radii r1 and r2 having charges are connected by a metallic wire, the separation between shells is large.
(ii) Two shells of radii r1 and r2 carry equal and opposite charges and are separated by a distance d.

View SolutionCapacitance of a spherical conductor of radius r is given as_____________ .
View SolutionA double-layer cylindrical capacitor consists of conductors of radii R1 and R3 and two layers of dielectrics of relative permittivtties ε1 and esπon2. The radius of the common surface of the dielectrics. Is R2 . The breakdown field strengths of the dielectrics are E1 and E2 respectively. What is the breakdown voltage of this capacitor if (a) ε1R1E1<ε2R2E2 (b)ε2R2E2>ε1E1R1?
[Hint: If E1=E1max and E2>,E2max dielectric 1 will break first and if E2=E2max'E1>E1max dielectric 2 will break first.]
View SolutionConducting sphere of radius R1 is covered by concentric sphere of radius R2. Capacity of this combination is proportional to
View Solution
