A uniform rod of mass m. length L, area of cross- secticn A is rotated about an axis passing through one of its ends and perpendicular to its length with constant angular velocity \(omega\) in a horizontal plane If Y is the Young's modulus of the material of rod, the increase in its length due to rotation of rod is
mω2L2AY
mω2L22AY
mω2L23AY
2mω2L2AY
The correct Answer is:C
Consider a small element element of length dx at a distance x from the axis of rotation.
Mass of the element dm=mLdx=μdx
where μ=mL
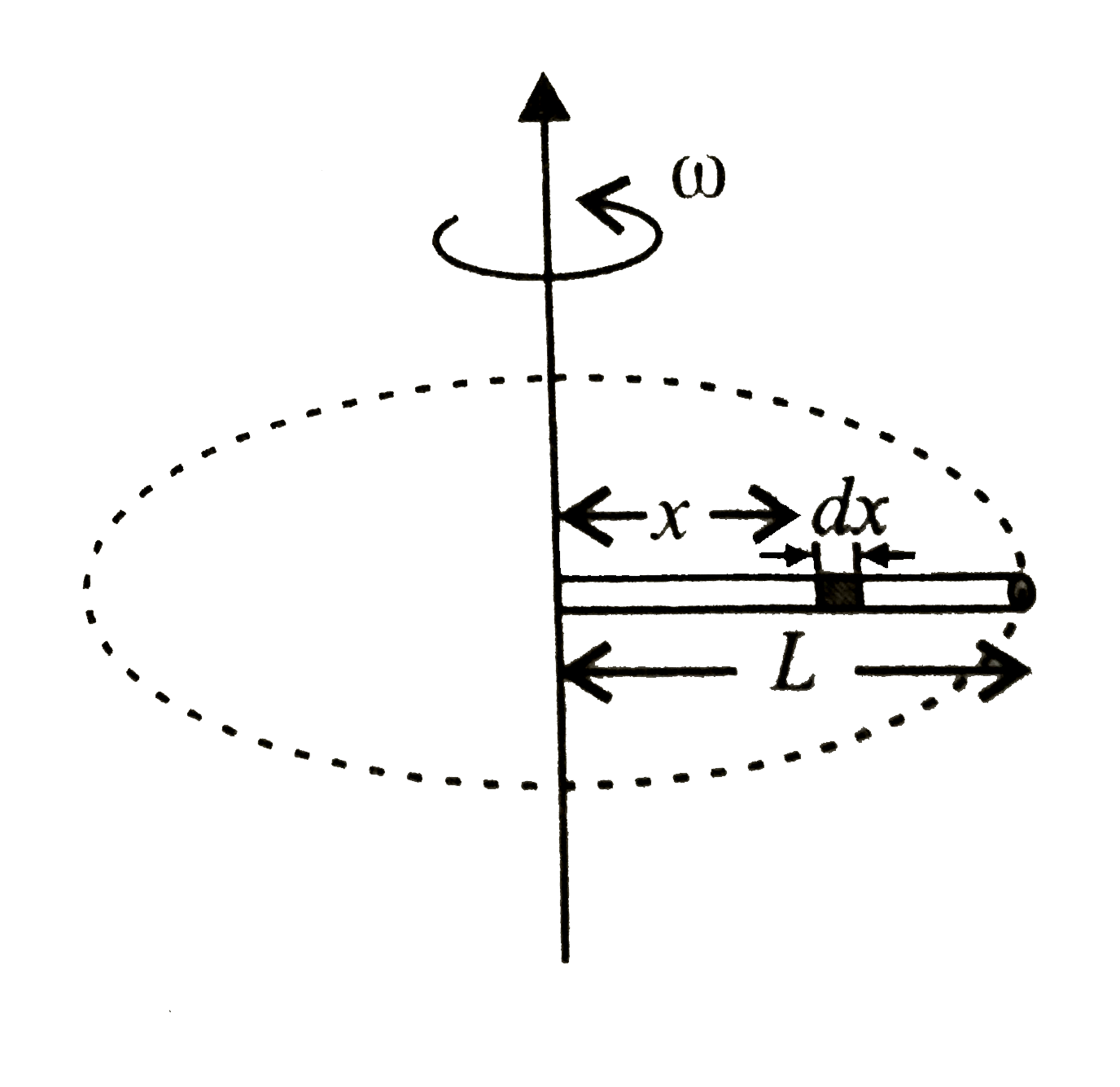
The centripetal force acting on the elment is dT=dmω2x=μω2xdx
As this force is provied by tensions in the rod (due to elasticity )so, the tension in the rod at distance x from the aixis of rotation will be due to the centripetal force due to all elements between x =x to x=L
∴T=L∫xμω2xdx=μω22[L2−x2] ....(i)
Let dl be increase in lengh of the element. Then
Y=T/Adl/dx
dl=TdxYA=μω22YA[L2−x2]dx [Using(i)]
So the total elnogation of the whole rod is l=L∫0μω22YA[L2−x2]dx=μω22YA[L2x−x33]L0=μω2L3YA=13mω2L2YA
Related Playlists
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - APPLICATIONS OF ELASTIC BEHAVIOUR OF MATERIALS8videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOTS8videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - STRESS-STRAIN CURVE5videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar5videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason15videos
MOTION IN A PLANE
Similar Questions
The radius of gyration of an uniform rod of length l about an axis passing through one of its ends and perpendicular to its length is.
View Solutionfind the radius of gyration of a rod of mass m and length 2l about an axis passing through one of its ends and perpendicular to its length.
View SolutionA stell rod of length 2l corss sectional area A and mass M is set rotating in a horizotnal plane about an axis passing through its cnetre and perpendicular to its length with constant angular velcoty ω. If Y is the Young's modulus for steel, find the extension in the lenght of the rod. (Assume the rod is uniform.)
View SolutionMoment of inertia of a uniform rod of length L and mass M, about an axis passing through L/4 from one end and perpendicular to its length is
View SolutionMoment of inertia of a uniform rod of length L and mass M, about an axis passing through L/4 from one end and perpendicular to its length is
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionMoment of inertia of a thin rod of mass m and length l about an axis passing through a point l/4 from one end and perpendicular to the rod is
View SolutionA non-conducting rod AB of length l has total charge Q . The rod is rotated about an axis passing through a point (l/3) distance from one end and perpendicular to plane of rod with constant angular speed omega, the magnetic moment of rod is
View SolutionA uniform rod of length l oscillates about an axis passing through its end. Find the oscillation period and the reduced length of this pendulum.
View SolutionA uniform rod of length l, mass m, cross-sectional area A and Young's modulus Y is rotated in horizontal plane about a fixed vertical axis passing through one end, with a constant angular velocity ω. Find the total extension in the rod due to the tension produced in the rod.
View Solution


