A block of mass m moving at a speed 'v' compresses as spring through a distance 'x' before its speed is halved. Find the spring constant of the spring.
The correct Answer is:B, C, D
To find the spring constant k of the spring when a block of mass m moving at speed v compresses the spring through a distance x before its speed is halved, we can use the principle of conservation of energy. Here’s the step-by-step solution:
Step 1: Write the initial kinetic energy
The initial kinetic energy KEi of the block when it is moving at speed v is given by:
KEi=12mv2
Step 2: Write the final kinetic energy
When the block compresses the spring by distance x, its speed is halved. Therefore, the final speed vb is:
vb=v2
The final kinetic energy KEf is then:
KEf=12mv2b=12m(v2)2=12mv24=18mv2
Step 3: Write the potential energy stored in the spring
The potential energy PE stored in the spring when it is compressed by distance x is given by:
PE=12kx2
Step 4: Apply the conservation of energy principle
According to the conservation of energy, the initial kinetic energy is equal to the sum of the final kinetic energy and the potential energy stored in the spring:
KEi=KEf+PE
Substituting the expressions we derived:
12mv2=18mv2+12kx2
Step 5: Simplify the equation
First, let's eliminate m from the equation (assuming m≠0):
12v2=18v2+12kx2
Now, multiply the entire equation by 8 to eliminate the fractions:
4v2=v2+4kx2
Step 6: Rearrange to solve for k
Subtract v2 from both sides:
4v2−v2=4kx2
3v2=4kx2
Now, isolate k:
k=3v24x2
Final Answer
The spring constant k is given by:
k=3mv24x2
The initial kinetic energy KEi of the block when it is moving at speed v is given by:
KEi=12mv2
When the block compresses the spring by distance x, its speed is halved. Therefore, the final speed vb is:
vb=v2
The final kinetic energy KEf is then:
KEf=12mv2b=12m(v2)2=12mv24=18mv2
The potential energy PE stored in the spring when it is compressed by distance x is given by:
PE=12kx2
According to the conservation of energy, the initial kinetic energy is equal to the sum of the final kinetic energy and the potential energy stored in the spring:
KEi=KEf+PE
Substituting the expressions we derived:
12mv2=18mv2+12kx2
First, let's eliminate m from the equation (assuming m≠0):
12v2=18v2+12kx2
Now, multiply the entire equation by 8 to eliminate the fractions:
4v2=v2+4kx2
Subtract v2 from both sides:
4v2−v2=4kx2
3v2=4kx2
Now, isolate k:
k=3v24x2
The spring constant k is given by:
k=3mv24x2
Topper's Solved these Questions
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -2
8 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
Similar Questions
A block of mass m moving at a speed v compresses a spring throgh a distance x before its speed is halved. Find the spring constant of the spring.
View SolutionA block of mass m moving at a speed v compresses a spring through a distance x before its speed is halved. The spring constant of the spring is 6mv2nx2. Find value of n.
View SolutionA block of 200 g mass is dropped from a height of 2 m on to a spring and compress the spring to a distance of 50 cm. The force constant of the spring is
View SolutionA block of mass m compresses a spring iof stifffness k through a distacne l/2 as shown in the figure .If the block is not fixed to the spring the period of motion of the block is

View SolutionA block of mass m is pushed up against a spring, compressing it a distance x, and the block is then released. The spring projects the block along a frictionaless horizontal surface, grving the block a speed v. The same spring projects a second block of mass 4m, giving it a speed 3v. What distance was the spring compressed in the second case ?
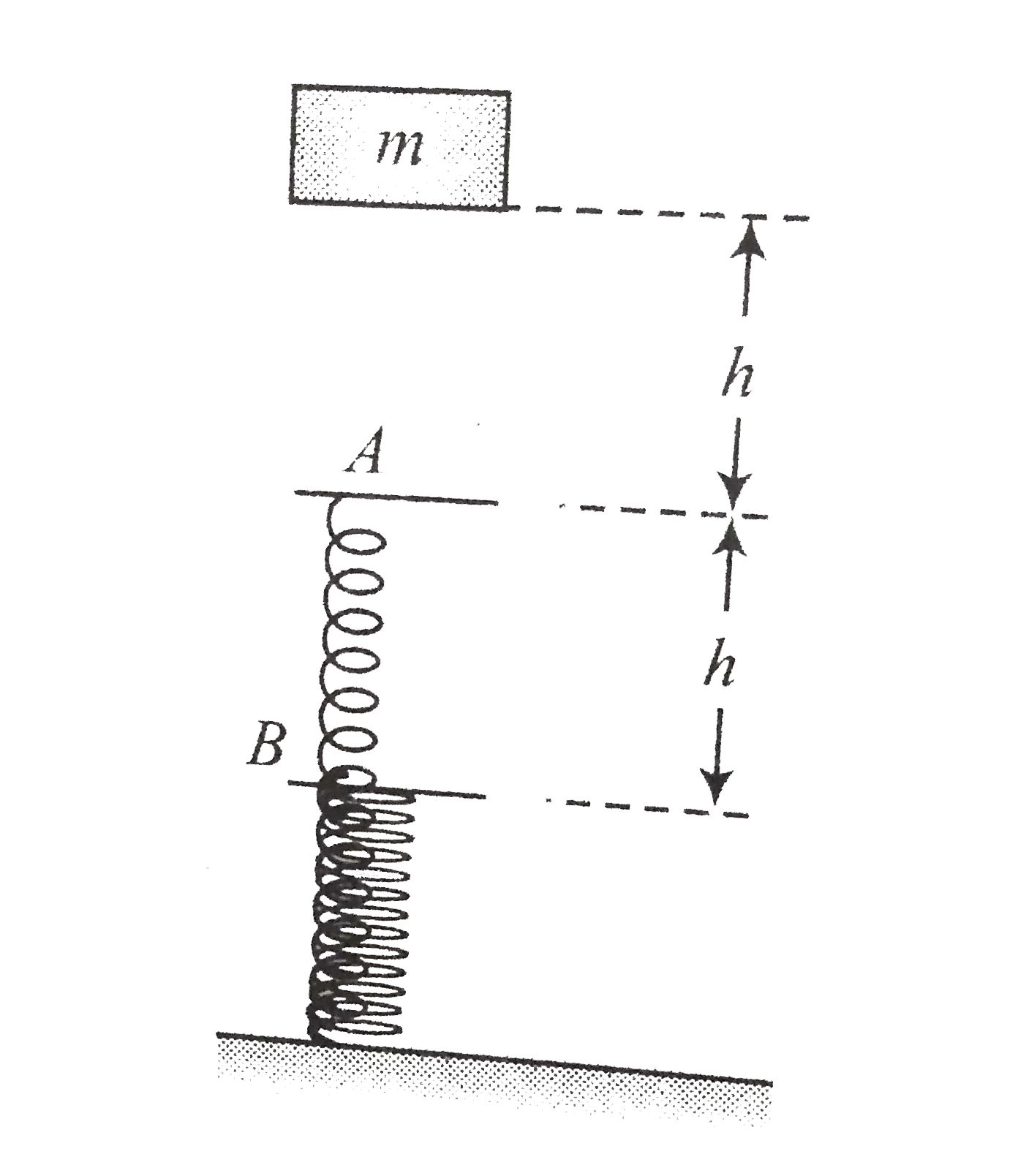
View SolutionA block of mass m strikes a light pan fitted with a vertical spring after falling through a distance h. If the stiffness of the spring is k, find the maximum compression of the spring.
View SolutionA block of mass m is released from a height h from the top of a smooth surface. There is an ideal spring of spring constant k at the bottom of the track. Find the maximum compression in the spring (Wedge is fixed)

View Solution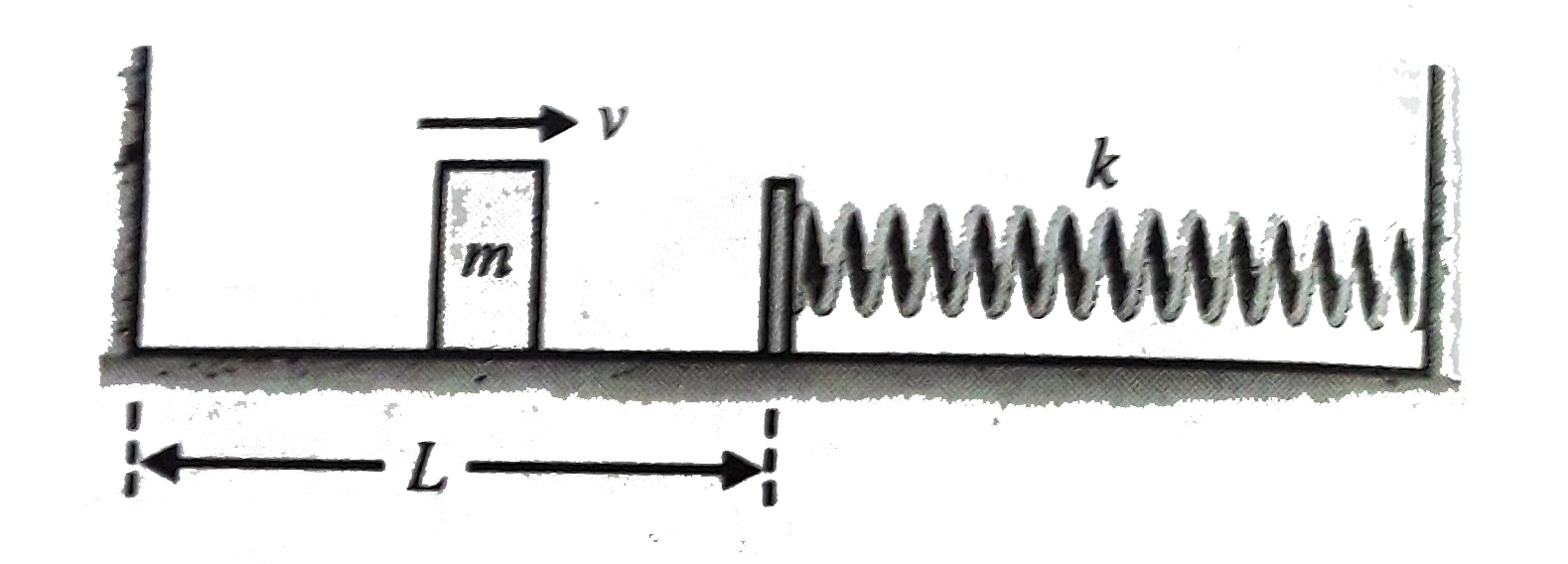
A spring having a spring constant k is fixed to a vertical wall as shown in Fig. A block of mass m moves with velocity v torards the spring from a parallel wall opposite to this wall. The mass hits the free end of the spring compressing it and is decelerated by the spring force and comes to rest and then turns the spring is decelerated by the spring force and comes to rest and then turns back till the spring acquires its natural length and contact with the spring is broken. In this process, it regains its angular speed in the opposite direction and makes a perfect elastice collision on the opposite left wall and starts moving with same speed as before towards right. The above processes are repeated and there is periodic oscillation.
Q. What is the time period of oscillation ?
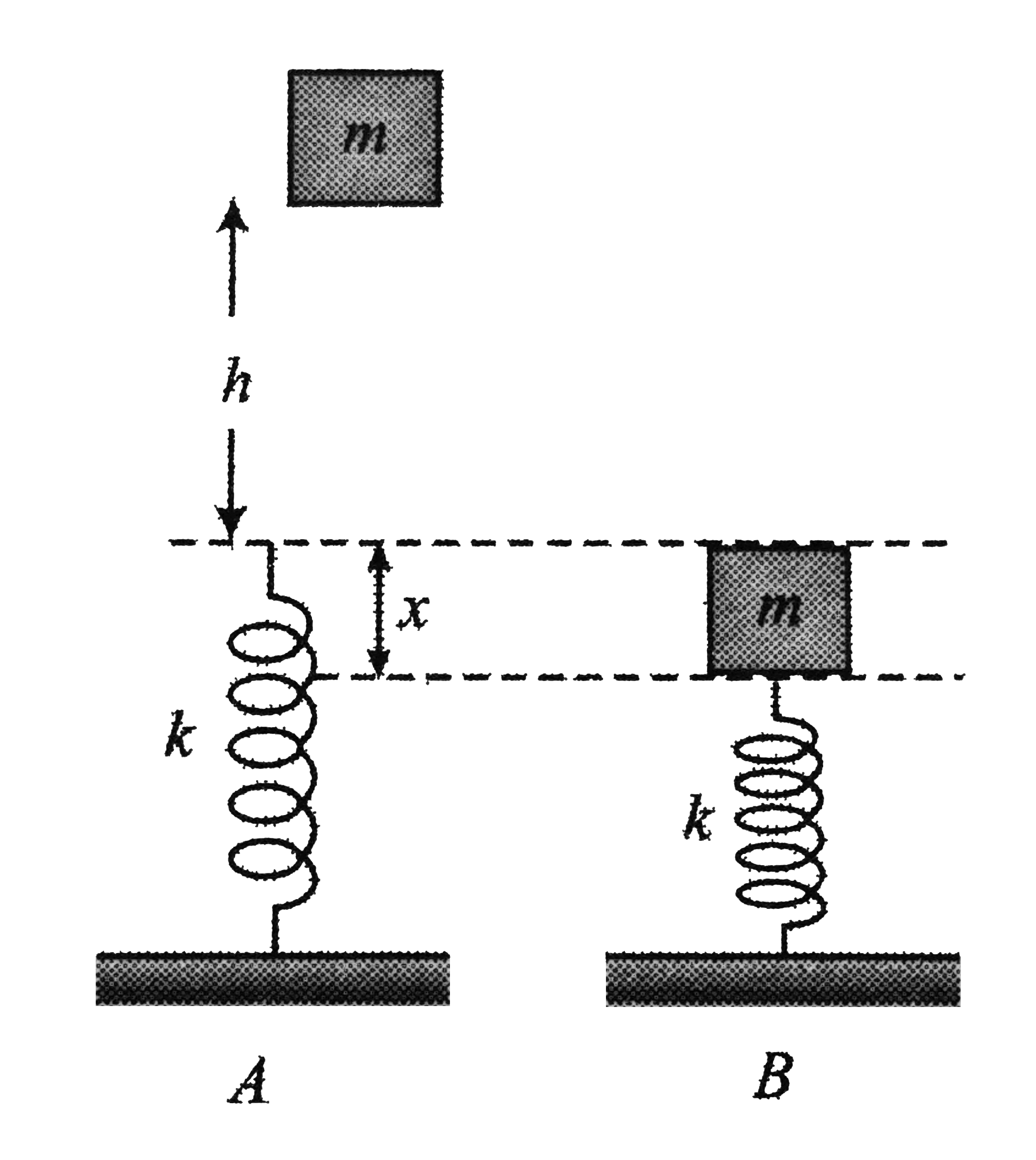
View SolutionA block of mass m is released from rest onto a spring. A having stiffness kA=mg/2h as shown in figure. If the block compresses spring B through a distance h, find the:
a. stiffness of the spring B
b. equilibrium position of the block
c. maximum velocity of the block
d. maximum acceleration of the block
View SolutionA block of mass m is released from rest onto a spring. A having stiffness kA=mg/2h as shown in figure. If the block compresses spring B through a distance h, find the:

a. stiffness of the spring B
b. equilibrium position of the block
c. maximum velocity of the block
d. maximum acceleration of the block
View Solution
